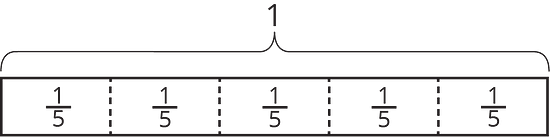
Lesson 4How Many Groups? (Part 1)
Let’s play with blocks and diagrams to think about division with fractions.
Learning Targets:
- I can find how many groups there are when the amount in each group is not a whole number.
- I can use diagrams and multiplication and division equations to represent “how many groups?” questions.
4.1 Equal-sized Groups
Write a multiplication equation and a division equation for each statement or diagram.
- Eight $5 bills are worth $40.
- There are 9 thirds in 3 ones.
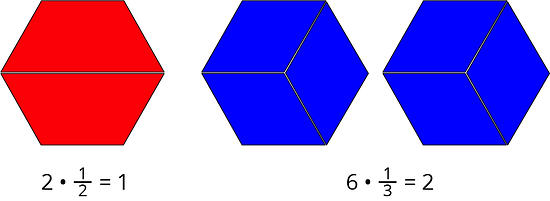
4.2 Reasoning with Pattern Blocks
Use the pattern blocks in the applet to answer the questions. (If you need help aligning the pieces, you can turn on the grid.)
- If a hexagon represents 1 whole, what fraction do each of the following shapes represent? Be prepared to show or explain your reasoning.
- 1 triangle
- 1 rhombus
- 1 trapezoid
- 4 triangles
- 3 rhombuses
- 2 hexagons
- 1 hexagon and 1 trapezoid
-
Here are Elena’s diagrams for and . Do you think these diagrams represent the equations? Explain or show your reasoning.
- Use pattern blocks to represent each multiplication equation. Recall that a hexagon represents 1 whole.
-
- Answer the following questions. If you get stuck, use pattern blocks.
-
How many s are in 4?
-
How many s are in 2?
-
How many s are in ?
-
Lesson 4 Summary
Some problems that involve equal-sized groups also involve fractions. Here is an example: “How many s are in 2?” We can express this question with multiplication and division equations.
Pattern-block diagrams can help us make sense of such problems. Here is a set of pattern blocks.

If the hexagon represents 1 whole, then a triangle must represent , because 6 triangles make 1 hexagon. We can use the triangle to represent the in the problem.

Twelve triangles make 2 hexagons, which means there are 12 groups of in 2.
If we write the 12 in the place of the “?” in the original equations, we have:
Lesson 4 Practice Problems
A shopper buys cat food in bags of 3 lbs. Her cat eats lb each week. How many weeks does one bag last?
-
Draw a diagram to represent the situation and label your diagram so it can be followed by others. Answer the question.
-
Write a multiplication or division equation to represent the situation.
-
Multiply your answer in the first question (the number of weeks) by . Did you get 3 as a result? If not, revise your previous work.
-
Use the diagram to answer the question: How many s are in ? The hexagon represents 1 whole. Explain or show your reasoning.
Which question can be represented by the equation ?
- How many 3s are in ?
- What is 3 groups of ?
- How many s are in 3?
- What is of 3?
Write two division equations for each multiplication equation.
Noah and his friends are going to an amusement park. The total cost of admission for 8 students is $100, and all students share the cost equally. Noah brought $13 for his ticket. Did he bring enough money to get into the park? Explain your reasoning.
Write a division expression with a quotient that is:
- greater than
- less than
- between and
Find each unknown number.
- 12 is 150% of what number?
- 5 is 50% of what number?
- 10% of what number is 300?
- 5% of what number is 72?
- 20 is 80% of what number?