Lesson 14Percent Error
Let’s use percentages to describe other situations that involve error.
Learning Targets:
- I can solve problems that involve percent error.
14.1 Number Talk: Estimating a Percentage of a Number
Estimate.
25% of 15.8
9% of 38
1.2% of 127
0.53% of 6
0.06% of 202
14.2 Plants, Bicycles, and Crowds
- Instructions to care for a plant say to water it with cup of water every day. The plant has been getting 25% too much water. How much water has the plant been getting?
- The pressure on a bicycle tire is 63 psi. This is 5% higher than what the manual says is the correct pressure. What is the correct pressure?
- The crowd at a sporting event is estimated to be 2,500 people. The exact attendance is 2,486 people. What is the percent error?
Are you ready for more?
A micrometer is an instrument that can measure lengths to the nearest micron (a micron is a millionth of a meter). Would this instrument be useful for measuring any of the following things? If so, what would the largest percent error be?
-
The thickness of an eyelash, which is typically about 0.1 millimeters.
-
The diameter of a red blood cell, which is typically about 8 microns.
-
The diameter of a hydrogen atom, which is about 100 picometers (a picometer is a trillionth of a meter).
14.3 Measuring in the Heat
A metal measuring tape expands when the temperature goes above . For every degree Fahrenheit above 50, its length increases by 0.00064%.
-
The temperature is 100 degrees Fahrenheit. How much longer is a 30-foot measuring tape than its correct length?
-
What is the percent error?
Lesson 14 Summary
Percent error can be used to describe any situation where there is a correct value and an incorrect value, and we want to describe the relative difference between them. For example, if a milk carton is supposed to contain 16 fluid ounces and it only contains 15 fluid ounces:
- the measurement error is 1 oz, and
- the percent error is 6.25% because .
We can also use percent error when talking about estimates. For example, a teacher estimates there are about 600 students at their school. If there are actually 625 students, then the percent error for this estimate was 4%, because and .
Glossary Terms
Percent error is a way to describe error, expressed as a percentage of the actual amount.
For example, a box is supposed to have 150 folders in it. Clare counts only 147 folders in the box. This is an error of 3 folders. The percent error is 2%, because 3 is 2% of 150. .
Lesson 14 Practice Problems
A student estimated that it would take 3 hours to write a book report, but it actually took her 5 hours. What is the percent error for her estimate?
A radar gun measured the speed of a baseball at 103 miles per hour. If the baseball was actually going 102.8 miles per hour, what was the percent error in this measurement?
It took 48 minutes to drive downtown. An app estimated it would be less than that. If the error was 20%, what was the app’s estimate?
- A farmer estimated that there were 25 gallons of water left in a tank. If this is an underestimate by 16%, how much water was actually in the tank?
For each story, write an equation that describes the relationship between the two quantities.
- Diego collected kg of recycling. Lin collected more than that.
- Lin biked km. Diego biked less than that.
- Diego read for minutes. Lin read of that.
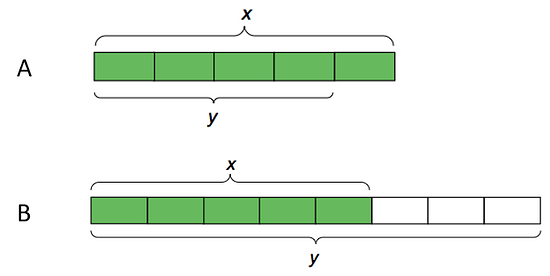
For each diagram, decide if is an increase or a decrease of . Then determine the percentage.
Lin is making a window covering for a window that has the shape of a half circle on top of a square of side length 3 feet. How much fabric does she need?