Lesson 9Solving Rate Problems
Let's use unit rates like a pro.
Learning Targets:
- I can choose how to use unit rates to solve problems.
9.1 Grid of 100
How much is shaded in each one?

9.2 Card Sort: Is it a Deal?
Your teacher will give you a set of cards showing different offers.
- Find card A and work with your partner to decide whether the offer on card A is a good deal. Explain or show your reasoning.
-
Next, split cards B–E so you and your partner each have two.
- Decide individually if your two cards are good deals. Explain your reasoning.
- For each of your cards, explain to your partner if you think it is a good deal and why. Listen to your partner’s explanations for their cards. If you disagree, explain your thinking.
- Revise any decisions about your cards based on the feedback from your partner.
- When you and your partner are in agreement about cards B–E, place all the cards you think are a good deal in one stack and all the cards you think are a bad deal in another stack. Be prepared to explain your reasoning.
Are you ready for more?
Time to make your own deal! Read the information on card F and then decide what you would charge if you were the clerk. When your teacher signals, trade cards with another group and decide whether or not you would take the other group’s offer.
Keep in mind that you may offer a fair deal or an unfair deal, but the goal is to set a price close enough to the value it should be so that the group cannot immediately tell if the deal you offer is a good one.
9.3 The Fastest of All
Wild animals from around the world wanted to hold an athletic competition, but no one would let them on an airplane. They decided to just measure how far each animal could sprint in one minute and send the results to you to decide the winner.
| animal | sprint distance |
|---|---|
| cougar | 1,408 yards |
| antelope | 1 mile |
| hare | 49,632 inches |
| kangaroo | 1,073 meters |
| ostrich | 1.15 kilometers |
| coyote | 3,773 feet |
You look up the following information about converting units of length:
1 inch = 2.54 centimeters
- Which animal sprinted the farthest?
-
What are the place rankings for all of the animals?
Lesson 9 Summary
Sometimes we can find and use more than one unit rate to solve a problem.
Suppose a grocery store is having a sale on shredded cheese. A small bag that holds 8 ounces is sold for $2. A large bag that holds 2 kilograms is sold for $16. How do you know which is a better deal?
Here are two different ways to solve this problem:
Compare dollars per kilogram.
- The large bag costs $8 per kilogram, because .
-
The small bag holds pound of cheese, because there are 16 ounces in 1 pound, and .
The small bag costs $4 per pound, because . This is about $8.80 per kilogram, because there are about 2.2 pounds in 1 kilogram, and .
The large bag is a better deal, because it costs less money for the same amount of cheese.
Compare ounces per dollar.
- With the small bag, we get 4 ounces per dollar, because .
-
The large bag holds 2,000 grams of cheese. There are 1,000 grams in 1 kilogram, and . This means 125 grams per dollar, because .
There are about 28.35 grams in 1 ounce, and , so this is about 4.4 ounces per dollar.
The large bag is a better deal, because you get more cheese for the same amount of money.
Another way to solve the problem would be to compare the unit prices of each bag in dollars per ounce. Try it!
Lesson 9 Practice Problems
This package of sliced cheese costs $2.97.
How much would a package with 18 slices cost at the same price per slice? Explain or show your reasoning.
A copy machine can print 480 copies every 4 minutes. For each question, explain or show your reasoning.
- How many copies can it print in 10 minutes?
- A teacher printed 720 copies. How long did it take to print?
Order these objects from heaviest to lightest. (Note: 1 pound = 16 ounces, 1 kilogram 2.2 pounds, and 1 ton = 2,000 pounds)
item weight school bus 9 tons horse 1,100 pounds elephant 5,500 kilograms grand piano 15,840 ounces Andre sometimes mows lawns on the weekend to make extra money. Two weeks ago, he mowed a neighbor’s lawn for hour and earned $10. Last week, he mowed his uncle’s lawn for hours and earned $30. This week, he mowed the lawn of a community center for 2 hours and earned $30.
Which jobs paid better than others? Explain your reasoning.
Calculate and express your answer in decimal form.
- Decompose this polygon so that its area can be calculated. All measurements are in centimeters.
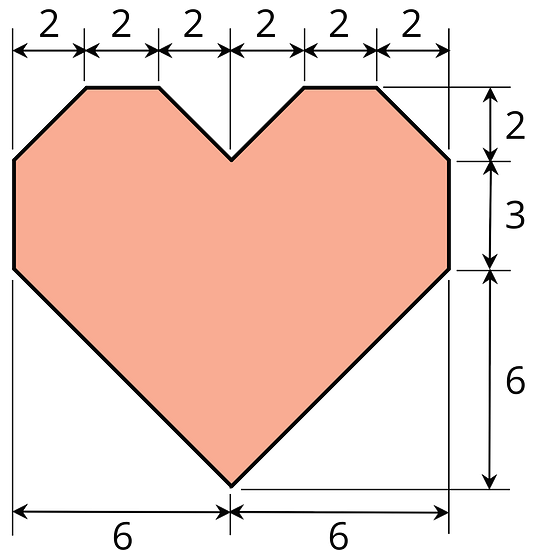
- Calculate its area. Organize your work so that it can be followed by others.