Open Up Resources Logo

Lesson 1Tape Diagrams and Equations

Let's see how tape diagrams and equations can show relationships between amounts.

Learning Targets:

  • I can tell whether or not an equation could represent a tape diagram.
  • I can use a tape diagram to represent a situation.

1.1 Which Diagram is Which?

Here are two diagrams. One represents 2+5=7 . The other represents 5 \boldcdot 2=10 . Which is which? Label the length of each diagram.

two tape diagrams are shown
Draw a diagram that represents each equation.
  1. 4+3=7
  1. 4 \boldcdot 3=12

1.2 Match Equations and Tape Diagrams

Here are two tape diagrams. Match each equation to one of the tape diagrams.

two tape diagrams are shown
  1. 4 + x = 12
  2. 12 \div 4 = x
  3. 4 \boldcdot x = 12
  1. 12 = 4 + x
  2. 12 - x = 4
  3. 12 = 4 \boldcdot x
  1. 12 - 4 = x
  2. x = 12 - 4
  3. x+x+x+x=12

1.3 Draw Diagrams for Equations

For each equation, draw a diagram and find the value of the unknown that makes the equation true.

  1. 18 = 3+x  
  2. 18 = 3 \boldcdot y

Are you ready for more?

You are walking down a road, seeking treasure. The road branches off into three paths. A guard stands in each path. You know that only one of the guards is telling the truth, and the other two are lying. Here is what they say:

  • Guard 1: The treasure lies down this path.
  • Guard 2: No treasure lies down this path; seek elsewhere.
  • Guard 3: The first guard is lying.
Which path leads to the treasure?

Lesson 1 Summary

Tape diagrams can help us understand relationships between quantities and how operations describe those relationships. 

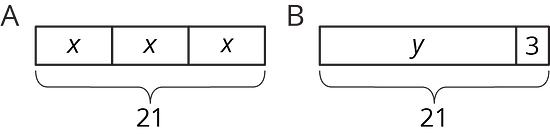
two tape diagrams are shown

Diagram A has 3 parts that add to 21. Each part is labeled with the same letter, so we know the three parts are equal. Here are some equations that all represent diagram A:

x+x+x=21 3\boldcdot {x}=21 x=21\div3 x=\frac13\boldcdot {21}

Notice that the number 3 is not seen in the diagram; the 3 comes from counting 3 boxes representing 3 equal parts in 21.

We can use the diagram or any of the equations to reason that the value of x is 7.

Diagram B has 2 parts that add to 21. Here are some equations that all represent diagram B:

y+3=21 y=21-3 3=21-y

We can use the diagram or any of the equations to reason that the value of  y  is 18.

Lesson 1 Practice Problems

  1. Here is an equation: x + 4 = 17

    1. Draw a tape diagram to represent the equation.
    1. Which part of the diagram shows the quantity x ? What about 4? What about 17?
    1. How does the diagram show that x+4 has the same value as 17?

  2. Diego is trying to find the value of x in 5 \boldcdot x = 35 . He draws this diagram but is not certain how to proceed.

    tape diagram of 5 x's
    1. Complete the tape diagram so it represents the equation 5 \boldcdot x = 35 .
    2. Find the value of x .

  3. For each equation, draw a tape diagram and find the unknown value.

    1. x+9=16
    1. 4 \boldcdot x = 28

  4. Match each equation to one of the two tape diagrams.

    1. x + 3 = 9
    2. 3 \boldcdot x = 9
    3. 9=3 \boldcdot x
    4. 3+x=9
    5. x = 9 - 3
    6. x = 9 \div 3
    7. x + x+ x = 9
    two tape diagrams are shown

  5. A shopper paid $2.52 for 4.5 pounds of potatoes, $7.75 for 2.5 pounds of broccoli, and $2.45 for 2.5 pounds of pears. What is the unit price of each item she bought? Show your reasoning.

  6. A sports drink bottle contains 16.9 fluid ounces. Andre drank 80% of the bottle. How many fluid ounces did Andre drink? Show your reasoning.

  7. The daily recommended allowance of calcium for a sixth grader is 1,200 mg. One cup of milk has 25% of the recommended daily allowance of calcium. How many milligrams of calcium are in a cup of milk? If you get stuck, consider using the double number line.

    A double number line with 2 tick marks at either end of the line. The top number line is labeled “calcium in milligrams” and the tick marks are labeled 0 and 1200. The bottom number line is not labeled and the tick marks are labeled 0 and 100 percent.