Lesson 3More about Constant of Proportionality
Let’s solve more problems involving proportional relationships using tables.
Learning Targets:
- I can find missing information in a proportional relationship using a table.
- I can find the constant of proportionality from information given in a table.
3.1 Equal Measures
Use the numbers and units from the list to find as many equivalent measurements as you can. For example, you might write “30 minutes is hour.”
You can use the numbers and units more than once.
1
12
0.4
60
50
40
0.01
30
0.3
24
6
2
centimeter
meter
hour
feet
minute
inch
3.2 Centimeters and Millimeters
There is a proportional relationship between any length measured in centimeters and the same length measured in millimeters.
There are two ways of thinking about this proportional relationship.
-
If you know the length of something in centimeters, you can calculate its length in millimeters.
- Complete the table.
- What is the constant of proportionality?
| length (cm) | length (mm) |
|---|---|
| 9 | |
| 12.5 | |
| 50 | |
| 88.49 |
-
If you know the length of something in millimeters, you can calculate its length in centimeters.
- Complete the table.
- What is the constant of proportionality?
| length (mm) | length (cm) |
|---|---|
| 70 | |
| 245 | |
| 4 | |
| 699.1 |
- How are these two constants of proportionality related to each other?
-
Complete each sentence:
- To convert from centimeters to millimeters, you can multiply by ________.
- To convert from millimeters to centimeters, you can divide by ________ or multiply by ________.
Are you ready for more?
- How many square millimeters are there in a square centimeter?
- How do you convert square centimeters to square millimeters? How do you convert the other way?
3.3 Pittsburgh to Phoenix
A plane traveling at a constant speed flew over Pittsburgh, Saint Louis, Albuquerque, and Phoenix on its way from New York to San Diego.
Complete the table as you answer the questions. Be prepared to explain your reasoning.
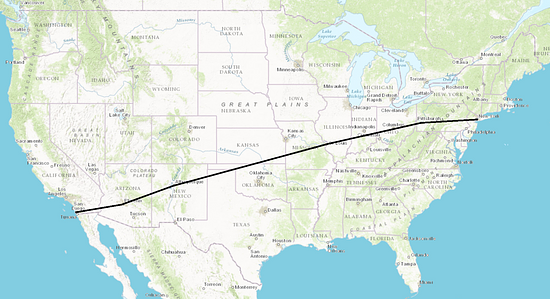
| segment | time | distance | speed |
|---|---|---|---|
| Pittsburgh to Saint Louis | 1 hour | 550 miles | |
| Saint Louis to Albuquerque | 1 hour 42 minutes | ||
| Albuquerque to Phoenix | 330 miles |
-
What is the distance between Saint Louis and Albuquerque?
-
How many minutes did it take to fly between Albuquerque and Phoenix?
- What is the proportional relationship represented by this table?
- Diego says the constant of proportionality is 550. Andre says the constant of proportionality is . Do you agree with either of them? Explain your reasoning.
Lesson 3 Summary
When something is traveling at a constant speed, there is a proportional relationship between the time it takes and the distance traveled. The table shows the distance traveled and elapsed time for a bug crawling on a sidewalk.
We can multiply any number in the first column by to get the corresponding number in the second column. We can say that the elapsed time is proportional to the distance traveled, and the constant of proportionality is . This means that the bug’s pace is seconds per centimeter.
This table represents the same situation, except the columns are switched.
We can multiply any number in the first column by to get the corresponding number in the second column. We can say that the distance traveled is proportional to the elapsed time, and the constant of proportionality is . This means that the bug’s speed is centimeters per second.
Notice that is the reciprocal of . When two quantities are in a proportional relationship, there are two constants of proportionality, and they are always reciprocals of each other. When we represent a proportional relationship with a table, we say the quantity in the second column is proportional to the quantity in the first column, and the corresponding constant of proportionality is the number we multiply values in the first column to get the values in the second.
Lesson 3 Practice Problems
Noah is running a portion of a marathon at a constant speed of 6 miles per hour.
Complete the table to predict how long it would take him to run different distances at that speed, and how far he would run in different time intervals.
time
in hoursmiles traveled at
6 miles per hour1 9 One kilometer is 1000 meters.
- Complete the tables. What is the interpretation of the constant of proportionality in each case?
meters kilometers 1,000 1 250 12 1 kilometers meters 1 1,000 5 20 0.3 The constant of proportionality tells us that:
The constant of proportionality tells us that:
- What is the relationship between the two constants of proportionality?
- Complete the tables. What is the interpretation of the constant of proportionality in each case?
Jada and Lin are comparing inches and feet. Jada says that the constant of proportionality is 12. Lin says it is . Do you agree with either of them? Explain your reasoning.
The area of the Mojave desert is 25,000 square miles. A scale drawing of the Mojave desert has an area of 10 square inches. What is the scale of the map?
Which of these scales is equivalent to the scale 1 cm to 5 km? Select all that apply.
-
3 cm to 15 km
-
1 mm to 150 km
-
5 cm to 1 km
-
5 mm to 2.5 km
-
1 mm to 500 m
-
Which one of these pictures is not like the others? Explain what makes it different using ratios.