Lesson 12What Is Surface Area?
Learning Goal
Let’s cover the surfaces of some three-dimensional objects.
Learning Targets
I know what the surface area of a three-dimensional object means.
Lesson Terms
- face
- surface area
Warm Up: Covering the Cabinet (Part 1)
Problem 1
Your teacher will show you a video about a cabinet or some pictures of it.
Estimate an answer to the question: How many sticky notes would it take to cover the cabinet, excluding the bottom?
Activity 1: Covering the Cabinet (Part 2)
Problem 1
Earlier, you learned about a cabinet being covered with sticky notes.
How could you find the actual number of sticky notes it will take to cover the cabinet, excluding the bottom? What information would you need to know?
Use the information you have to find the number of sticky notes needed to cover the cabinet. Show your reasoning.
Are you ready for more?
Problem 1
How many sticky notes are needed to cover the outside of 2 cabinets pushed together (including the bottom)? What about 3 cabinets? 20 cabinets?
Activity 2: Building with Snap Cubes
Problem 1
Here is a rectangular prism built from 12 cubes:
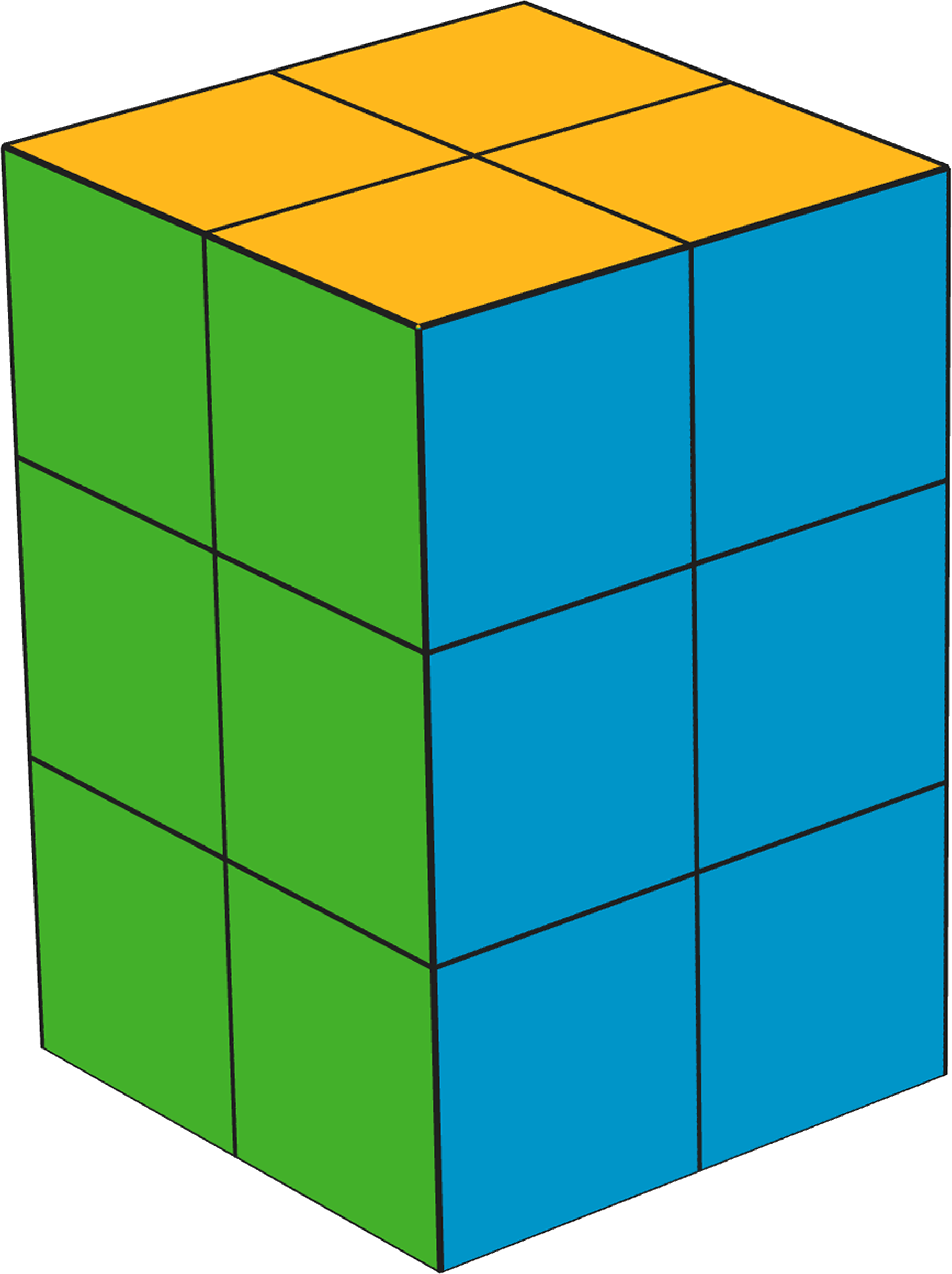
It has six faces, but you can only see three of them in the sketch. It has a surface area of 32 square units.
The applet has 12 blocks, too. They are all in the same spot on the screen, like a hidden stack of blocks. You will always know where the stack is because it sits on a gray square. To use a block, drag the red point to move it. Click on the red points to change from left/right movement to up/down movement.
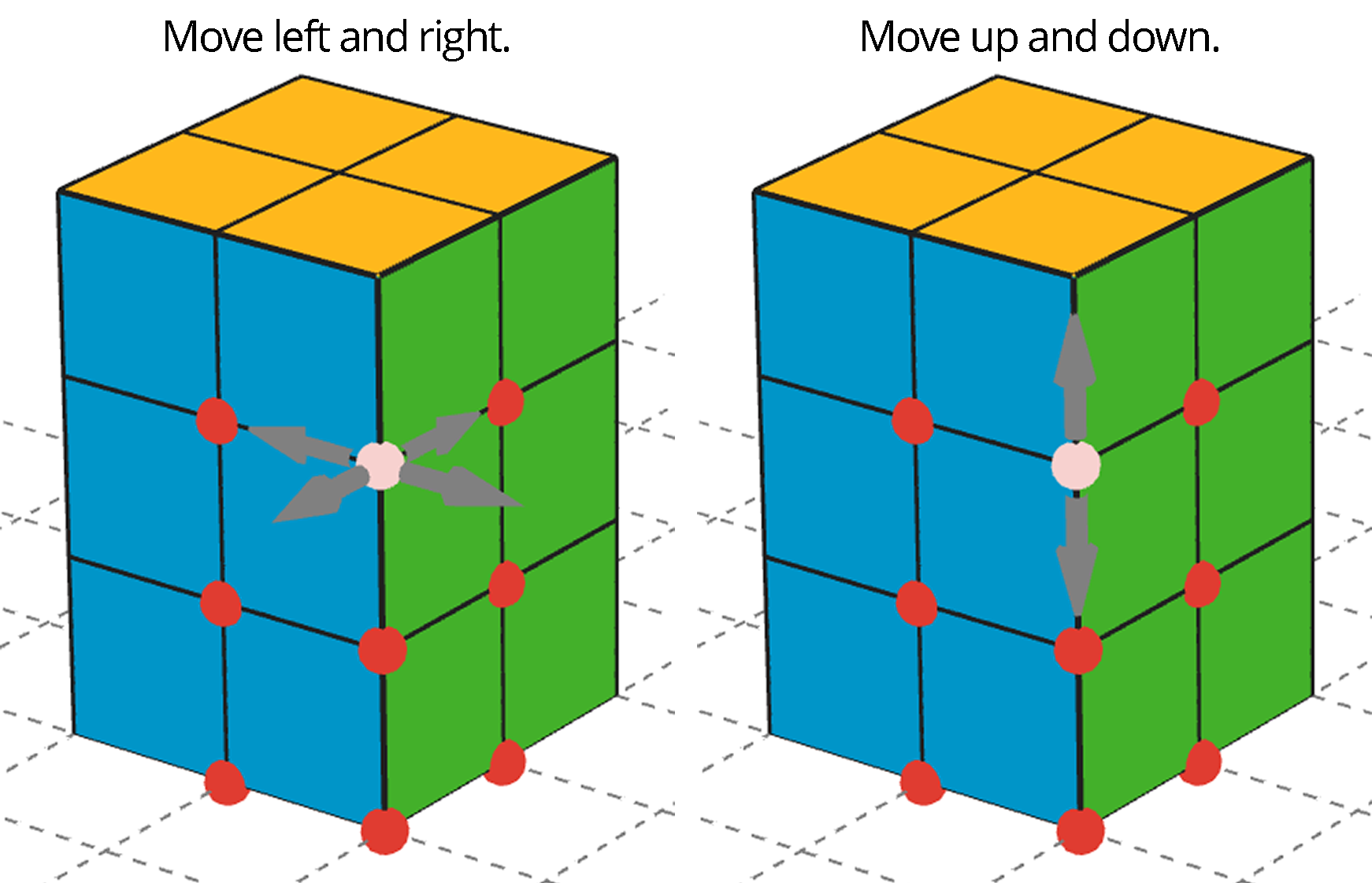
Use all 12 cubes to build a different rectangular prism (with different edge lengths than shown in the prism here). You can turn the view to see all of the faces of your figure.
How many faces does your figure have?
What is the surface area of your figure in square units?
Draw your figure on isometric dot paper. Color each face a different color.

Print Version
Here is a sketch of a rectangular prism built from 12 cubes:
It has six faces, but you can only see three of them in the sketch. It has a surface area of 32 square units.
You have 12 snap cubes from your teacher. Use all of your snap cubes to build a different rectangular prism (with different edge lengths than shown in the prism here).
How many faces does your figure have?
What is the surface area of your figure in square units?
Draw your figure on isometric dot paper. Color each face a different color.

Lesson Summary
The surface area of a figure (in square units) is the number of unit squares it takes to cover the entire surface without gaps or overlaps.
If a three-dimensional figure has flat sides, the sides are called faces.
The surface area is the total of the areas of the faces.
For example, a rectangular prism has six faces. The surface area of the prism is the total of the areas of the six rectangular faces.
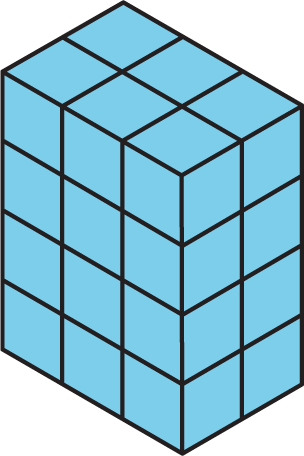
So the surface area of a rectangular prism that has edge-lengths 2 cm, 3 cm, and 4 cm has a surface area of