Lesson 8Calculating Products of Decimals
Let’s multiply decimals.
Learning Targets:
- I can describe and apply a method for multiplying decimals.
- I know how to use a product of whole numbers to find a product of decimals.
8.1 Number Talk: Twenty Times a Number
Evaluate mentally.
8.2 Calculating Products of Decimals
-
A common way to find a product of decimals is to calculate a product of whole numbers, then place the decimal point in the product.
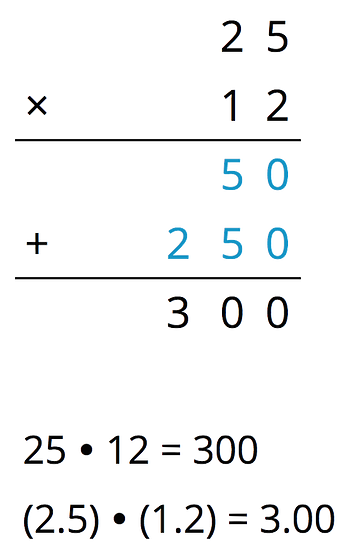
Here is an example for .
Use what you know about decimals and place value to explain why the decimal point of the product is placed where it is.
-
Use the method shown in the first question to calculate each product.
-
Use area diagrams to check your earlier calculations. For each problem:
- Decompose each number into its base-ten units and write them in the boxes on each side of the rectangle.
- Write the area of each lettered region in the diagram. Then find the area of the entire rectangle. Show your reasoning.
-
About how many centimeters are in 6.25 inches if 1 inch is about 2.5 centimeters? Show your reasoning.
8.3 Practicing Multiplication of Decimals
-
Calculate each product. Show your reasoning. If you get stuck, draw an area diagram to help.
-
A rectangular playground is 18.2 meters by 12.75 meters.
- Find its area in square meters. Show your reasoning.
- If 1 meter is approximately 3.28 feet, what are the approximate side lengths of the playground in feet? Show your reasoning.
Are you ready for more?
-
Write the following expressions as decimals.
- Describe the decimal that results as this process continues.
- What would happen to the decimal if all of the positive and negative signs became multiplication symbols? Explain your reasoning.
Lesson 8 Summary
We can use and what we know about place value to find .
Since 8.4 is 84 tenths and 4.3 is 43 tenths, then:
That means we can compute and then divide by 100 to find .
Using fractions such as , , and allows us to find the product of two decimals using the following steps:
- Write each decimal factor as a product of a whole number and a fraction.
- Multiply the whole numbers.
- Multiply the fractions.
- Multiply the products of the whole numbers and fractions.
We know multiplying by fractions such as , , and is the same as dividing by 10, 100, and 1,000, respectively. This means we can move the decimal point in the whole-number product to the left the appropriate number of spaces to correctly place the decimal point.
Lesson 8 Practice Problems
- Here are an unfinished calculation of and a 0.54-by-3.8 rectangle.
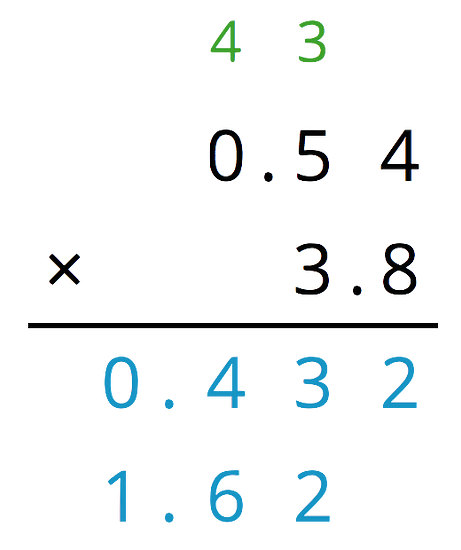

- Which part of the rectangle has an area of 0.432? Which part of the rectangle has an area of 1.62? Show your reasoning.
- What is ?
Explain how the product of 3 and 65 could be used to find .
- Use vertical calculation to find each product.
- A pound of blueberries costs $3.98 and a pound of clementines costs $2.49. What is the combined cost of 0.6 pound of blueberries and 1.8 pounds of clementines? Round your answer to the nearest cent.
Complete the calculations so that each shows the correct sum or difference.
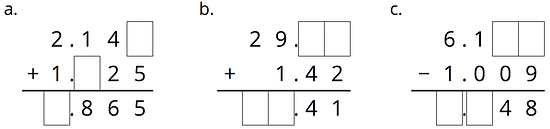
Which has a greater value: or ? Show your reasoning.
Andre is planting saplings (baby trees). It takes him 30 minutes to plant 3 saplings. If each sapling takes the same amount of time to plant, how long will it take Andre to plant 14 saplings? If you get stuck, consider using the table.
number of saplings time in minutes 3 30 1 14