Open Up Resources Logo

Lesson 2Exploring Circles

Let’s explore circles.

Learning Targets:

  • I can describe the characteristics that make a shape a circle.
  • I can identify the diameter, center, radius, and circumference of a circle.

2.1 How Do You Figure?

Here are two figures.

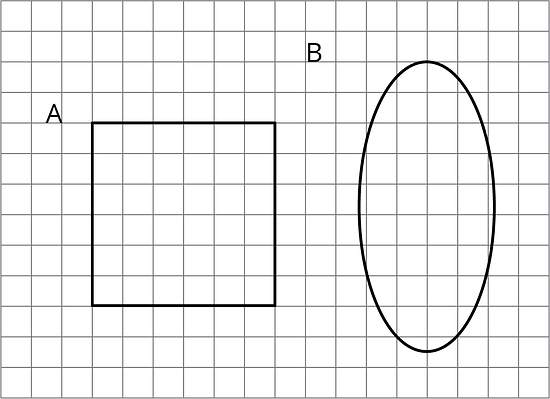
Two figures on a grid labeled A and B. Figure A is a square with horizontal distance 6 units. Figure B is a curved figure with horizontal distance of approximately 4 units and vertical distance of approximately 9.5 units.

Figure C looks more like Figure A than like Figure B. Sketch what Figure C might look like. Explain your reasoning.

2.2 Sorting Round Objects

Your teacher will give you some pictures of different objects.

  1. How could you sort these pictures into two groups? Be prepared to share your reasoning.
  2. Work with your partner to sort the pictures into the categories that your class has agreed on. Pause here so your teacher can review your work.
  3. What are some characteristics that all circles have in common?
  4. Put the circular objects in order from smallest to largest.
  5. Select one of the pictures of a circular object. What are some ways you could measure the actual size of your circle?

Are you ready for more?

On January 3rd, the Earth is 147,500,000 kilometers away from the Sun. On July 4th, the Earth is 152,500,000 kilometers away from the Sun. The sun has a radius of about 865,000 kilometers.

Could the Earth’s orbit be a circle with some point in the Sun as its center? Explain your reasoning.

A circle with a diameter and points at January 3 and July 4

2.3 Measuring Circles

Priya, Han, and Mai each measured one of the circular objects from earlier.

  • Priya says that the bike wheel is 24 inches.
  • Han says that the yo-yo trick is 24 inches.
  • Mai says that the glow necklace is 24 inches.
  1. Do you think that all these circles are the same size?
  2. What part of the circle did each person measure? Explain your reasoning.

2.4 Drawing Circles

Spend some time familiarizing yourself with the tools that are available in this applet.

open applet in presentation mode

Draw and label each circle. 

  • Circle A, with a diameter of 6 cm.
  • Circle B, with a radius of 5 cm.
  • Circle C, with a radius that is equal to Circle A’s diameter.
  • Circle D, with a diameter that is equal to Circle B’s radius. Pause here so your teacher can review your work.
  • Use a compass to recreate one of these designs.
Different images are formed by multiple circles

Lesson 2 Summary

A circle consists of all of the points that are the same distance away from a particular point called the center of the circle.

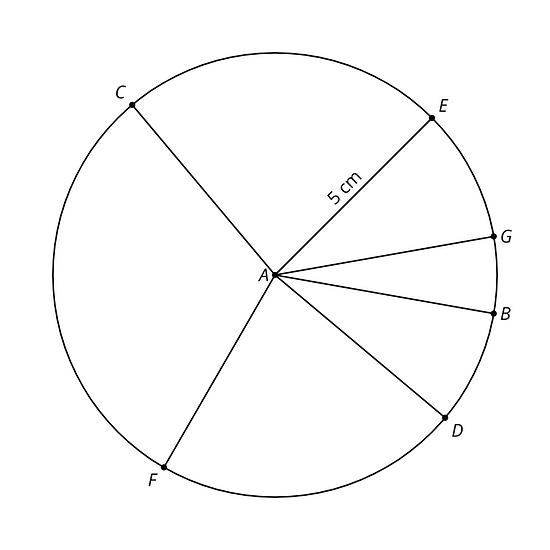
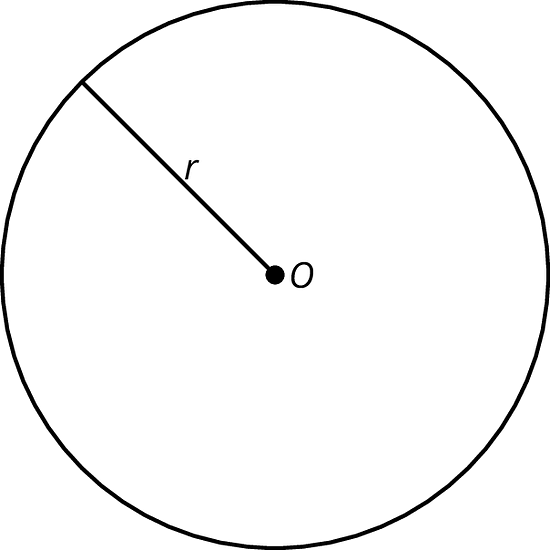
A segment that connects the center with any point on the circle is called a radius. For example, segments QG , QH , QI , and QJ are all radii of circle 2. (We say one radius and two radii.) The length of any radius is always the same for a given circle. For this reason, people also refer to this distance as the radius of the circle.

Two circles labeled circle 1 and circle 2. Circle 1 has a center at P and the points A, C, F, B, D, and E lie on the circle. Diameters A B, C D, and E F are drawn. Circle 2 has a center at Q and the points G, H, I and J lie on the circle. Line segments Q G, Q H, Q I, and Q J are drawn.

A segment that connects two opposite points on a circle (passing through the circle’s center) is called a diameter. For example, segments AB , CD , and EF are all diameters of circle 1. All diameters in a given circle have the same length because they are composed of two radii. For this reason, people also refer to the length of such a segment as the diameter of the circle.

The circumference of a circle is the distance around it. If a circle was made of a piece of string and we cut it and straightened it out, the circumference would be the length of that string. A circle always encloses a circular region. The region enclosed by circle 2 is shaded, but the region enclosed by circle 1 is not. When we refer to the area of a circle, we mean the area of the enclosed circular region.

Glossary Terms

circle

A circle is made out of all the points that are the same distance from a given point.

For example, every point on this circle is 5 cm away from point A , which is the center of the circle.

Cicrle A has 6 radii of 5 centimeters
circumference

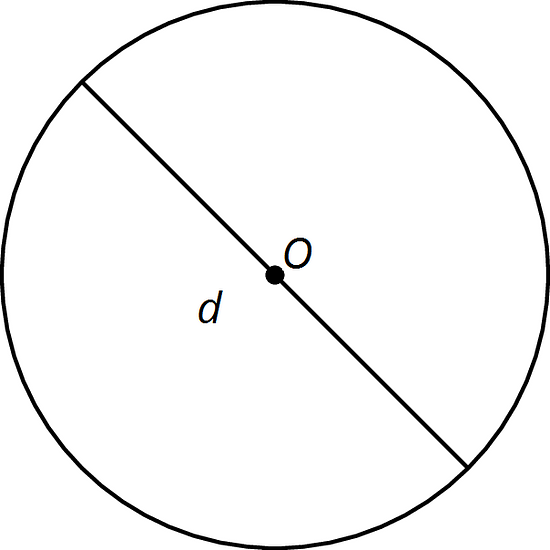
The circumference of a circle is the distance around the circle. If you imagine the circle as a piece of string, it is the length of the string. If the circle has radius r then the circumference is 2\pi r .

The circumference of a circle of radius 3 is 2\boldcdot \pi \boldcdot 3 = 6\pi , which is about 18.85. 

diameter

A diameter is a line segment that goes from one edge of a circle to the other and passes through the center. A diameter can go in any direction. Every diameter of the circle is the same length. We also use the word diameter to mean the length of this segment.

For example, d is the diameter of this circle with center O .

Circle O has a diameter of d
radius

A radius is a line segment that goes from the center to the edge of a circle. A radius can go in any direction. Every radius of the circle is the same length. We also use the word radius to mean the length of this segment.

For example, r is the radius of this circle with center O .  

Circle O has a radius of r

Lesson 2 Practice Problems

  1. Use a geometric tool to draw a circle. Draw and measure a radius and a diameter of the circle.

  2. Here is a circle with center H and some line segments and curves joining points on the circle.

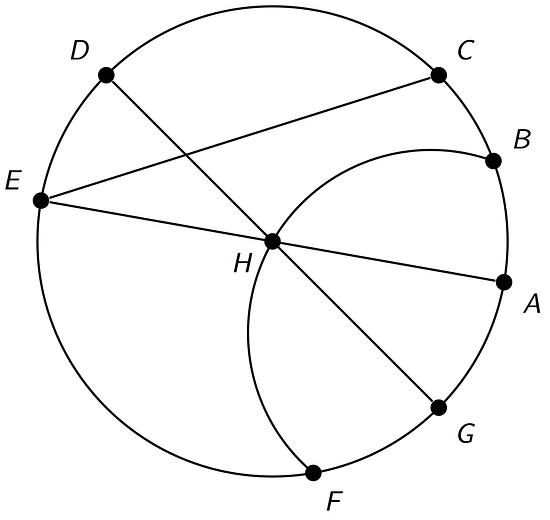
    A circle, with a center labeled H. Around the edge of the circle are points labeled A, B, C, D, E, F, and G. A line extends from point A through center H to point E. A curved extends from point B to center H. A line extends from point C to point E. A line extends from point D through center H to point G. A curved line extends from point F to center H.

    Identify examples of the following. Explain your reasoning.

    1. Diameter

    2. Radius

  3. Lin measured the diameter of a circle in two different directions. Measuring vertically, she got 3.5 cm, and measuring horizontally, she got 3.6 cm. Explain some possible reasons why these measurements differ.

  4. A small, test batch of lemonade used \frac{1}{4} cup of sugar added to 1 cup of water and \frac{1}{4} cup of lemon juice. After confirming it tasted good, a larger batch is going to be made with the same ratios using 10 cups of water. How much sugar should be added so that the large batch tastes the same as the test batch?

  5. The graph of a proportional relationship contains the point with coordinates (3, 12) . What is the constant of proportionality of the relationship?