Unit 7 Rational Numbers (Family Materials)

This week, your student will work with signed numbers, or positive and negative numbers. We often compare signed numbers when talking about temperatures. For example, -30 degrees Fahrenheit is colder than -10 degrees Fahrenheit. We say “-30 is less than -10” and write: .

We also use signed numbers when referring to elevation, or height relative to the sea level. An elevation of 2 feet (which means 2 feet above sea level) is higher than an elevation of -4 feet (which means 4 feet below sea level). We say “2 is greater than -4” and write .

We can plot positive and negative numbers on the number line. Numbers to the left are always less than numbers to the right.

We can see that -1.3 is less than 0.8 because -1.3 is to the left of 0.8, but -1.3 is greater than -2.7 because it is to the right of -2.7.

We can also talk about a number in terms of its absolute value, or its distance from zero on the number line. For example, 0.8 is 0.8 units away from zero, which we can write as , and -2.7 is 2.7 units away from zero, which we can write as . The numbers -3 and 3 are both 3 units from 0, which we can write as and .

Here is a task to try with your student:

1. A diver is at the surface of the ocean, getting ready to make a dive. What is the diver’s elevation in relation to sea level?

2. The diver descends 100 feet to the top of a wrecked ship. What is the diver’s elevation now?

3. The diver descends 25 feet more toward the ocean floor. What is the absolute value of the diver’s elevation now?

4. Plot each of the three elevations as a point on a number line. Label each point with its numeric value.

Solution:

1. 0, because sea level is 0 feet above or below sea level

2. -100, because the diver is 100 feet below sea level

3. The new elevation is -125 feet or 125 feet below sea level, so its absolute value is 125 feet.

4. A number line with 0, -100, and -125 marked, as shown:

   

This week your student will be adding and subtracting with negative numbers. We can represent this on a number line using arrows. The arrow for a positive number points right, and the arrow for a negative number points left. We add numbers by putting the arrows tail to tip.

For example, here is a number line that shows .

The first number is represented by an arrow that starts at 0 and points 5 units to the left. The next number is represented by an arrow that starts directly above the tip of the first arrow and points 12 units to the right. The answer is 7 because the tip of this arrow ends above the 7 on the number line.

In elementary school, students learned that every addition equation has two related subtraction equations. For example, if we know , then we also know and .

The same thing works when there are negative numbers in the equation. From the previous example, , we also know and .

Here is a task to try with your student:

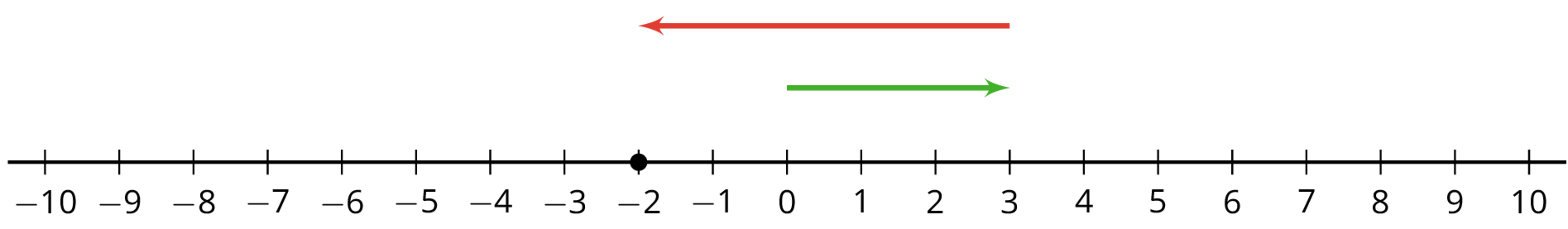
1. Use the number line to show .

   

2. What does your answer tell you about the value of:

   1. ?

   2. ?

Solution:

1. The first arrow starts at 0 and points 3 units to the right. The next arrow starts at the tip of the first arrow and points 5 units to the left. This arrow ends above the -2, so .

   

2. From the addition equation , we get the related subtraction equations:

This week, your students will plot and interpret points on the coordinate plane. In earlier grades, they plotted points where both coordinates are positive, such as point in the figure. They will now plot points that have positive and negative coordinates, such as points and .

To find the distance between two points that share the same horizontal line or the same vertical lines, we can simply count the grid units between them. For example, if we plot the point on the grid above (try it!), we can tell that the point will be 7 units away from point .

Points on a coordinate plane can also represent situations that involve positive and negative numbers. For instance, the points on this coordinate plane shows the temperature in degrees Celsius every hour before and after noon on a winter day. Times before noon are negative and times after noon are positive.

For example, the point tells us that 5 hours after noon, or 5:00 p.m, the temperature was 10 degrees Celsius.

Here is a task to try with your student:

In the graph of temperatures above:

1. What was the temperature at 7 a.m.?

2. For which recorded times was it colder than 5 degrees Celsius?

Solution:

1. It was -5 degrees Celsius at 7:00 a.m. You can see this at the point .

2. It was 5 degrees Celsius right at noon, and for the times recorded before that, it was colder.

This week your student will be multiplying and dividing with negative numbers. The rules for multiplying positive and negative numbers are designed to make sure that addition and multiplication work the same way they always have.

For example, in elementary school students learned to think of “4 times 3” as 4 groups of 3, like . We can think of “4 times -3” the same way: . Also, an important property of multiplication is that we can multiply numbers in either order. This means that .

What about ? It may seem strange, but the answer is 12. To understand why this is, we can think of -4 as .

After more practice, your student will be able to remember this without needing to think through examples:

• A positive times a negative is a negative.

• A negative times a positive is a negative.

• A negative times a negative is a positive.

Here is a task to try with your student:

1. Calculate .

2. Use your answer to the previous question to calculate:

Solution:

1. The answer is -10. We can think of as 5 groups of -2, so

2. 1. The answer is -10. We can multiply numbers in either order, so

   2. The answer is 10. We can think of -5 as , and .

   3. The answer is 10. Possible Strategies:

      • We can think of -2 as , and .

      • We can multiply numbers in either order, so .

This week your student will use what they know about negative numbers to solve equations.

• The opposite of 5 is -5, because . This is also called the additive inverse.

• The reciprocal of 5 is , because . This is also called the multiplicative inverse.

Thinking about opposites and reciprocals can help us solve equations. For example, what value of makes the equation true?

The solution is -15.

What value of makes the equation true?

The solution is -18.

Here is a task to try with your student:

Solve each equation:

Solution:

1. -8, because .

2. 7.5 or equivalent, because .

3. -16, because .