Open Up Resources Logo

Lesson 3Nonadjacent Angles

Let’s look at angles that are not right next to one another.

Learning Targets:

  • I can determine if angles that are not adjacent are complementary or supplementary.
  • I can explain what vertical angles are in my own words.

3.1 Finding Related Statements

Given a and b are numbers, and a+b=180 , which statements also must be true?

a=180-b

a-180=b

360=2a+2b

a=90 and b=90

3.2 Polygon Angles

Use any useful tools in the geometry toolkit to identify any pairs of angles in these figures that are complementary or supplementary. 

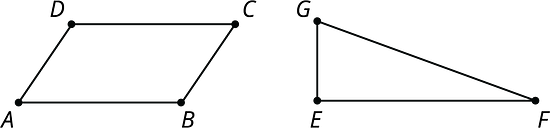
parallelogram ABCD and Traingle EFG are shown.

3.3 Vertical Angles

Use a straightedge to draw two intersecting lines. Use a protractor to measure all four angles whose vertex is located at the intersection.

Compare your drawing and measurements to the people in your group. Make a conjecture about the relationships between angle measures at an intersection.

3.4 Row Game: Angles

Find the measure of the angles in one column. Your partner will work on the other column. Check in with your partner after you finish each row. Your answers in each row should be the same. If your answers aren’t the same, work together to find the error and correct it.

column A column B

P is on line m . Find the value of a .

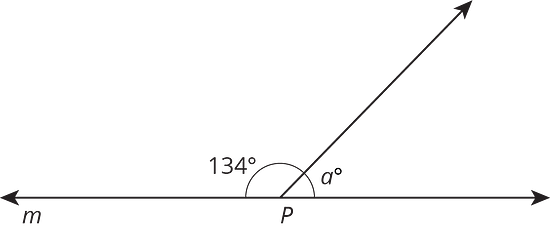A 134 degree angle and angle a form a straight angle.

Find the value of b .

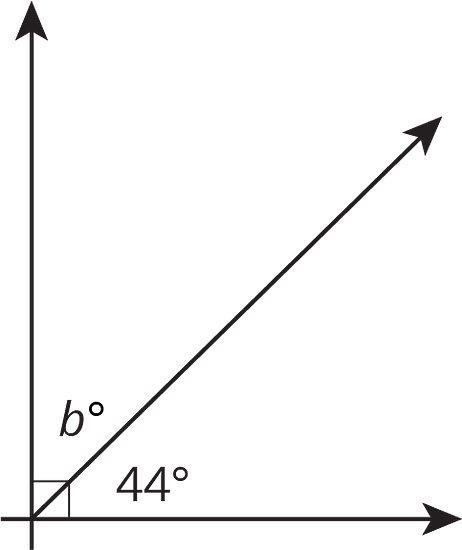A 44 degree angle and its angle next to it form a right angle.

Find the value of a .

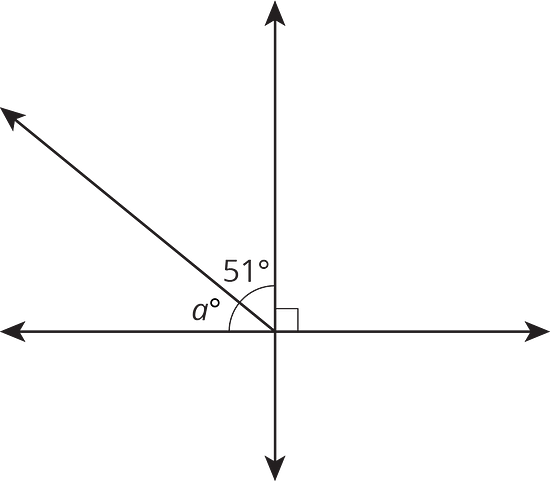Angle a and is next to an angle of 51 degrees. They form a 90 degree angle.

In right triangle LMN , angles L and M are complementary. Find the measure of angle 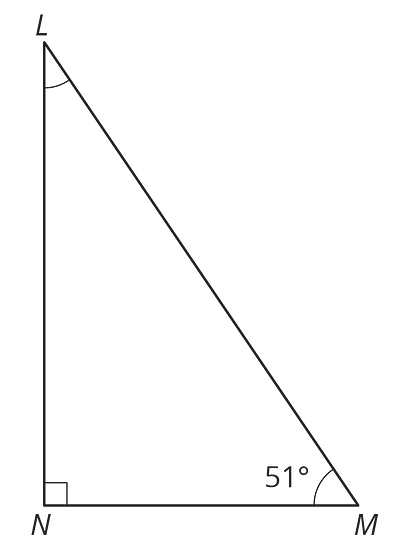L .

Right angle LMN has an angle of 51 degrees
column A column B

Angle C and angle E are supplementary. Find the measure of angle 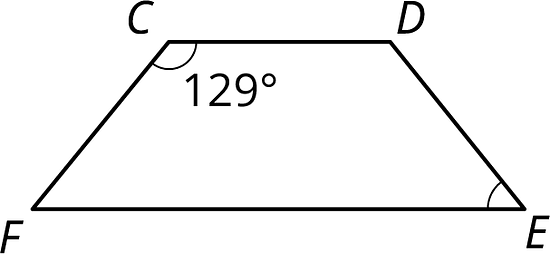E .

Trapezoid CDEF has an angle of 129 degrees

X is on line WY . Find the value of b .

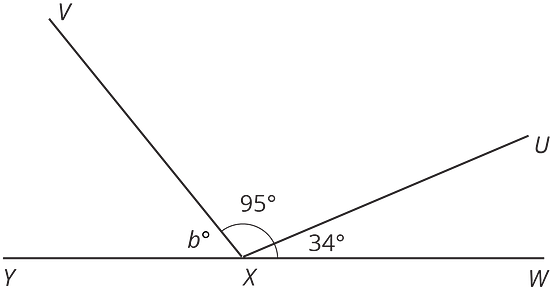Angles of 34, 95, and b degrees formed a straight angle

Find the value of c .

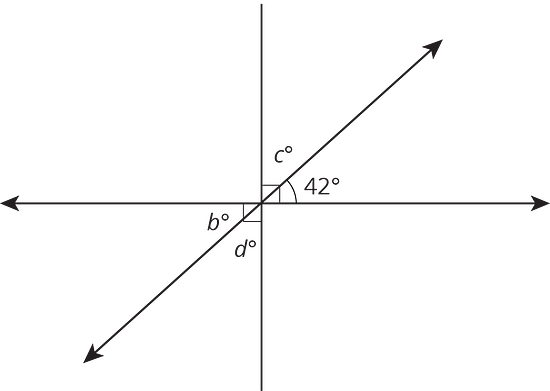B is on line FW . Find the measure of angle 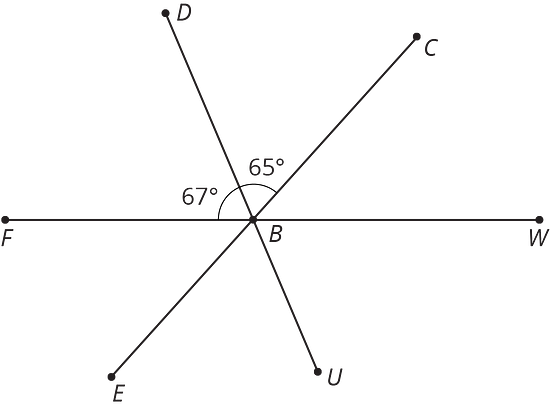CBW .

Angles are formed by 3 intersecting lines. The two labeled angles are 65 and 67 degrees

Two angles are complementary. One angle measures 37 degrees. Find the measure of the other angle.

Two angles are supplementary. One angle measures 127 degrees. Find the measure of the other angle.

Lesson 3 Summary

When two lines cross, they form two pairs of vertical angles. Vertical angles are across the intersection point from each other.

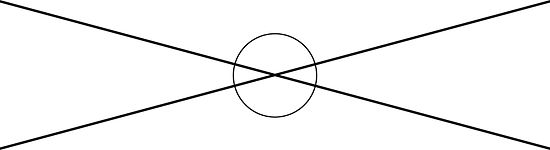
Two intersecting lines form 4 different angles

Vertical angles always have equal measure. We can see this because they are always supplementary with the same angle. For example:

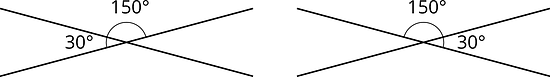
Straigh angles are formed by 30 and 150 degrees.

This is always true!

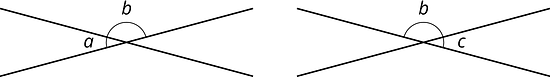
Angles A and B form a straight angle. Angles B and C also form a straight angle.

a+b = 180 so a = 180-b .

c+b = 180 so c = 180-b .

That means a = c .

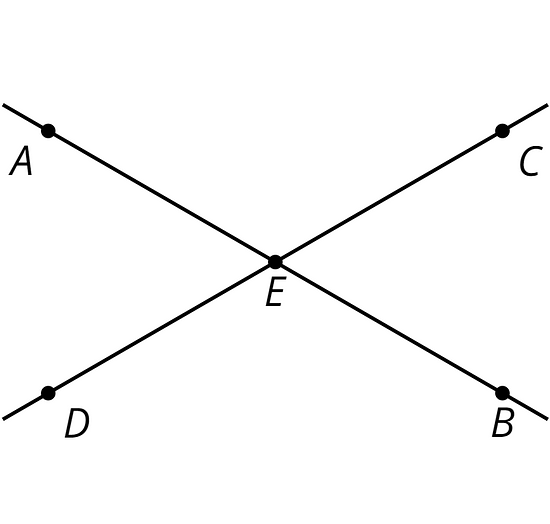
Glossary Terms

vertical angles

Vertical angles are opposite angles that share the same vertex. They are formed by a pair of intersecting lines. Their angle measures are equal.

For example, angles AEC and DEB are vertical angles. If angle AEC measure 120^\circ , then angle DEB must also measure 120^\circ .

Angles AED and BEC are another pair of vertical angles.

Two lines intersect at point E. There are also Points A, B, C, and D along the lines.

Lesson 3 Practice Problems

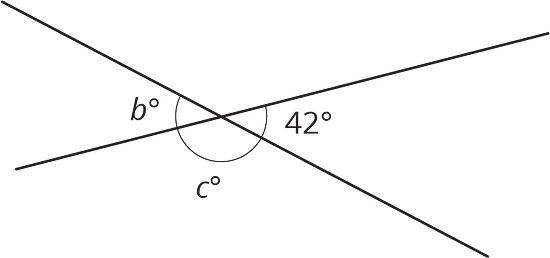
  1. Two lines intersect. Find the value of b and c .

    An angle of 42 degrees and angle c form a straight angle. Angle C and B also form a straight angle.

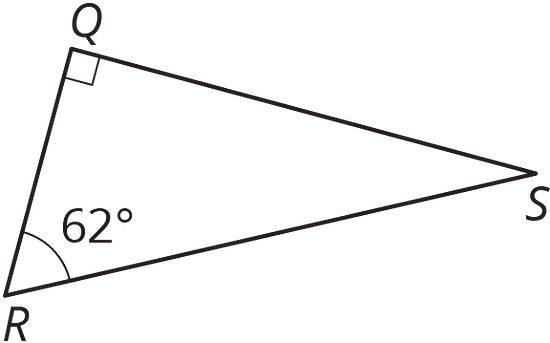
  2. In this figure, angles R and S are complementary. Find the measure of angle S .

    Right triangle QRS has a 62 degree angle.

  3. If two angles are both vertical and supplementary, can we determine the angles? Is it possible to be both vertical and complementary? If so, can you determine the angles? Explain how you know.

  4. Match each expression in the first list with an equivalent expression from the second list.

    1. 5(x+1) - 2x + 11
    2. 2x + 2 + x + 5
    3. \frac {\text{-}3}{8}x - 9 + \frac58x + 1
    4. 2.06x - 5.53 + 4.98 - 9.02
    5. 99x + 44
    1. \frac14x - 8
    2. \frac12(6x+14)
    3. 11(9x+4)
    4. 3x+16
    5. 2.06x +(\text-5.53) + 4.98 + (\text-9.02)

  5. Factor each expression.

    1. 15a-13a
    2. \text-6x-18y
    3. 36abc+54ad

  6. The directors of a dance show expect many students to participate but don’t yet know how many students will come. The directors need 7 students to work on the technical crew. The rest of the students work on dance routines in groups of 9. For the show to work, they need at least 6 full groups working on dance routines.

    1. Write and solve an inequality to represent this situation, and graph the solution on a number line.
    2. Write a sentence to the directors about the number of students they need.

  7. A small dog gets fed \frac{3}{4} cup of dog food twice a day. Using d for the number of days and f for the amount of food in cups, write an equation relating the variables. Use the equation to find how many days a large bag of dog food will last if it contains 210 cups of food.