5.1: A Rope and a Wheel
Han says that you can wrap a 5-foot rope around a wheel with a 2-foot diameter because \frac52 is less than pi. Do you agree with Han? Explain your reasoning.
Let’s explore how far different wheels roll.
Han says that you can wrap a 5-foot rope around a wheel with a 2-foot diameter because \frac52 is less than pi. Do you agree with Han? Explain your reasoning.
Your teacher will give you a circular object.
Follow these instructions to create the drawing:
Use your ruler to measure each distance. Record these values in the first row of the table:
| object | diameter | distance traveled in one rotation |
\text{distance} \div \text{diameter} | |
|---|---|---|---|---|
| row 1 | ||||
| row 2 | ||||
| row 3 |
A car wheel has a diameter of 20.8 inches.
About how far does the car wheel travel in 1 rotation? 5 rotations? 30 rotations?
Write an equation relating the distance the car travels in inches, c, to the number of wheel rotations, x.
About how many rotations does the car wheel make when the car travels 1 mile? Explain or show your reasoning.
A bike wheel has a radius of 13 inches.
About how far does the bike wheel travel in 1 rotation? 5 rotations? 30 rotations?
Write an equation relating the distance the bike travels in inches, b, to the number of wheel rotations, x.
About how many rotations does the bike wheel make when the bike travels 1 mile? Explain or show your reasoning.
Here are some photos of a spring toy.
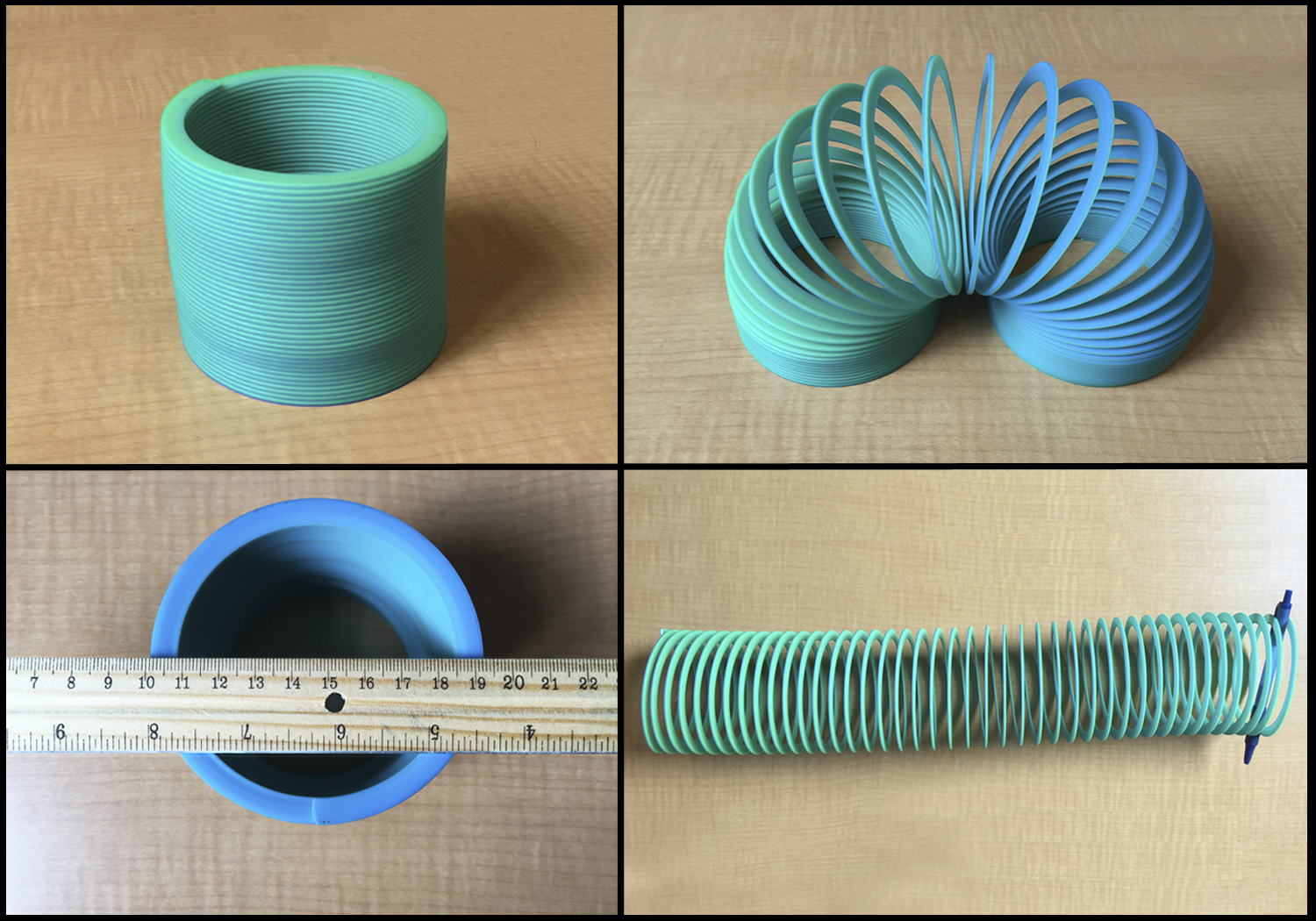
The circumference of a car wheel is about 65 inches.
The circumference of a circle is the distance around the circle. This is also how far the circle rolls on flat ground in one rotation. For example, a bicycle wheel with a diameter of 24 inches has a circumference of 24\pi inches and will roll 24\pi inches (or 2\pi feet) in one complete rotation. There is an equation relating the number of rotations of the wheel to the distance it has traveled. To see why, let’s look at a table showing how far the bike travels when the wheel makes different numbers of rotations.
| number of rotations | distance traveled (feet) | |
|---|---|---|
| row 1 | 1 | 2\pi |
| row 2 | 2 | 4 \pi |
| row 3 | 3 | 6 \pi |
| row 4 | 10 | 20 \pi |
| row 5 | 50 | 100 \pi |
| row 6 | x | ? |
In the table, we see that the relationship between the distance traveled and the number of wheel rotations is a proportional relationship. The constant of proportionality is 2\pi. To find the missing value in the last row of the table, note that each rotation of the wheel contributes 2\pi feet of distance traveled. So after x rotations the bike will travel 2\pi x feet. If d is the distance, in feet, traveled when this wheel makes x rotations, we have the relationship: d = 2\pi x