Let’s examine some special angles.
Use the applet to answer the questions.
If you place three copies of the hexagon together so that one vertex from each hexagon touches the same point, as shown, they fit together without any gaps or overlaps. Use this to figure out the degree measure of the angle inside the hexagon pattern block.
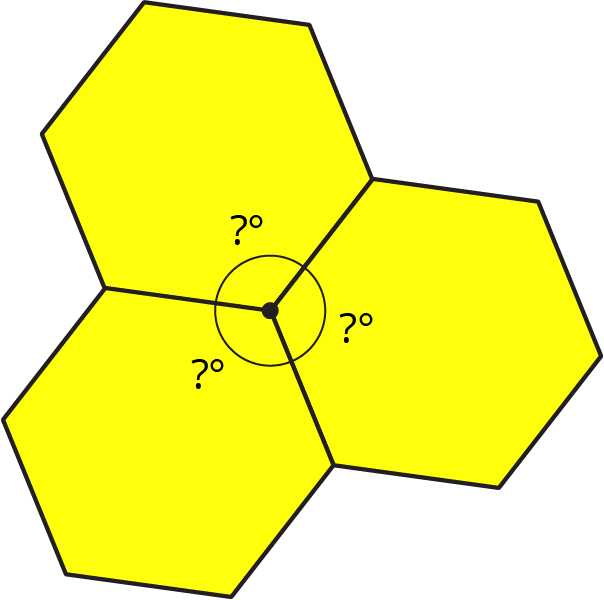
We saw that it is possible to fit three copies of a regular hexagon snugly around a point.
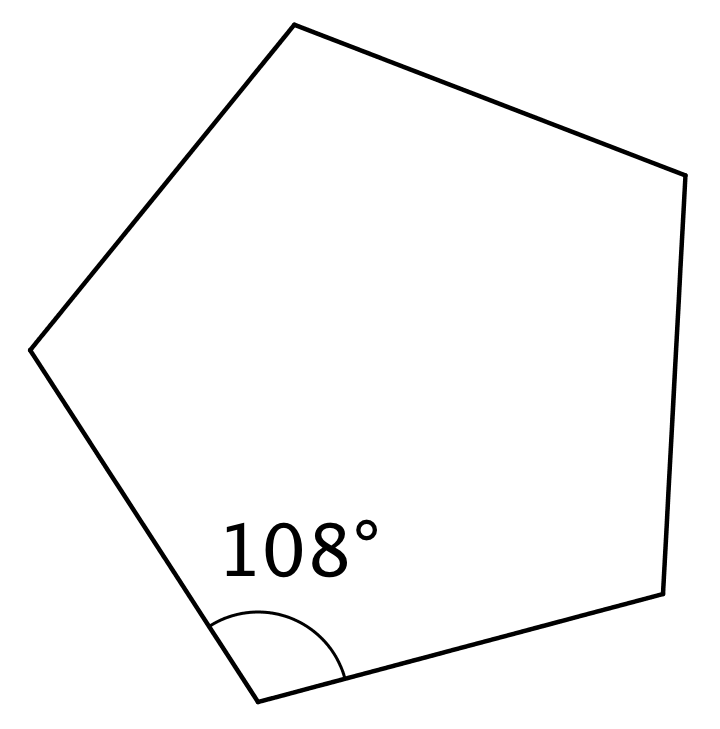
Each interior angle of a regular pentagon measures $108^\circ$. Is it possible to fit copies of a regular pentagon snugly around a point? If yes, how many copies does it take? If not, why not?
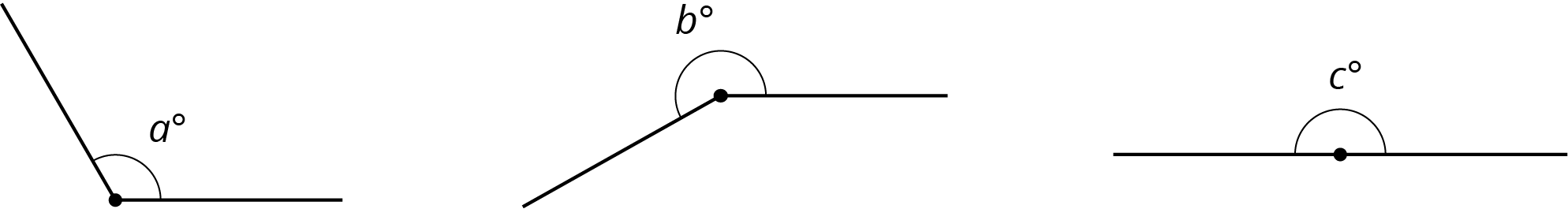
Use pattern blocks to determine the measure of each of these angles.
Use the applet if you choose. (Hint: turn on the grid to help align the pieces.)
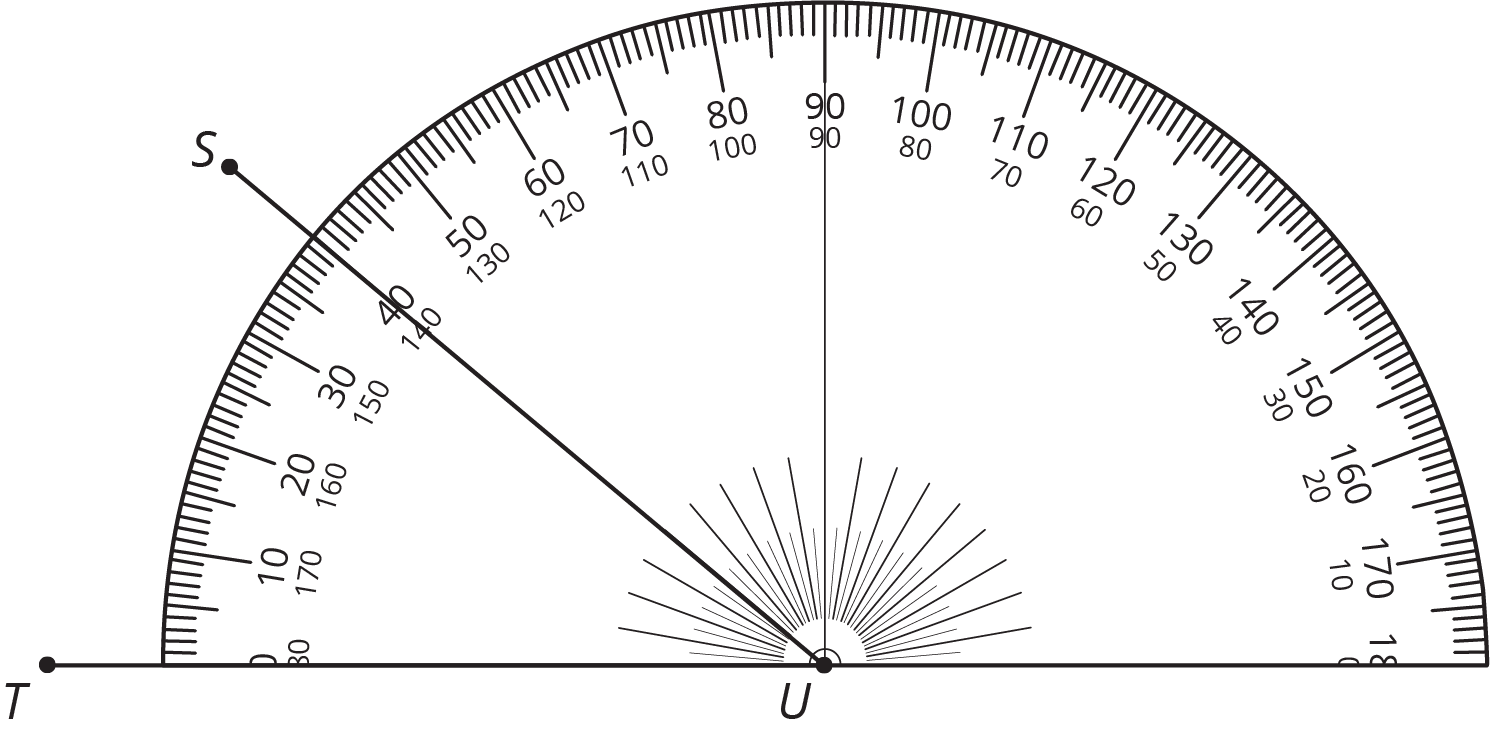
Tyler and Priya were both measuring angle $TUS$.
When two lines intersect and form four equal angles, we call each one a right angle. A right angle measures $90^\circ$. You can think of a right angle as a quarter turn in one direction or the other.
An angle in which the two sides form a straight line is called a straight angle. A straight angle measures $180^\circ$. A straight angle can be made by putting right angles together. You can think of a straight angle as a half turn, so that you are facing in the opposite direction after you are done.
If you put two straight angles together, you get an angle that is $360^\circ$. You can think of this angle as turning all the way around so that you are facing the same direction as when you started the turn.
When two angles share a side and a vertex, and they don't overlap, we call them adjacent angles.
Two angles are adjacent if they share a side and a vertex, and they don't overlap.
If the two rays that make an angle form a straight line, we call the angle a straight angle.
When you divide a straight angle into two angles with equal measures, each of the two angles is a right angle. For example, the four corners of a square are right angles.
