Lesson 2: Multiplying Powers of Ten

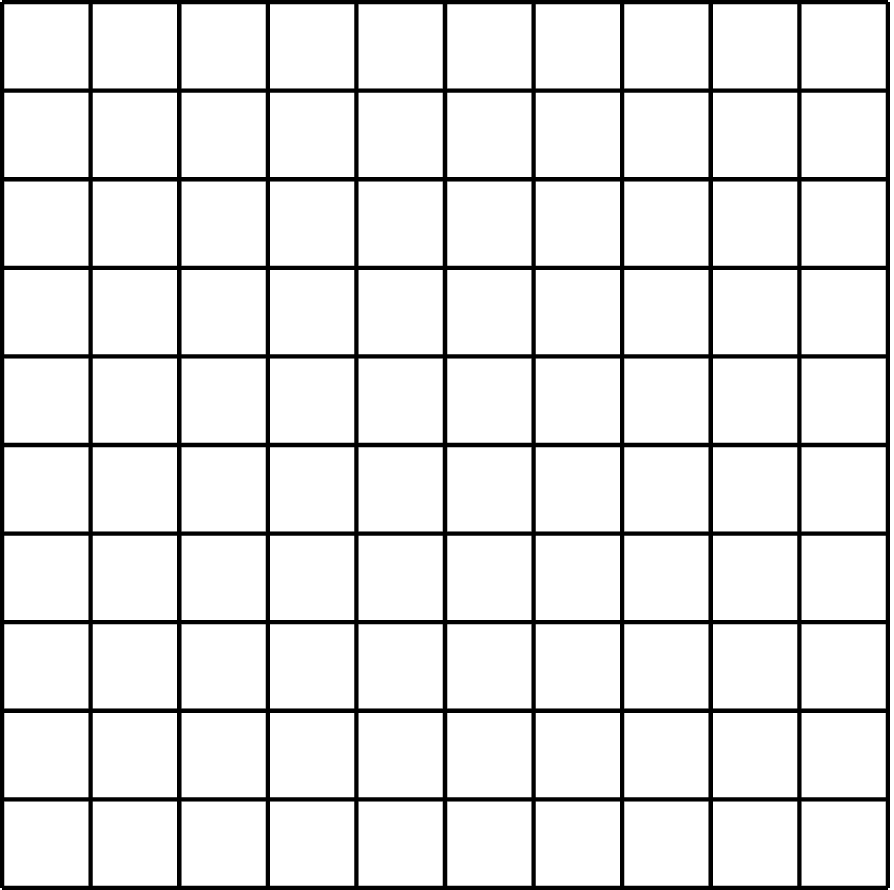
Clare said she sees 100.

Tyler says he sees 1.

Mai says she sees $\frac{1}{100}$.

In the diagram, the medium rectangle is made up of 10 small squares. The large square is made up of 10 medium rectangles.

1. How could you represent the large square as a power of 10?
2. If each small square represents $10^2$, then what does the medium rectangle represent? The large square?
3. If the medium rectangle represents $10^5$, then what does the large square represent? The small square?
4. If the large square represents $10^{100}$, then what does the medium rectangle represent? The small square?

1. 1. Complete the table to explore patterns in the exponents when multiplying powers of 10. You may skip a single box in the table, but if you do, be prepared to explain why you skipped it.

      	expression	expanded	single power of 10
      Row 1	$10^2 \boldcdot 10^3$	$(10 \boldcdot 10)(10\boldcdot 10 \boldcdot 10)$	$10^5$
      Row 2	$10^4 \boldcdot 10^3$
      Row 3	$10^4 \boldcdot 10^4$
      Row 4		$(10 \boldcdot 10 \boldcdot 10)(10 \boldcdot 10 \boldcdot 10 \boldcdot 10 \boldcdot 10)$
      Row 5	$10^{18} \boldcdot 10^{23}$

   2. If you chose to skip one entry in the table, which entry did you skip? Why?
2. 1. Use the patterns you found in the table to rewrite $10^n \boldcdot 10^m$ as an equivalent expression with a single exponent, like $10^{\boxed{\phantom{3}}}$.
   2. Use your rule to write $10^4 \boldcdot 10^0$ with a single exponent.  What does this tell you about the value of $10^0$?
3. The state of Georgia has roughly $10^7$ human residents. Each human has roughly $10^{13}$ bacteria cells in his or her digestive tract. How many bacteria cells are there in the digestive tracts of all the humans in Georgia?