Lesson 8: Rotation Patterns

Here is a right isosceles triangle:

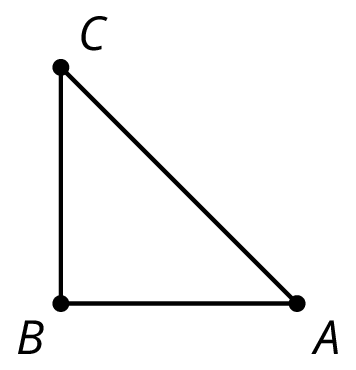
1. Rotate triangle $ABC$ 90 degrees clockwise around $B$. 
2. Rotate triangle $ABC$ 180 degrees clockwise round $B$.
3. Rotate triangle $ABC$ 270 degrees clockwise around $B$.
4. What would it look like when you rotate the four triangles 90 degrees clockwise around $B$? 180 degrees? 270 degrees clockwise?

Create a segment $AB$ and a point $C$ that is not on segment $AB$.

1. Rotate segment $AB$ $180^\circ$ around point $B$. 

2. Rotate segment $AB$ $180^\circ$ around point $C$. 

Construct the midpoint of segment $AB$ with the Midpoint tool. 

3. Rotate segment $AB$ $180^\circ$ around its midpoint. What is the image of A?

4. What happens when you rotate a segment $180^\circ$?

Here is a diagram built with three different rigid transformations of triangle $ABC$.

Use the applet to answer the questions. It may be helpful to reset the image after each question.

1. Describe a rigid transformation that takes triangle $ABC$ to triangle $CDE$.
2. Describe a rigid transformation that takes triangle $ABC$ to triangle $EFG$.
3. Describe a rigid transformation that takes triangle $ABC$ to triangle $GHA$.
4. Do segments $AC$, $CE$, $EG$, and $GA$ all have the same length? Explain your reasoning.