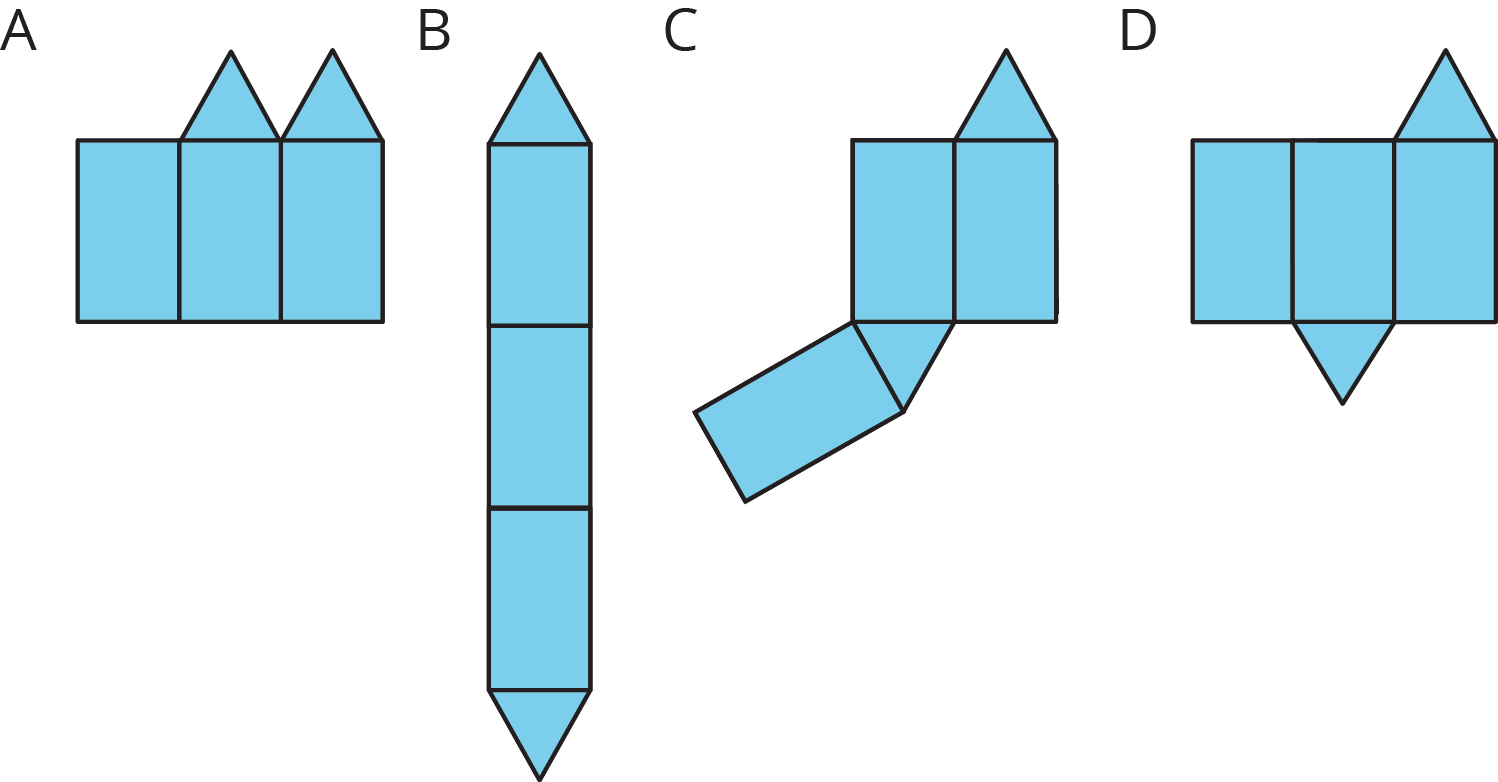
14.1: Matching Nets
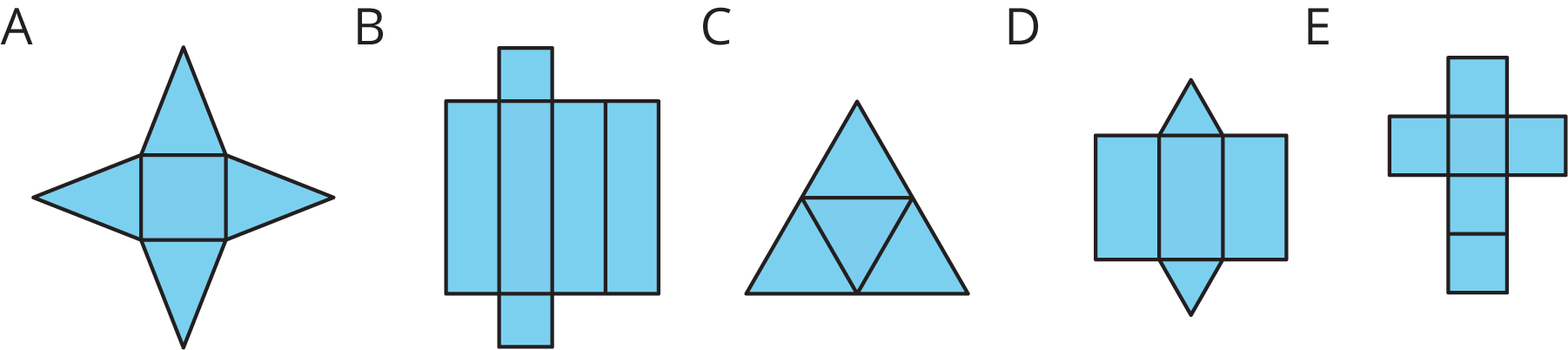
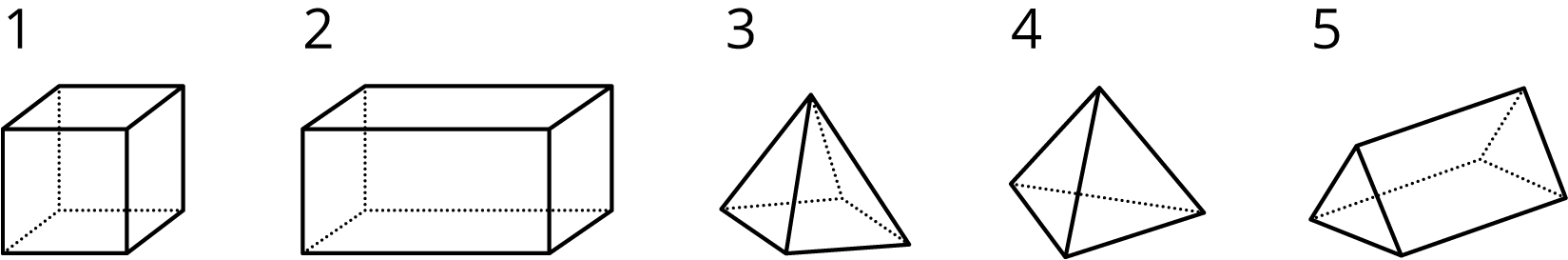
Each of the following nets can be assembled into a polyhedron. Match each net with its corresponding polyhedron, and name the polyhedron. Be prepared to explain how you know the net and polyhedron go together.
A:
Lesson 14: Nets and Surface Area
Let’s use nets to find the surface area of polyhedra.
14.2: Using Nets to Find Surface Area
Your teacher will give you the nets of three polyhedra to cut out and assemble.
- Name the polyhedron that each net would form when assembled.
B:
C:
-
Cut out your nets and use them to create three-dimensional shapes.
- Find the surface area of each polyhedron. Explain your reasoning clearly.
-
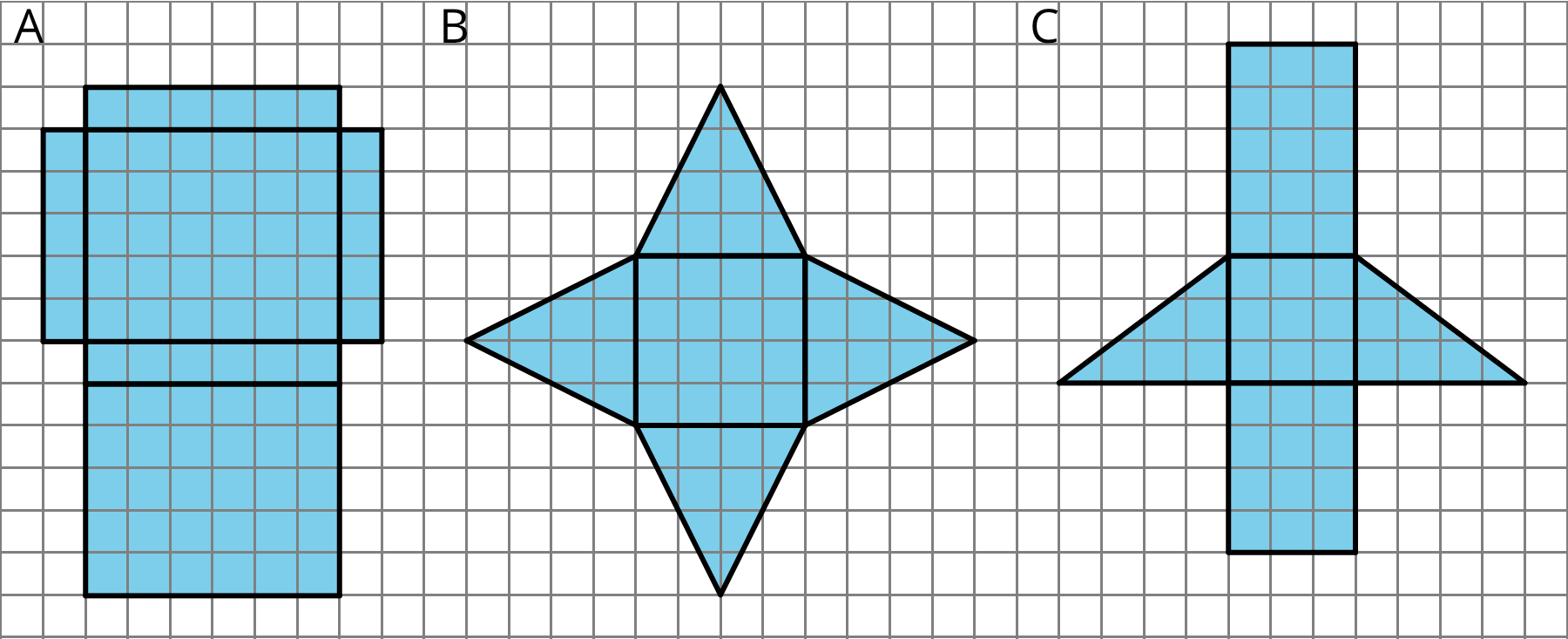
For each of these nets, decide if it can be assembled into a rectangular prism.
-
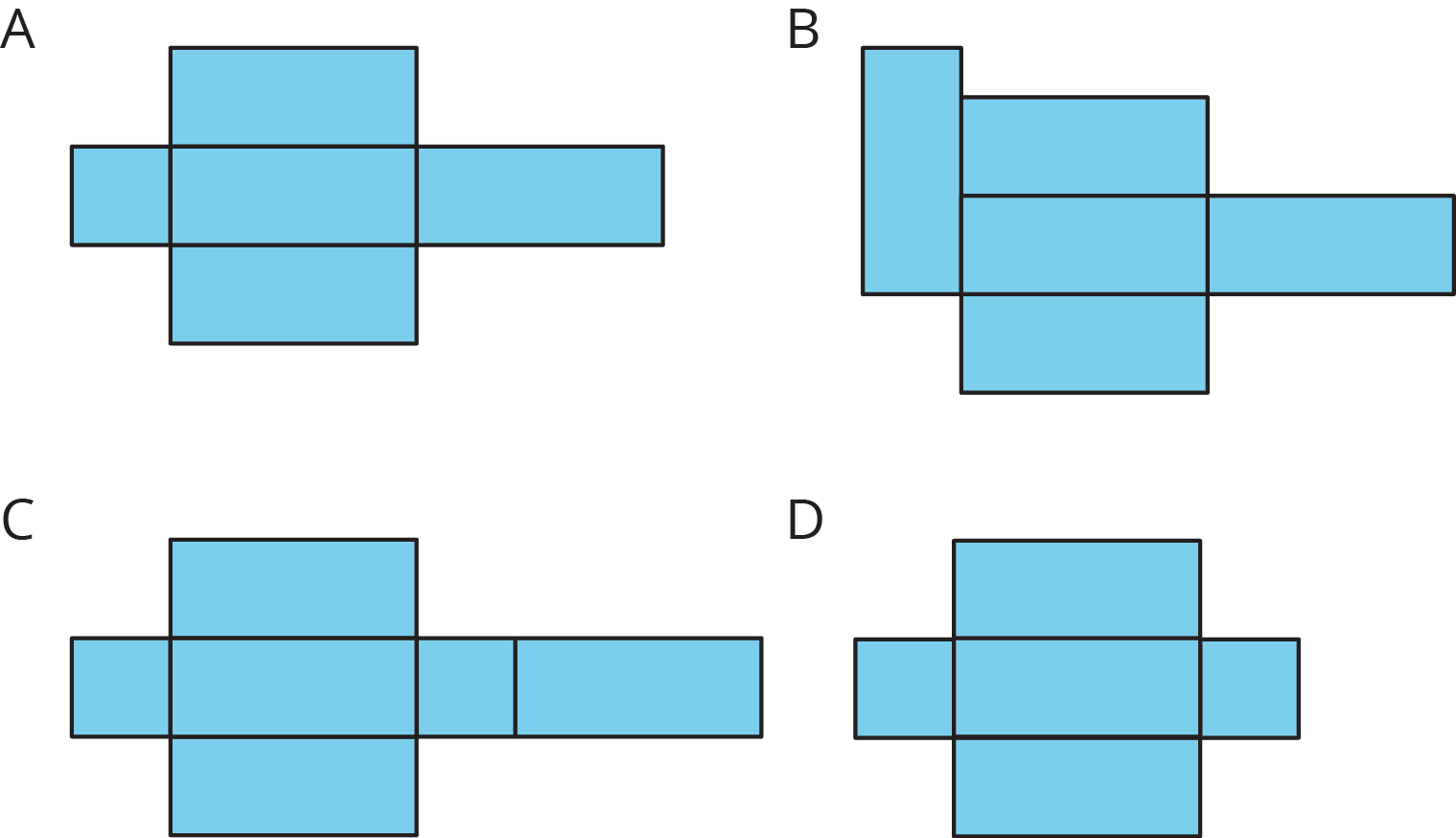
For each of these nets, decide if it can be folded into a triangular prism.
Summary
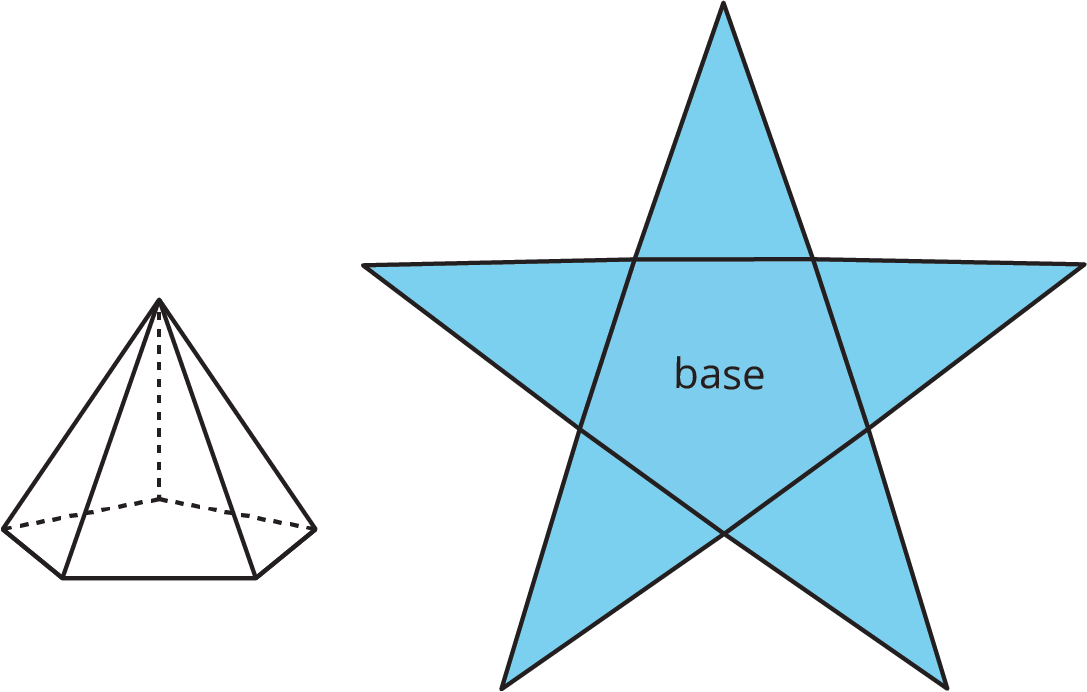
A net of a pyramid has one polygon that is the base. The rest of the polygons are triangles. A pentagonal pyramid and its net are shown here.
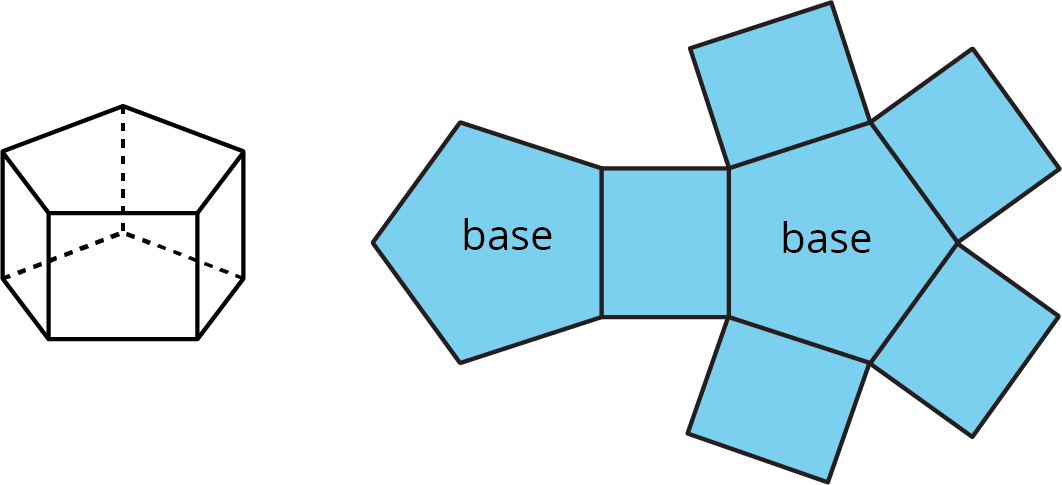
A net of a prism has two copies of the polygon that is the base. The rest of the polygons are rectangles. A pentagonal prism and its net are shown here.
In a rectangular prism, there are three pairs of parallel and identical rectangles. Any pair of these identical rectangles can be the bases.
Because a net shows all the faces of a polyhedron, we can use it to find its surface area.
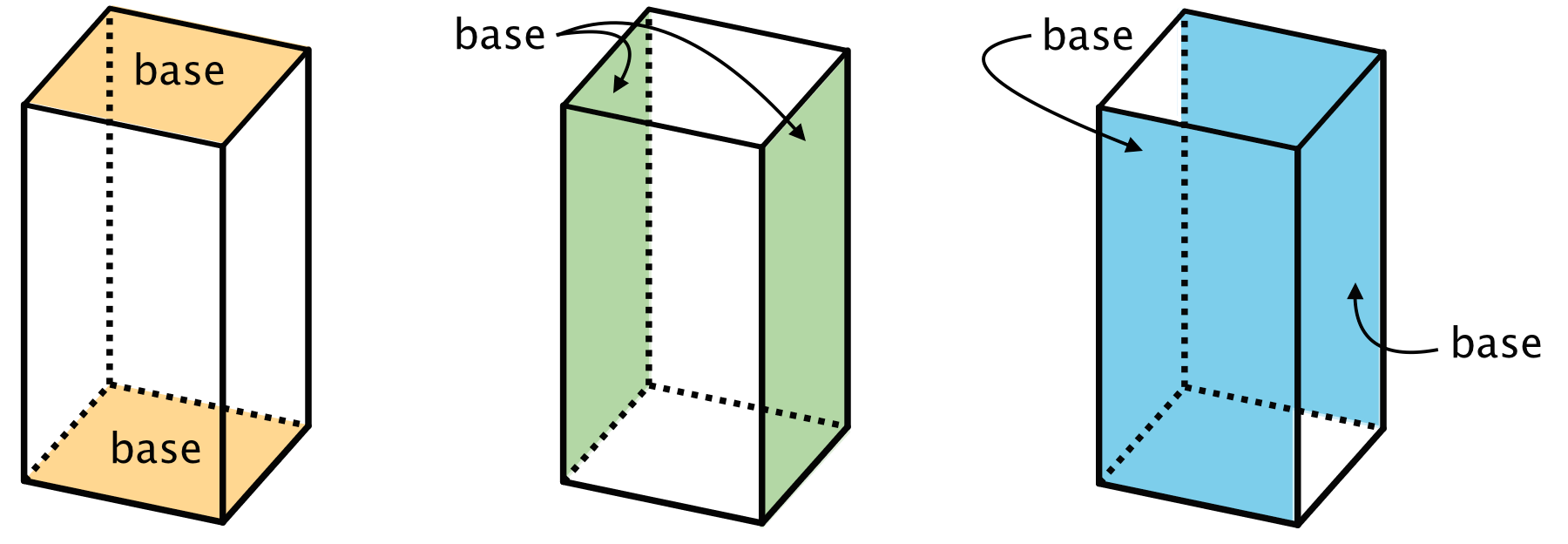
For instance, the net of a rectangular prism shows three pairs of rectangles: 4 units by 2 units, 3 units by 2 units, and 4 units by 3 units.
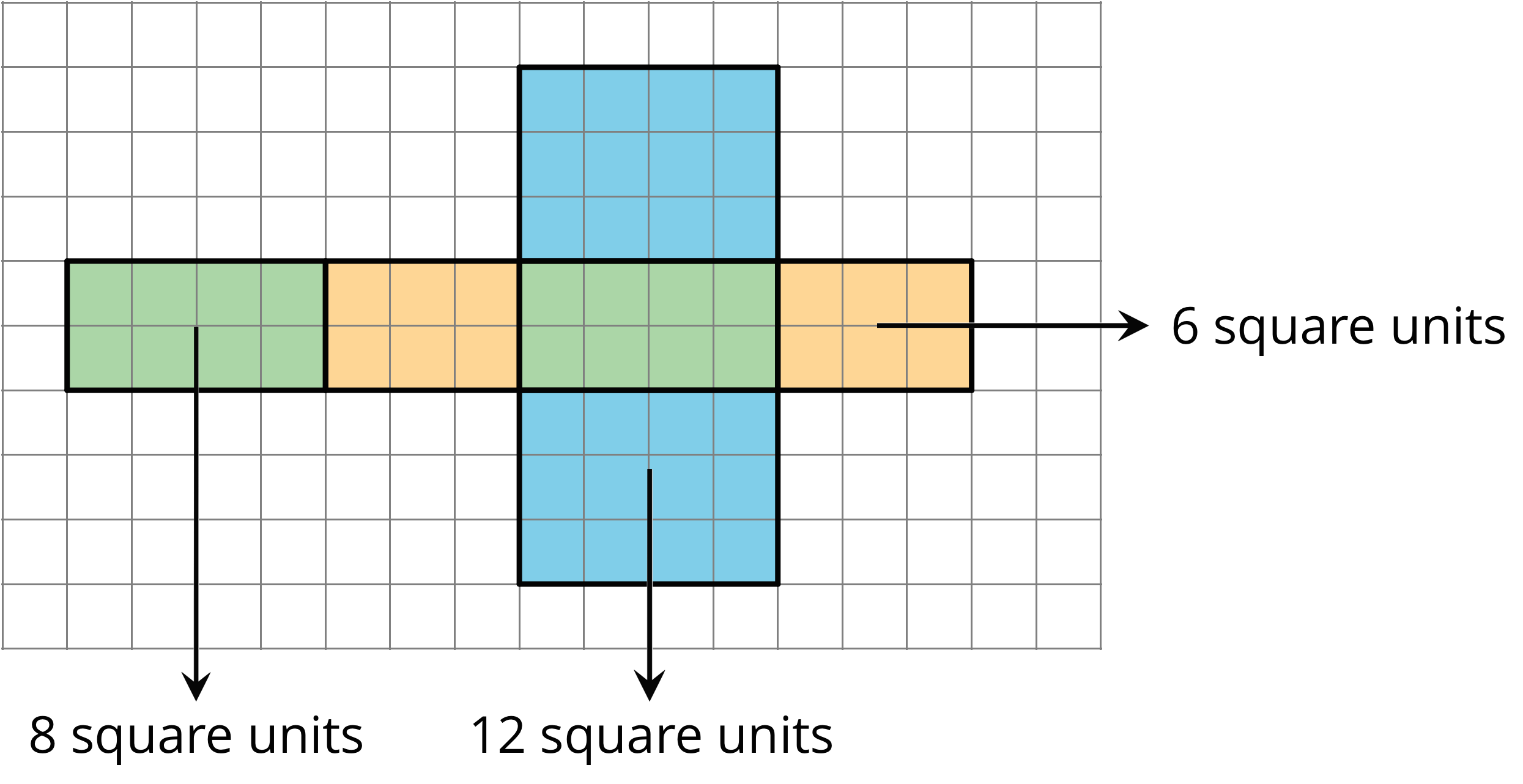
The surface area of the rectangular prism is 52 square units because $8+8+6+6+12+12=52$.