Lesson 1: Introducing Ratios and Ratio Language

Let’s describe two quantities at the same time.

1.1: What Kind and How Many?

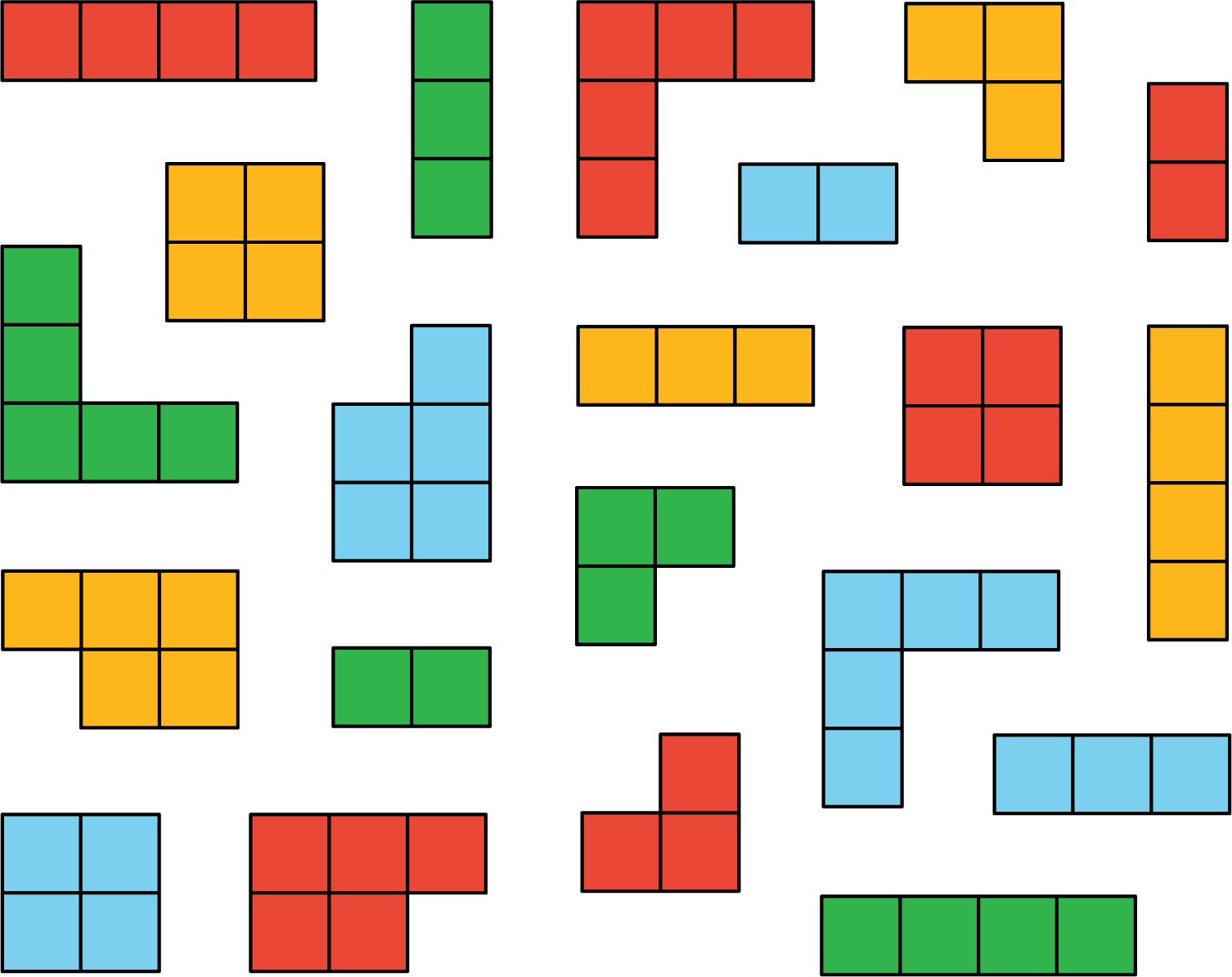
  1. If you sorted this set by color, how many groups would you have?
  2. If you sorted this set by area, how many groups would you have?
  3. Think of a third way you could sort these figures. What categories would you use? How many groups would you have?

1.2: The Teacher’s Collection

  1. Think of a way to sort your teacher’s collection into two or three categories. Record your categories in the top row of the table and the amounts in the second row.
    category name      
    category amount      

    Pause here so your teacher can review your work.

  2. Write at least two sentences that describe ratios in the collection. Remember, there are many ways to write a ratio:

    • The ratio of one category to another category is ________ to ________.

    • The ratio of one category to another category is ________ : ________.

    • There are _______ of one category for every _______ of another category.

1.3: The Student’s Collection

  1. Sort your collection into three categories. You can experiment with different ways of arranging these categories. Then, count the items in each category, and record the information in the table.

    category name      
    category amount      

  2. Write at least two sentences that describe ratios in the collection. Remember, there are many ways to write a ratio:

    • The ratio of one category to another category is ________ to ________.
    • The ratio of one category to another category is ________ : ________.

    • There are _______ of one category for every _______ of another category.

    Pause here so your teacher can review your sentences.

  3. Make a visual display of your items that clearly shows one of your statements. Be prepared to share your display with the class.

Summary

A ratio is an association between two or more quantities. There are many ways to describe a situation in terms of ratios. For example, look at this collection:

A discrete diagram of squares and circles such that the top row contains 6 squares and the bottom row contains 3 circles.

Here are some correct ways to describe the collection:

  • The ratio of squares to circles is $6:3$.
  • The ratio of circles to squares is 3 to 6.

Notice that the shapes can be arranged in equal groups, which allow us to describe the shapes using other numbers.

A discrete diagram of 6 squares and 3 circles organized into 3 equal groups of 2 squares and 1 circle each.
  • There are 2 squares for every 1 circle.
  • There is 1 circle for every 2 squares.

Practice Problems ▶

Glossary

ratio

ratio

A ratio associates two or more quantities. Ratios can be described in words such as “3 to 2” and “3 for every 2” and “3 out of every 5” and “3 parts to 2 parts.” We write ratios with symbols like this: $3 : 2$.