Lesson 6: Methods for Multiplying Decimals

Let’s look at some ways we can represent multiplication of decimals.

6.1: Which One Doesn’t Belong: Products

Which expression doesn’t belong? Explain your reasoning.

A.  $2 \boldcdot (0.3)$

B.  $2 \boldcdot 3 \boldcdot (0.1)$

C.  $6 \boldcdot (0.1)$

D.  $(0.1) \boldcdot 6$

 

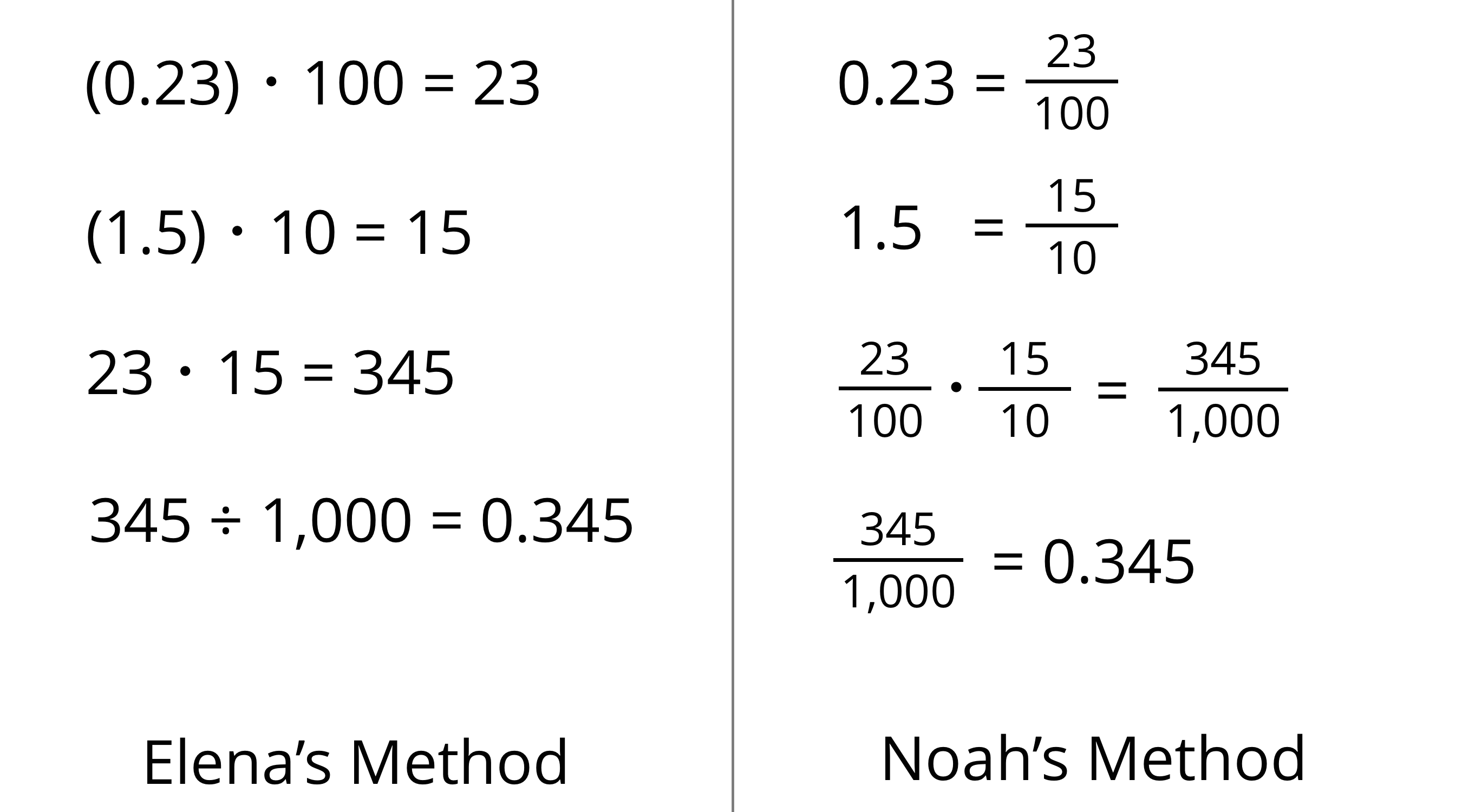
6.2: Using Properties of Numbers to Reason about Multiplication

  1. Elena and Noah used different methods to compute $(0.23) \boldcdot (1.5)$. Both computations were correct.

    Analyze the two methods, then discuss these questions with your partner.

    • Which method makes more sense to you? Why?
    • What might Elena do to compute $(0.16) \boldcdot (0.03)$? What might Noah do to compute $(0.16) \boldcdot (0.03)$? Will the two methods result in the same value?

  1. Compute each product using the equation $21 \boldcdot 47 = 987$ and what you know about fractions, decimals, and place value. Explain or show your reasoning.

    1. $(2.1) \boldcdot (4.7)$
    1. $21 \boldcdot (0.047)$
    1. $(0.021) \boldcdot (4.7)$

6.3: Using Area Diagrams to Reason about Multiplication

  1. In the diagram, the side length of each square is 0.1 unit.

    1. Explain why the area of each square is not 0.1 square unit.
    A rectangle is partitioned into 8 identical squares. There are 2 rows of 4 squares. The top horizontal side length of each square is labeled 0 point 1. The left vertical side length of each square is labeled 0 point 1.
    1. How can you use the area of each square to find the area of the rectangle? Explain or show your reasoning.
    2. Explain how the diagram shows that the equation $(0.4) \boldcdot (0.2) = 0.08$ is true.

  1. Label the squares with their side lengths so the area of this rectangle represents $40 \boldcdot 20$.

    1. What is the area of each square?
    2. Use the squares to help you find $40 \boldcdot 20$. Explain or show your reasoning. 
A rectangle area model. The rectangle is partitioned into 8 identical squares. There are 2 rows of 4 squares in each row.
  1. Label the squares with their side lengths so the area of this rectangle represents $(0.04) \boldcdot (0.02)$.

    Next, use the diagram to help you find $(0.04) \boldcdot (0.02)$. Explain or show your reasoning.

A rectangle area model. The rectangle is partitioned into 8 identical squares. There are 2 rows of 4 squares in each row.
 

Summary

Here are three other ways to calculate a product of two decimals such as $(0.04) \boldcdot (0.07)$. 

  • First, we can multiply each decimal by the same power of 10 to obtain whole-number factors.

    Because we multiplied both 0.04 and 0.07 by 100 to get 4 and 7, the product 28 is $(100 \boldcdot 100)$ times the original product, so we need to divide 28 by 10,000.

$$(0.04) \boldcdot 100 = 4$$ $$(0.07) \boldcdot 100 = 7$$ $$4 \boldcdot 7=28$$

$$28\div 10,\!000=0.0028$$

  • Second, we can write each decimal as a fraction, $0.04 = \frac{4}{100}$ and $0.07 = \frac{7}{100}$, and multiply them.  $$\frac{4}{100} \boldcdot \frac{7}{100} = \frac{28}{10,\!000}=0.0028$$

  • Third, we can use an area model. The product $(0.04) \boldcdot (0.07)$ can be thought of as the area of a rectangle with side lengths of 0.04 unit and 0.07 unit.

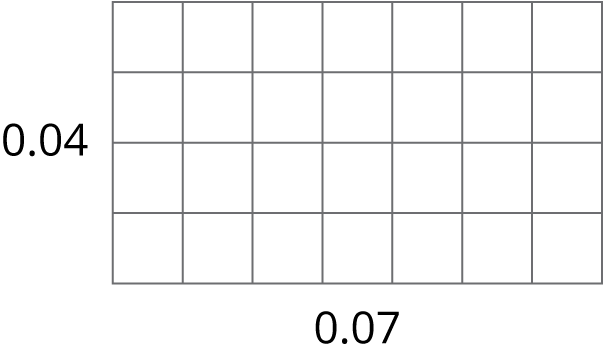
    An area model with horizontal length of 7 units and a vertical length of 4 units. The horizontal length is labeled 0.07 and the vertical length is labeled 0.04.

    In this diagram, each small square is 0.01 unit by 0.01 unit. Its area, in square units, is therefore $\left(\frac{1}{100} \boldcdot \frac{1}{100}\right)$, which is $\frac{1}{10,000}$.

    Because the rectangle is composed of 28 small squares, its area, in square units, must be: $$28 \boldcdot \frac{1}{10,000} = \frac{28}{10,000}=0.0028$$

All three calculations show that $(0.04) \boldcdot (0.07) = 0.0028$.

Practice Problems ▶