Let’s investigate the area of parallelograms some more.
Elena and Tyler were finding the area of this parallelogram:
Move the slider to see how Tyler did it:
Move the slider to see how Elena did it:
How are the two strategies for finding the area of a parallelogram the same? How they are different?
Each parallelogram has a side that is labeled “base.”
Study the examples and non-examples of bases and heights of parallelograms. Then, answer the questions that follow.
Examples: The dashed segment in each drawing represents the corresponding height for the given base.
Non-examples: The dashed segment in each drawing does not represent the corresponding height for the given base.
Select all statements that are true about bases and heights in a parallelogram.
In the applet, the parallelogram is made of solid line segments, and the height and supporting lines are made of dashed line segments. A base ($b$) and corresponding height ($h$) are labeled.
Experiment with dragging all of the movable points around the screen. Can you change the parallelogram so that . . .
For each parallelogram:
In the last row, write an expression using $b$ and $h$ for the area of any parallelogram.
| parallelogram | base (units) | height (units) | area (sq units) |
| A | |||
| B | |||
| C | |||
| D | |||
| any parallelogram | $b$ | $h$ |
If we draw any perpendicular segment from a point on the base to the opposite side of the parallelogram, that segment will always have the same length. We call that value the height. There are infinitely many line segments that can represent the height!
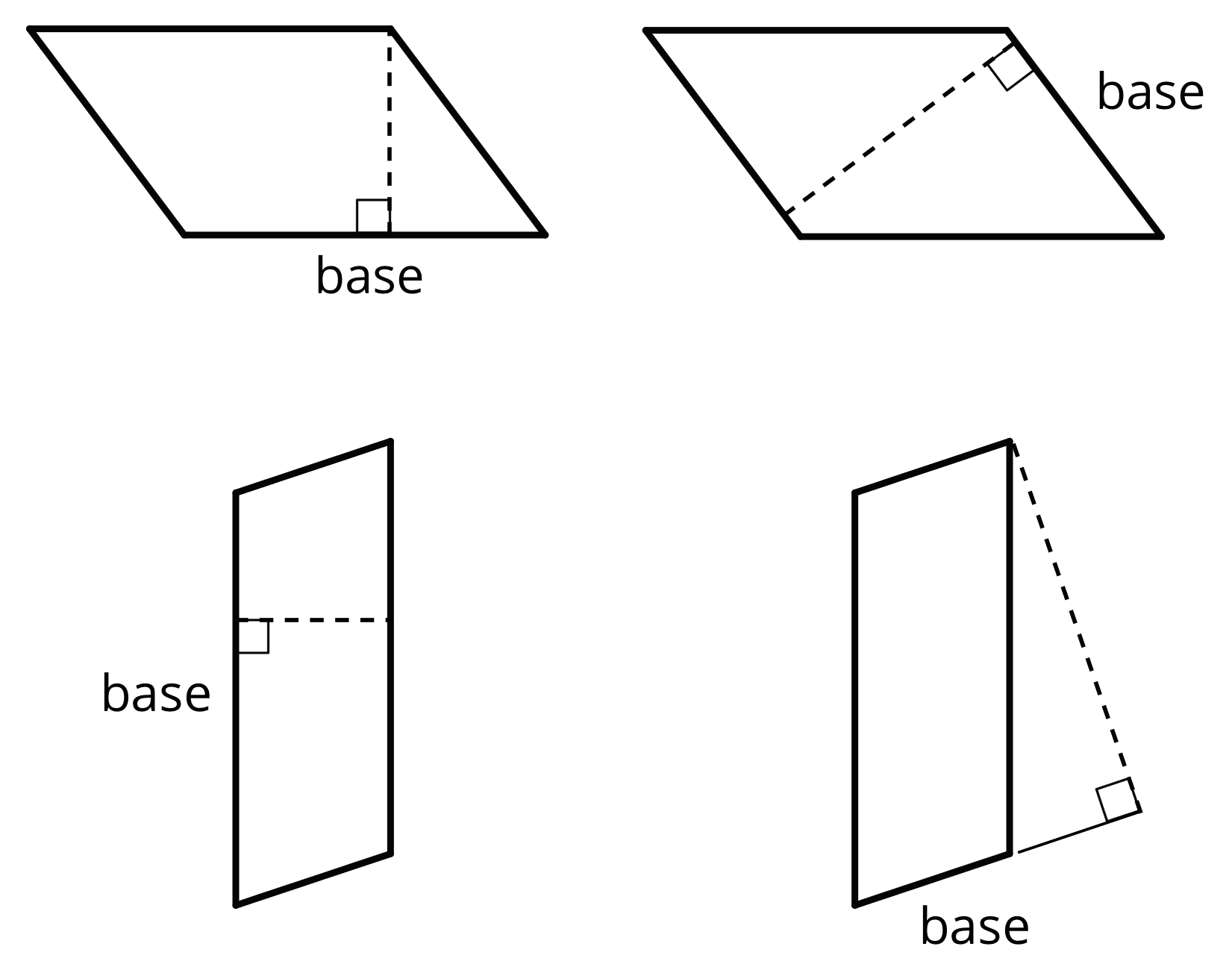
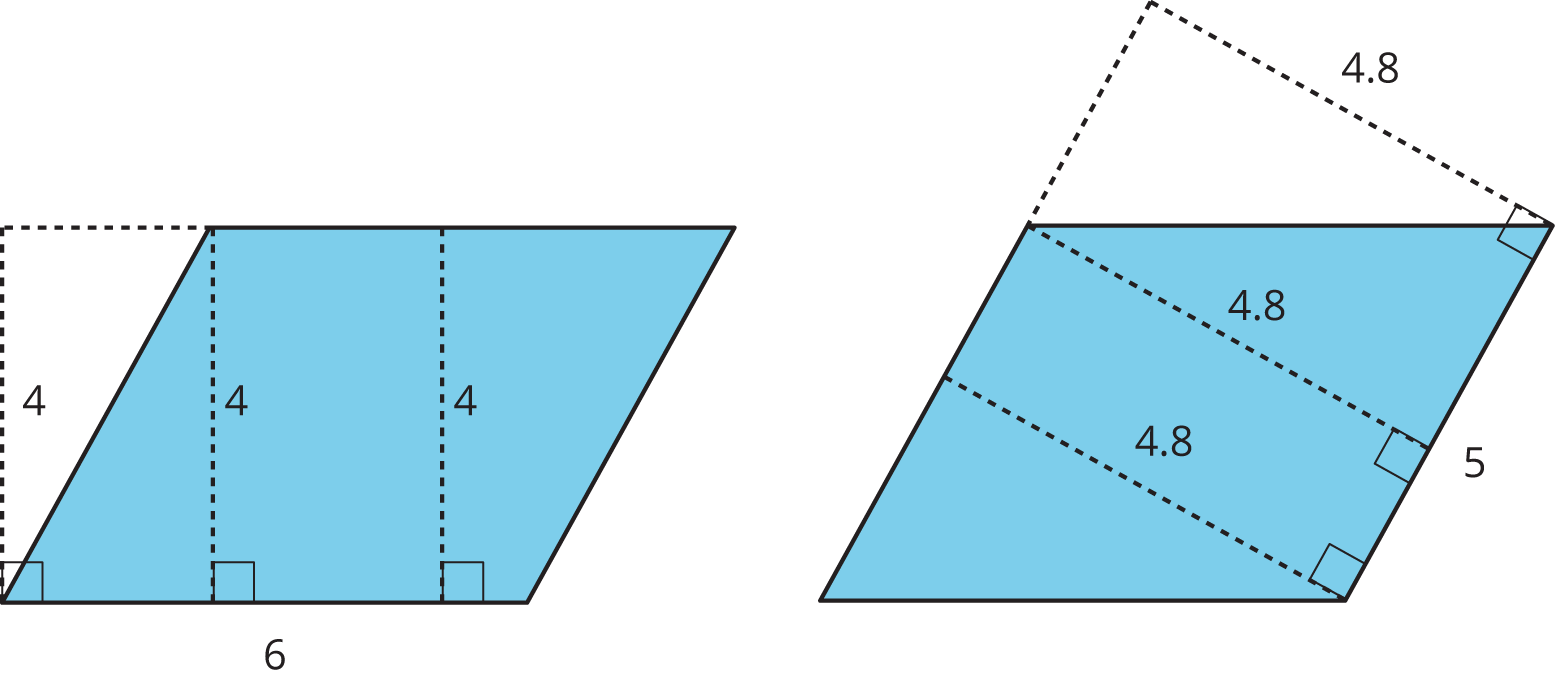
Here are two copies of the same parallelogram. On the left, the side that is the base is 6 units long. Its corresponding height is 4 units. On the right, the side that is the base is 5 units long. Its height is 4.8 units. For both, three different segments are shown to represent the height. We could draw in many more!
No matter which side is chosen as the base, the area of the parallelogram is the product of that base and its corresponding height. We can check it:
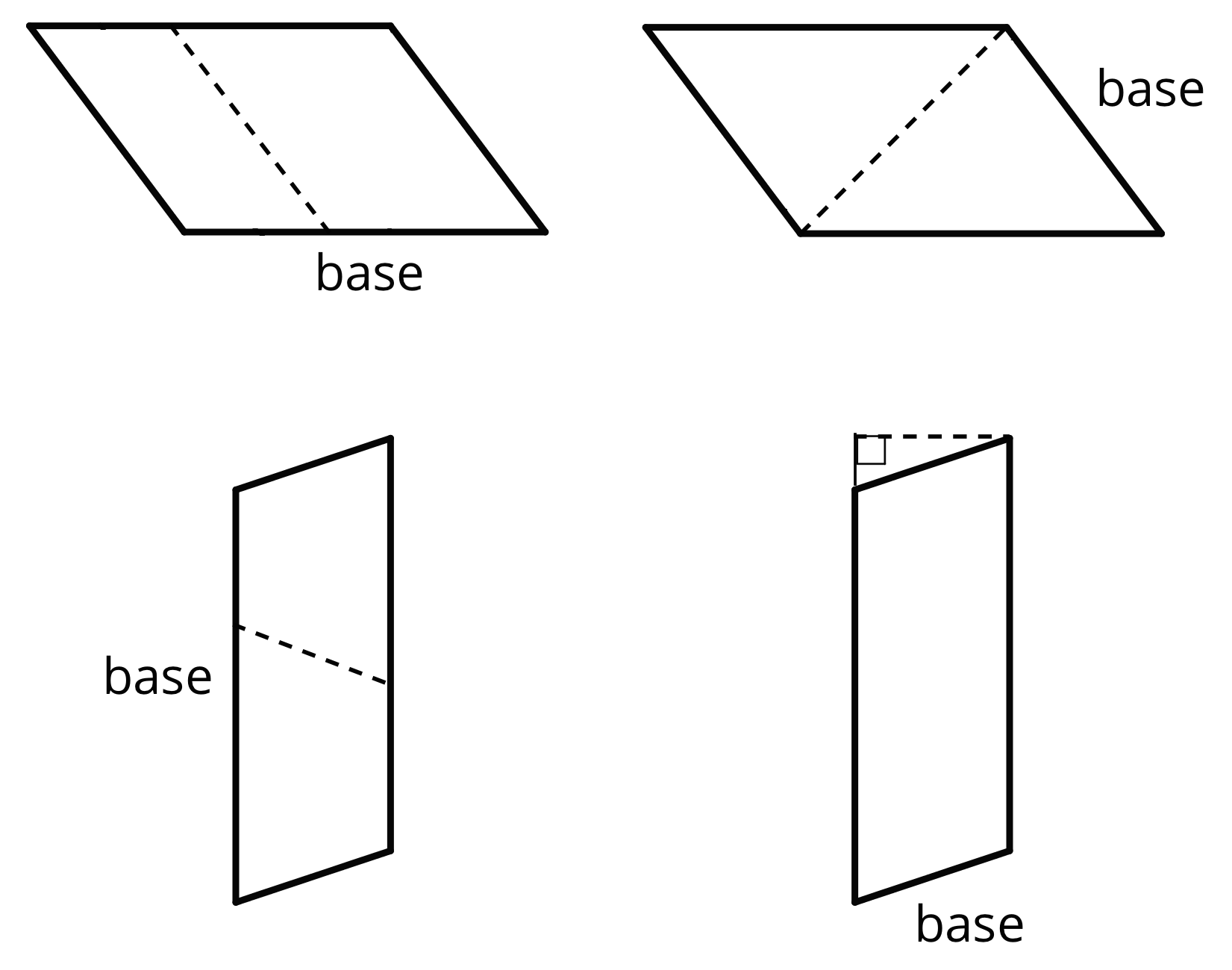
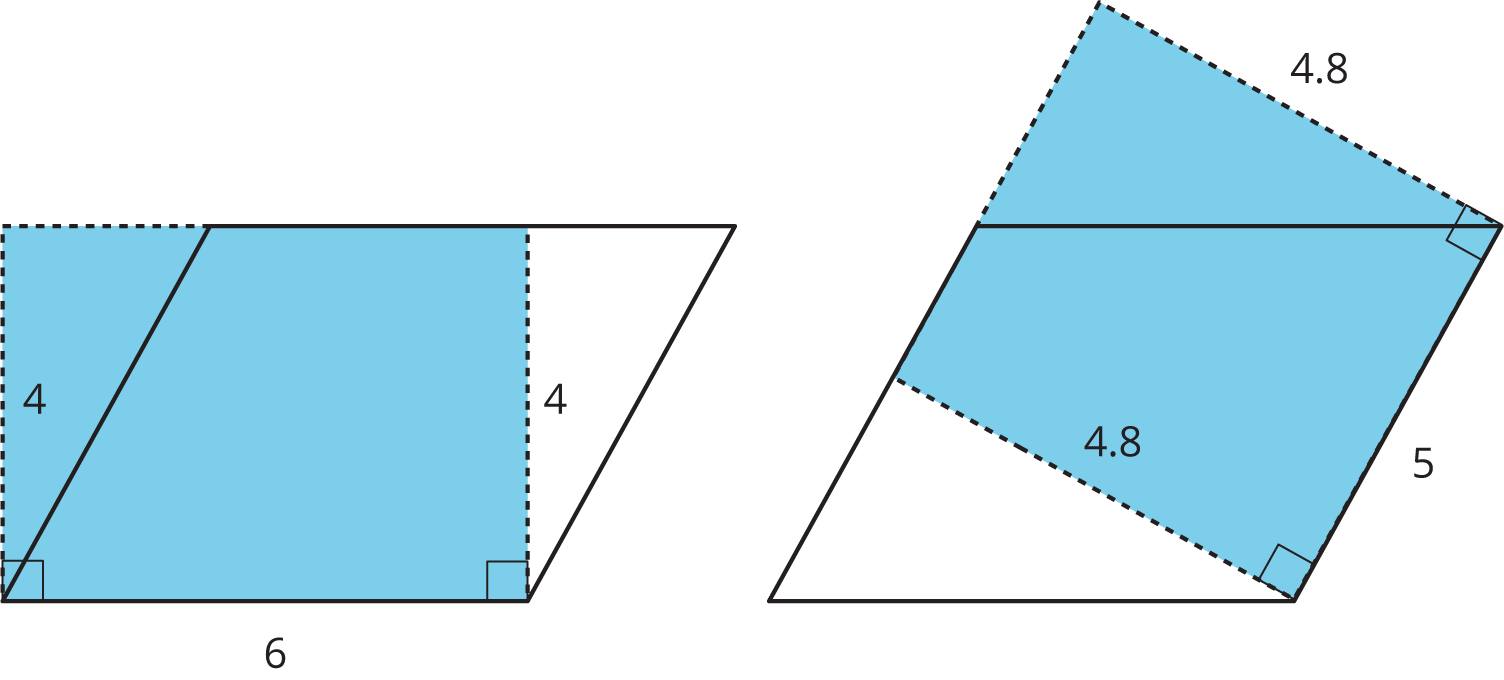
We can see why this is true by decomposing and rearranging the parallelograms into rectangles.
Notice that the side lengths of each rectangle are the base and height of the parallelogram. Even though the two rectangles have different side lengths, the products of the side lengths are equal, so they have the same area! And both rectangles have the same area as the parallelogram.
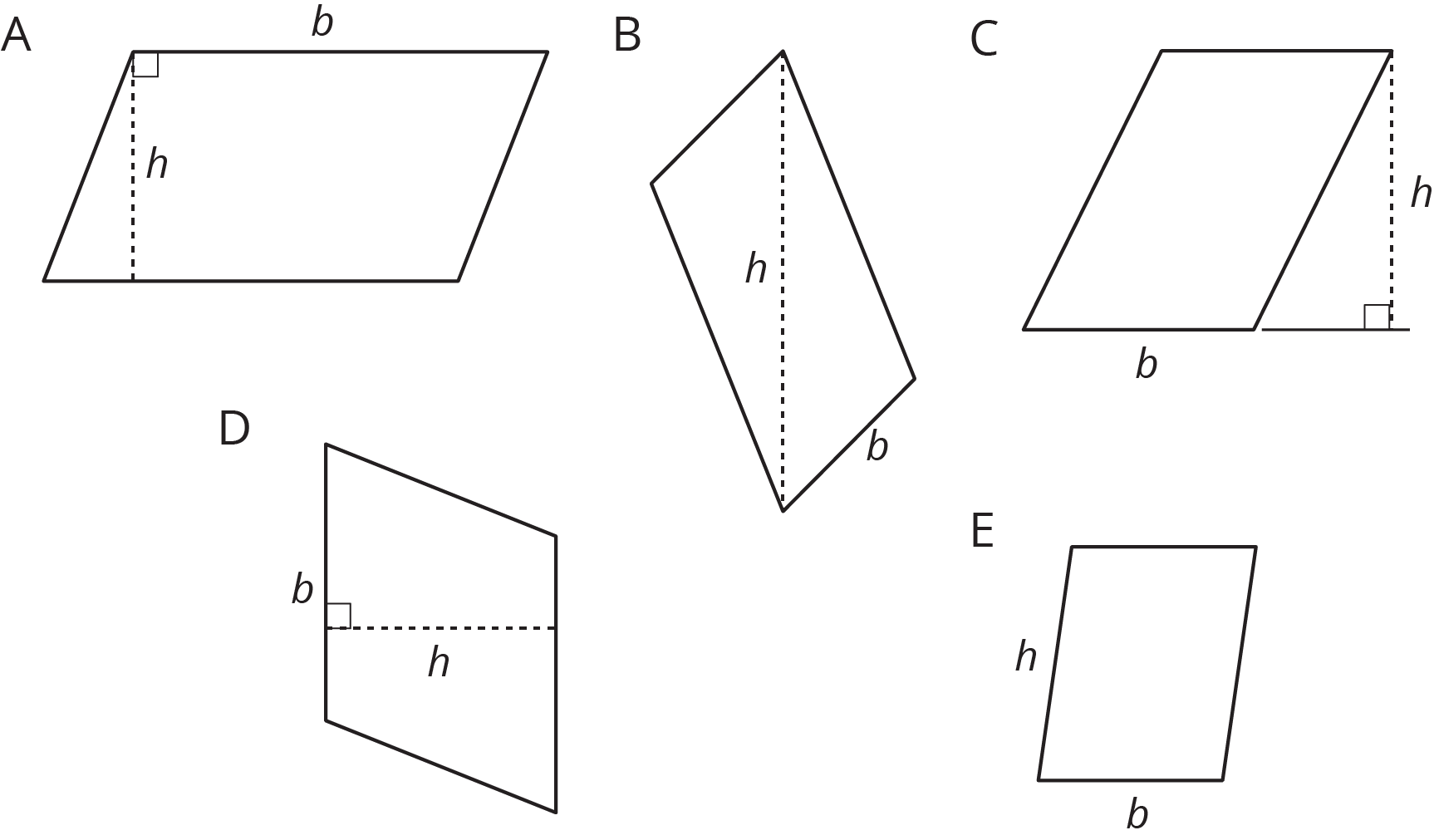
We often use letters to stand for numbers. If $b$ is base of a parallelogram (in units), and $h$ is the corresponding height (in units), then the area of the parallelogram (in square units) is the product of these two numbers. $$b \boldcdot h$$
Notice that we write the multiplication symbol with a small dot instead of a $\times$ symbol. This is so that we don’t get confused about whether $\times$ means multiply, or whether the letter $x$ is standing in for a number.
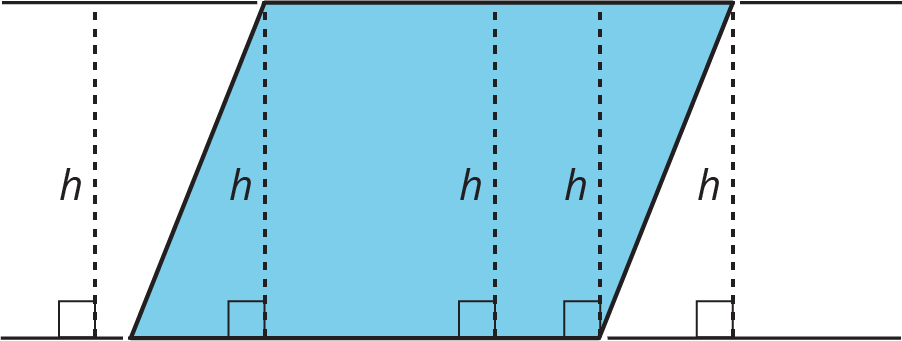
In high school, you will be able to prove that a perpendicular segment from a point on one side of a parallelogram to the opposite side will always have the same length.
You can see this most easily when you draw a parallelogram on graph paper. For now, we will just use this as a fact.
Any of the four sides of a parallelogram can be chosen as a base. The term base can also refer to the length of this side. Once we have chosen a base, then a perpendicular segment from a point on the base of a parallelogram to the opposite side will always have the same length. We call that value the height.
