Let’s use area diagrams to find products.
For each of the following products, choose the best estimate of its value. Be prepared to explain your reasoning.
$(6.8) \boldcdot (2.3)$
$74 \boldcdot (8.1)$
$166 \boldcdot (0.09)$
$(3.4) \boldcdot (1.9)$
Here are three ways of finding the area of a rectangle that is 24 units by 13 units.
Discuss with your partner:
You may be familiar with different ways to write multiplication calculations. Here are two ways to calculate 24 times 13.
Discuss with your partner:
Use the two following methods to find the product of 18 and 14, then compare the values obtained.
Here is a rectangle that is 18 units by 14 units. Find its area, in square units by decomposing it. Show your reasoning.
Compare the values of $18 \boldcdot 14$ that you obtained using the two methods. If they are not the same, check your work.
Use the applet to verify your answers and explore your own scenarios. In order to adjust the values, move the dots on the ends of the segments.
Which region represents $(0.4) \boldcdot (0.3)$? Label that region with its area of 0.12.
Label each of the other regions with their respective areas.
Find the value of $(2.4) \boldcdot (1.3)$. Show your reasoning.
Here are two ways of calculating 2.4 times 1.3.
Analyze the calculations and discuss with a partner:
Find the product of $(3.1) \boldcdot (1.5)$ by drawing and labeling an area diagram. Show your reasoning.
Show how to calculate $(3.1) \boldcdot (1.5)$ using numbers without a diagram. Be prepared to explain your reasoning. If you are stuck, use the examples in a previous question to help you.
Use the applet to verify your answers and explore your own scenarios. In order to adjust the values, move the dots on the ends of the segments.
How many hectares is the property of your school? How many morgens is that?
Label the area diagram to represent $(2.5) \boldcdot (1.2)$ and to find that product.
Here are two ways to calculate $(2.5) \boldcdot (1.2)$. Each number with a box gives the area of one or more regions in the area diagram.
In the boxes next to each number, write the letter(s) of the corresponding region(s).
In Calculation B, which two numbers are being multiplied to obtain 0.5? Which two are being multiplied to obtain 2.5?
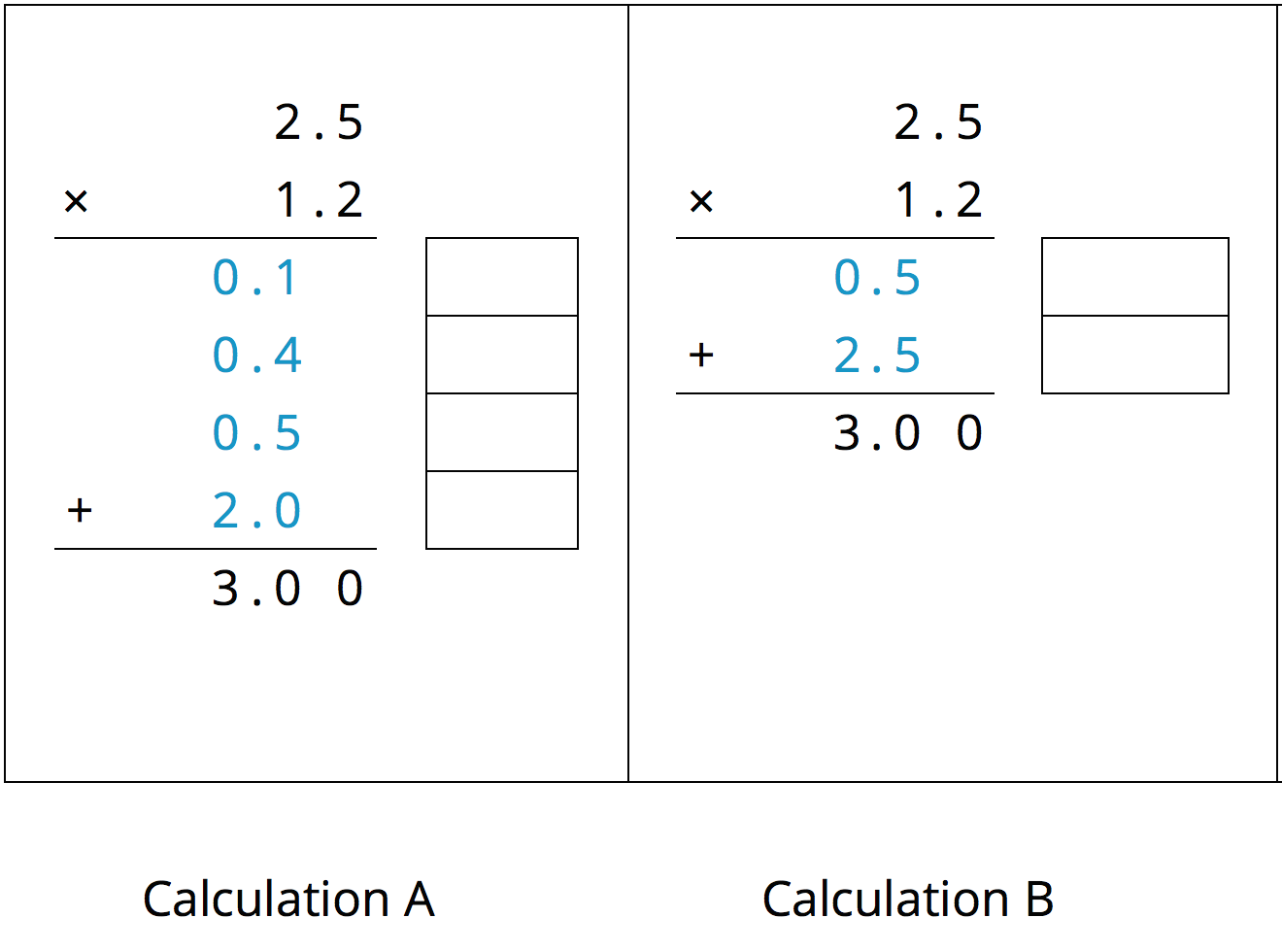
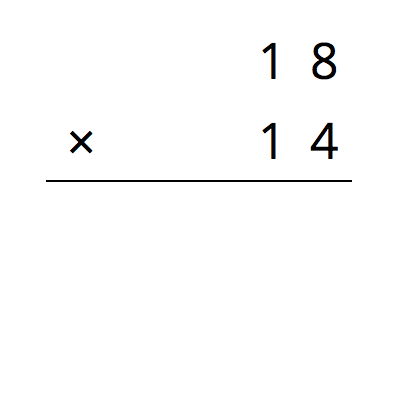Suppose that we want to calculate the product of two numbers that are written in base ten. To explain how, we can use what we know about base-ten numbers and areas of rectangles.
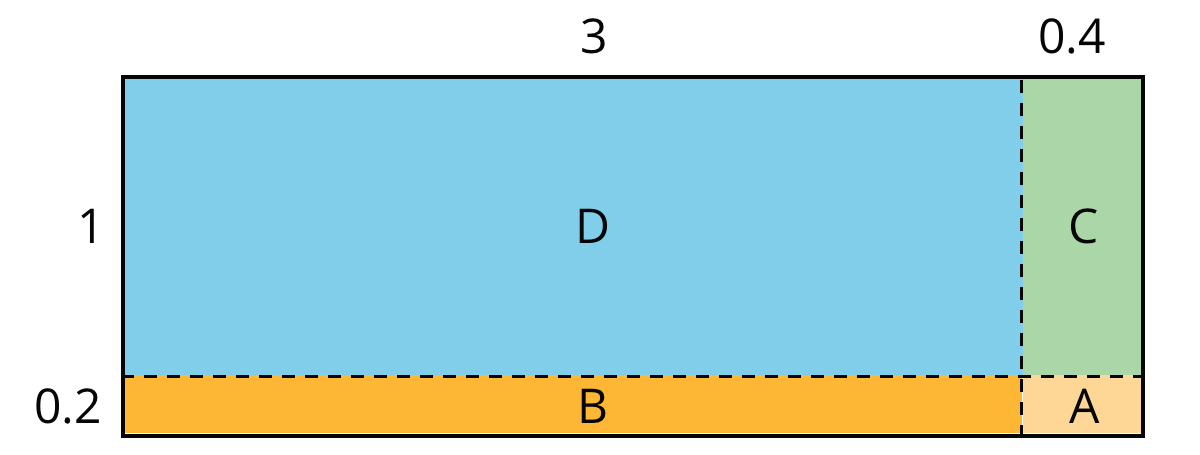
Here is a diagram of a rectangle whose side lengths are 3.4 units and 1.2 units. Its area, in square units, is the product
$(3.4) \boldcdot (1.2)$. To calculate this product and find the area of the rectangle, we can decompose each side length into its base-ten units, $3.4 = 3 + 0.4$ and $1.2= 1 + 0.2$, decomposing the rectangle into four smaller sub-rectangles.
We can rewrite the product and expand it twice:
\(\begin{align} (3.4) \boldcdot (1.2) &= (3 + 0.4) \boldcdot (1 + 0.2)\\ &=(3 + 0.4) \boldcdot 1 + (3 + 0.4) \boldcdot 0.2\\ &=3 \boldcdot 1+ 3 \boldcdot (0.2)+ (0.4) \boldcdot 1 + (0.4)\boldcdot (0.2)\\ \end{align}\)
In the last expression, each of the four terms is called a partial product. Each partial product gives the area of a sub-rectangle in the diagram. The sum of the four partial products gives the area of the entire rectangle.
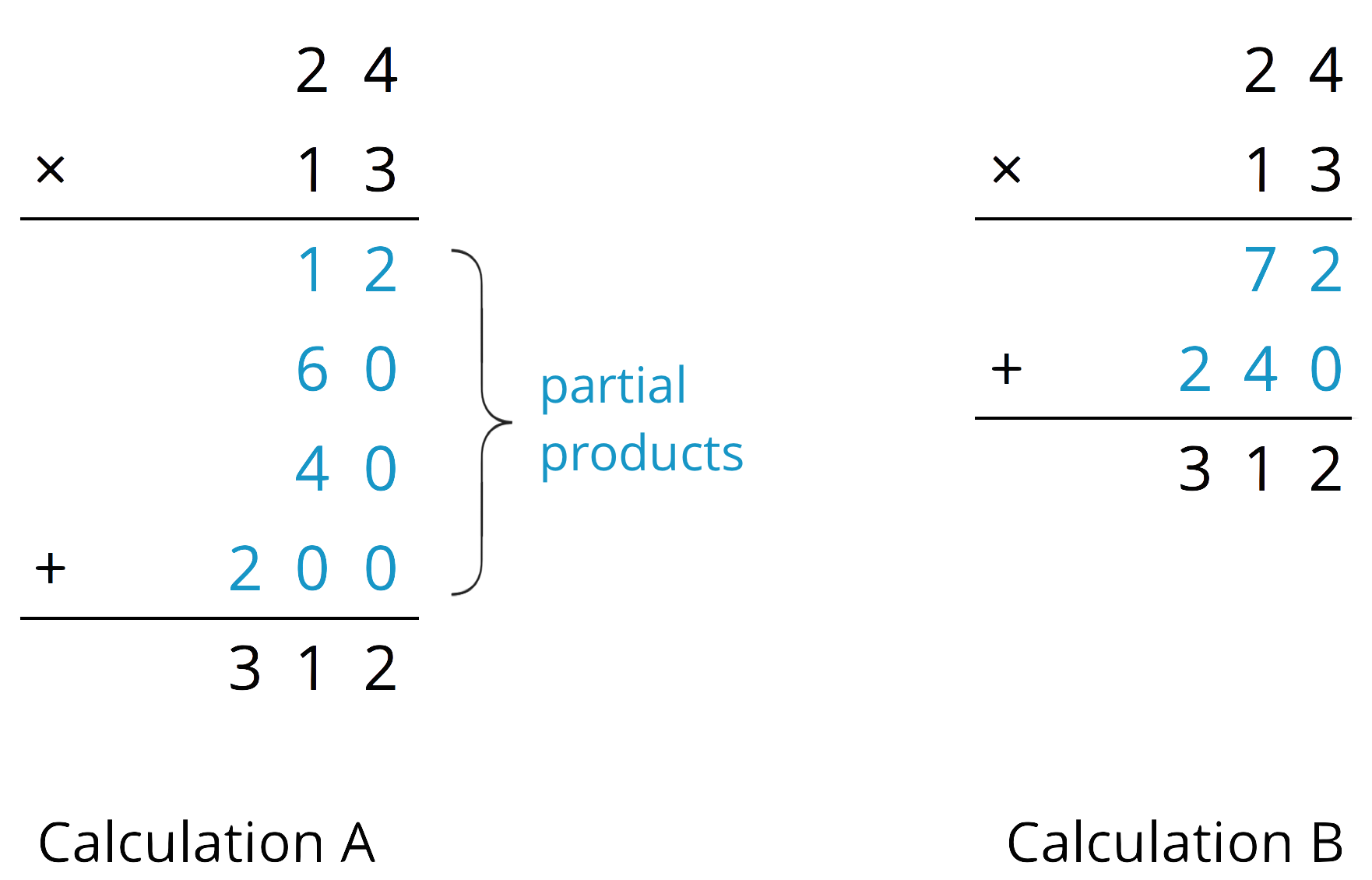
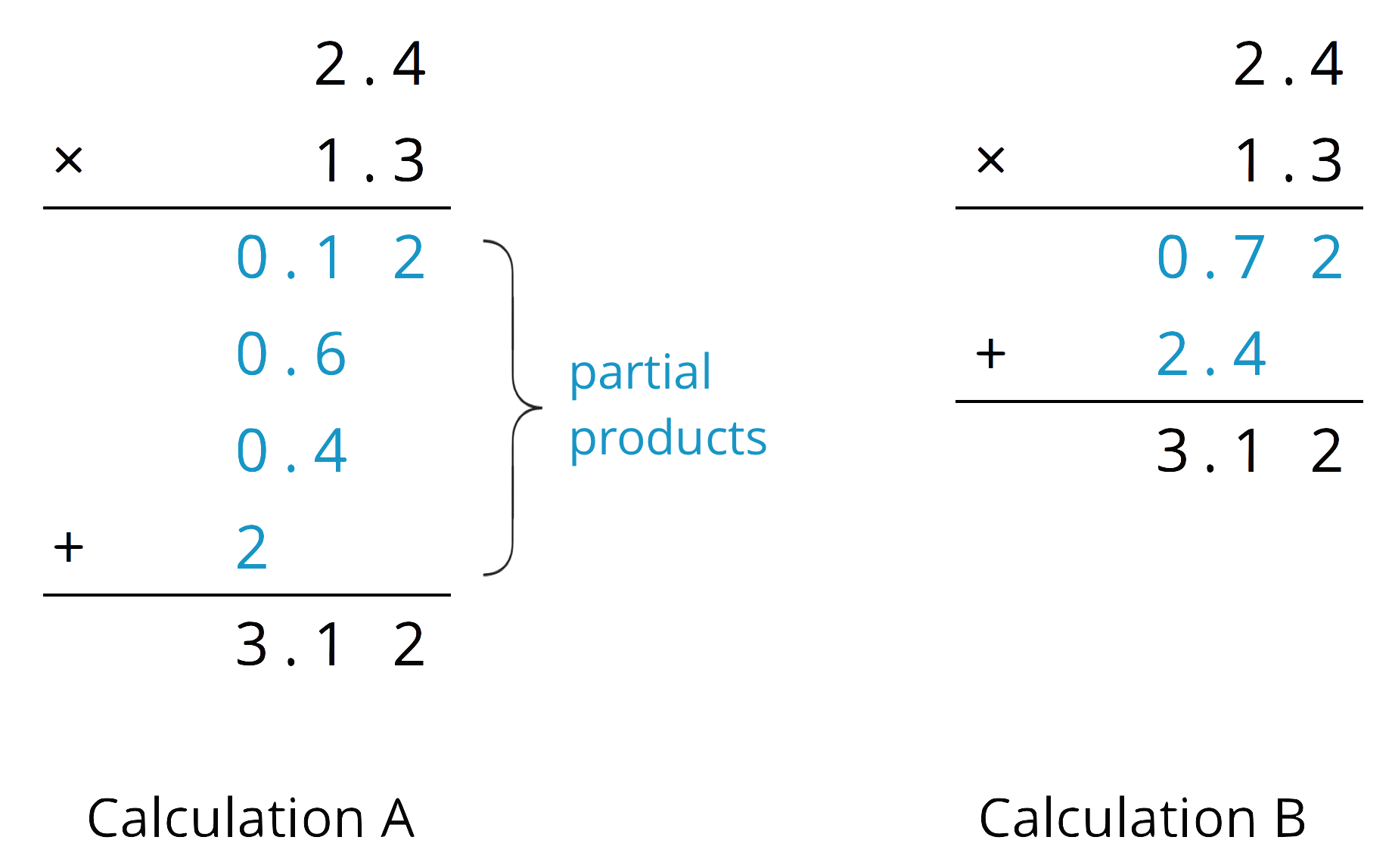
We can show the horizontal calculations above as two vertical calculations.
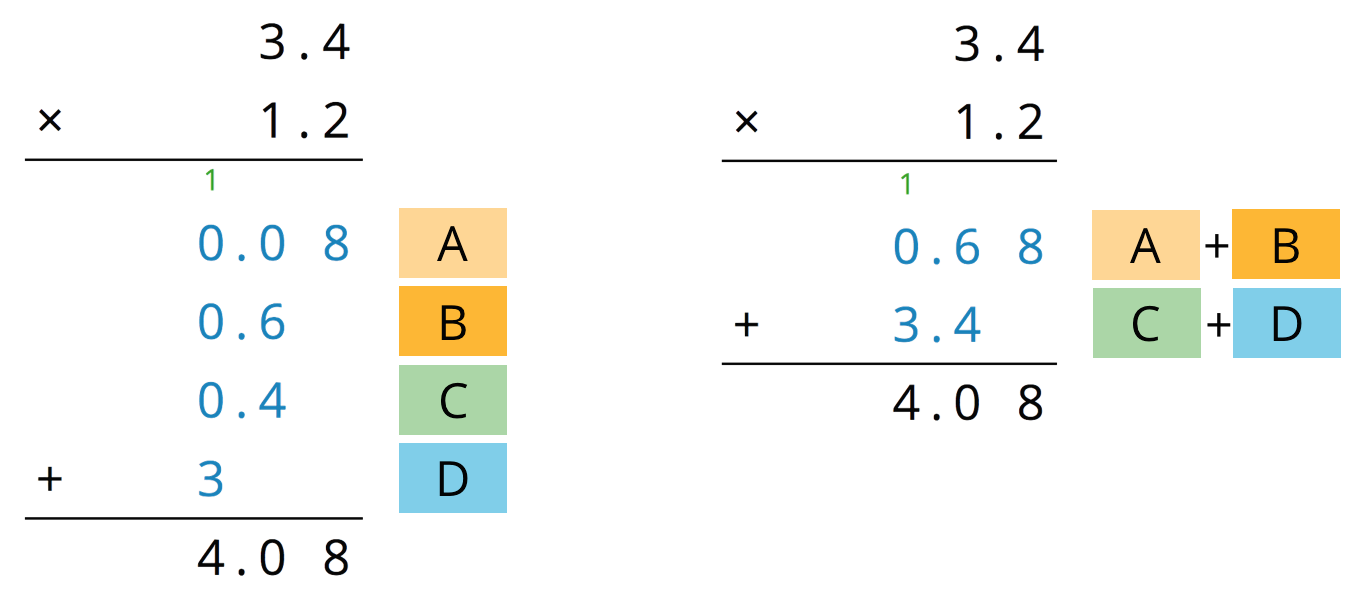
The vertical calculation on the left is an example of the partial products method. It shows the values of each partial product and the letter of the corresponding sub-rectangle. Each partial product gives an area:
The calculation on the right shows the values of two products. Each value gives a combined area of two sub-rectangles:
