Lesson 11: Representing Ratios with Tables

Let’s use tables to represent equivalent ratios.

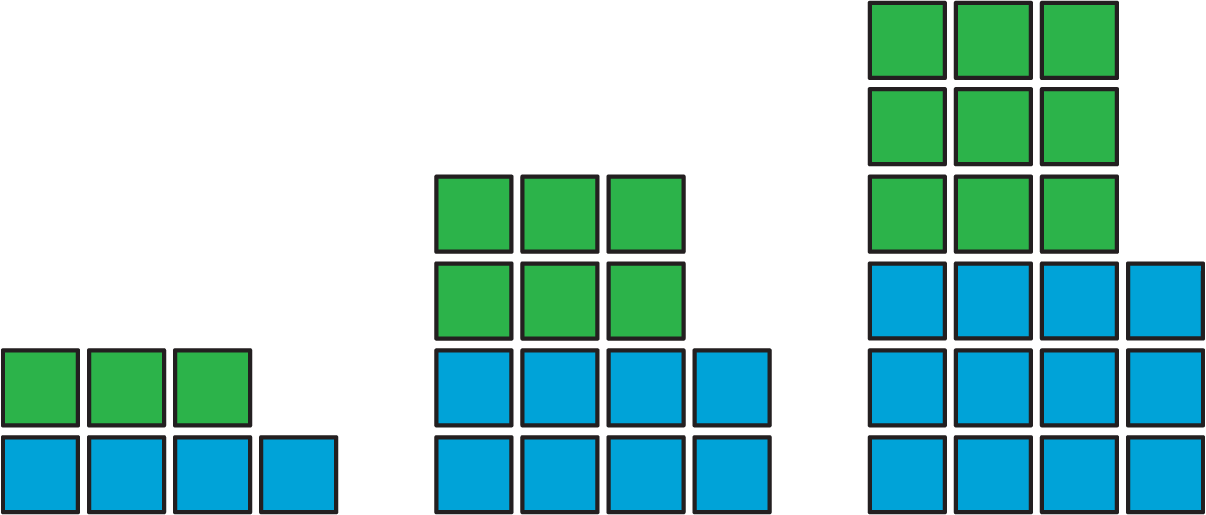
11.1: How Is It Growing?

Look for a pattern in the figures.

  1. How many total tiles will be in:

    1. the 4th figure?
    2. the 5th figure?
    3. the 10th figure?

  2. How do you see it growing?

A growing pattern of tiles arranged in rows. The first figure: row 1, 3 green tiles; row 2, 4 blue tiles. The second figure: row 1, 3 green tiles; row 2, 3 green tiles; row 3, 4 blue tiles; row 4, 4 blue tiles. The third figure: row 1, 3 green tiles; row 2, 3 green tiles; row 3, 3 green tiles; row 4, 4 blue tiles; row 5, 4 blue tiles; row 6, 4 blue tiles.

11.2: A Huge Amount of Sparkling Orange Juice

Noah’s recipe for one batch of sparkling orange juice uses 4 liters of orange juice and 5 liters of soda water.

  1. Use the double number line to show how many liters of each ingredient to use for different-sized batches of sparkling orange juice.

    GeoGebra Applet ycJSQpXT

  2. If someone mixes 36 liters of orange juice and 45 liters of soda water, how many batches would they make?
  3. If someone uses 400 liters of orange juice, how much soda water would they need?
  4. If someone uses 455 liters of soda water, how much orange juice would they need?
  5. Explain the trouble with using a double number line diagram to answer the last two questions.

11.3: Batches of Trail Mix

A recipe for trail mix says: “Mix 7 ounces of almonds with 5 ounces of raisins.” Here is a table that has been started to show how many ounces of almonds and raisins would be in different-sized batches of this trail mix.

  almonds (oz) raisins (oz)
row 1 7 5
row 2 28  
row 3   10
row 4 3.5  
row 5   250
row 6 56  

  1. Complete the table so that ratios represented by each row are equivalent.

  2. What methods did you use to fill in the table?
  3. How do you know that each row shows a ratio that is equivalent
    to $7:5$? Explain your reasoning.
 

Summary

A table is a way to organize information. Each horizontal set of entries is called a row, and each vertical set of entries is called a column. (The table shown has 2 columns and 5 rows.) A table can be used to represent a collection of equivalent ratios.

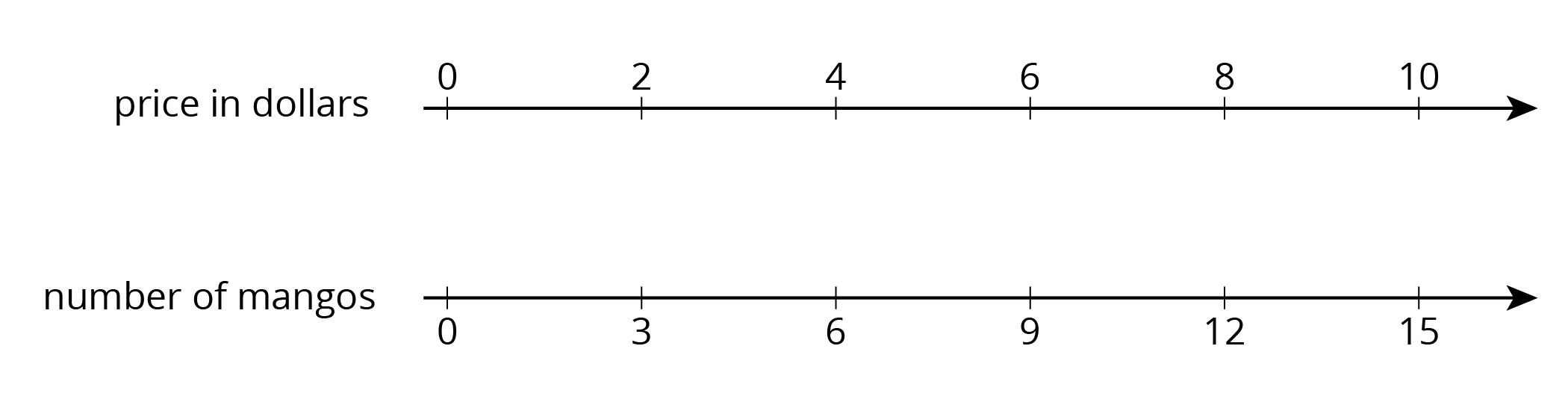
Here is a double number line diagram and a table that both represent the situation: “The price is \$2 for every 3 mangos.”

A double number line with 6 evenly spaced tick marks: For "price in dollars" the numbers 0, 2, 4, 6, 8, and 10 are indicated. For "number of mangos" the numbers 0, 3, 6, 9, 12, and 15 are indicated.
A 2-column table with 5 rows of data. First column is labeled "price in dollars" and the second column is labeled "number of mangos." The data is as follows: Row 1: 2, 3 Row 2: 4, 6 Row 3: 6, 9 Row 4: 8, 12 Row 5: 10, 15
 

Practice Problems ▶

Glossary

table

table

A table is a way to organize information. Each rectangle in the table is called a cell. Each horizontal set of entries is called a row, and each vertical set of entries is called a column. The first row in a table often contains headers to explain what information is in each column. 

This table shows the tail-lengths of three different pets. It has four rows and two columns.

The first cell in each column tells you what kind of information is in that column.

pet tail length (inches)
dog 22
cat 12
mouse 2