Let’s add and subtract decimals.
Evaluate mentally: $1.009+0.391$
Decide if each equation is true or false. Be prepared to explain your reasoning.
a. $34.56000 = 34.56$
b. $25 = 25.0$
c. $2.405 = 2.45$
Andre and Jada drew base-ten diagrams to represent $0.007 + 0.004$. Andre drew 11 small rectangles. Jada drew only two figures: a square and a small rectangle.
Here are two calculations of $0.2 + 0.05$. Which is correct? Explain why one is correct and the other is incorrect.
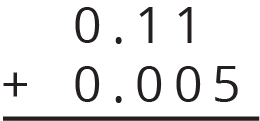
Click on the Move tool when you are done choosing blocks.
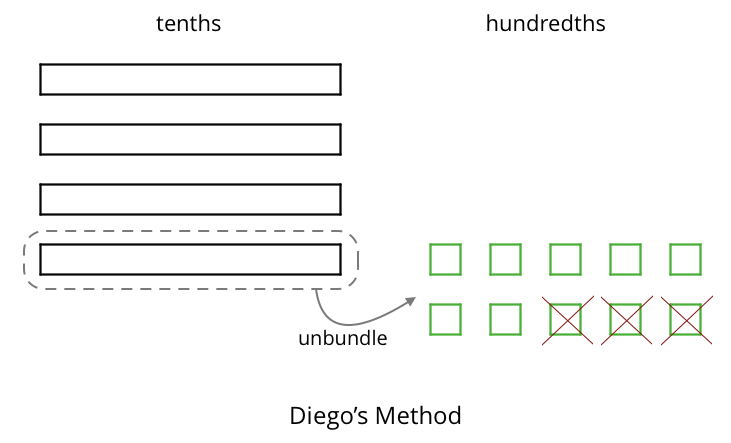
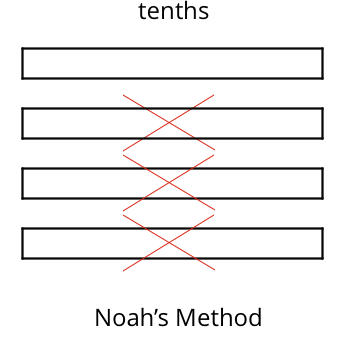
To represent $0.4 - 0.03$, Diego and Noah drew different diagrams. Each rectangle shown here represents 0.1. Each square represents 0.01.
Diego started by drawing 4 rectangles for 0.4. He then replaced 1 rectangle with 10 squares and crossed out 3 squares for the subtraction of 0.03, leaving 3 rectangles and 7 squares in his drawing.
Noah started by drawing 4 rectangles for 0.4. He then crossed out 3 of them to represent the subtraction, leaving 1 rectangle in his drawing.
Do you agree that either diagram correctly represents $0.4 - 0.03$? Discuss your reasoning with a partner.
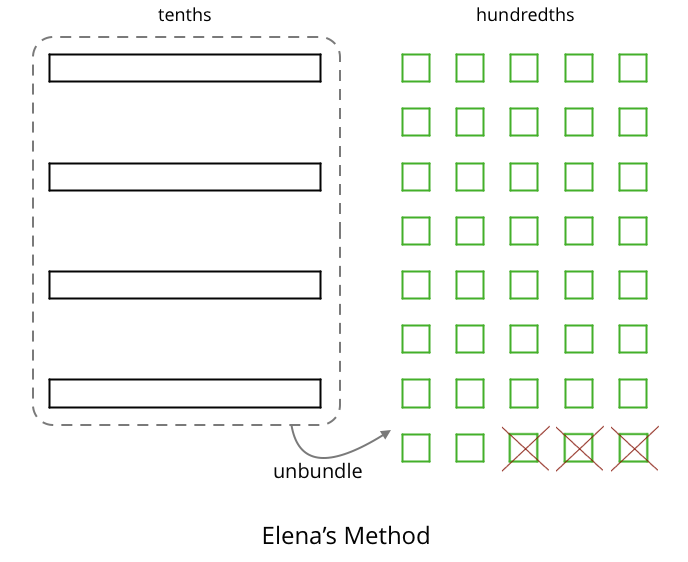
To represent $0.4 - 0.03$, Elena drew another diagram. She also started by drawing 4 rectangles. She then replaced all 4 rectangles with 40 squares and crossed out 3 squares for the subtraction of 0.03, leaving 37 squares in her drawing. Is her diagram correct? Discuss your reasoning with a partner.
Be prepared to explain your reasoning.
Click on the Move tool when you are done choosing blocks.
Subtract by deleting with the delete tool, not crossing out.
A distant, magical land uses jewels for their bartering system. The jewels are valued and ranked in order of their rarity. Each jewel is worth 3 times the jewel immediately below it in the ranking. The ranking is red, orange, yellow, green, blue, indigo, and violet. So a red jewel is worth 3 orange jewels, a green jewel is worth 3 blue jewels, and so on.
At the Auld Shoppe, a shopper buys items that are worth 2 yellow jewels, 2 green jewels, 2 blue jewels, and 1 indigo jewel. If they came into the store with 1 red jewel, 1 yellow jewel, 2 green jewels, 1 blue jewel, and 2 violet jewels, what jewels do they leave with? Assume the shopkeeper gives them their change using as few jewels as possible.
Base-ten diagrams can help us understand subtraction as well as addition. Suppose we are finding $0.023 - 0.007$. Here is a diagram showing 0.023, or 2 hundredths and 3 thousandths.
Subtracting 7 thousandths means removing 7 small squares, but we do not have enough to remove. Because 1 hundredth is equal to 10 thousandths, we can “unbundle” (or decompose) one of the hundredths (1 rectangle) into 10 thousandths (10 small squares).
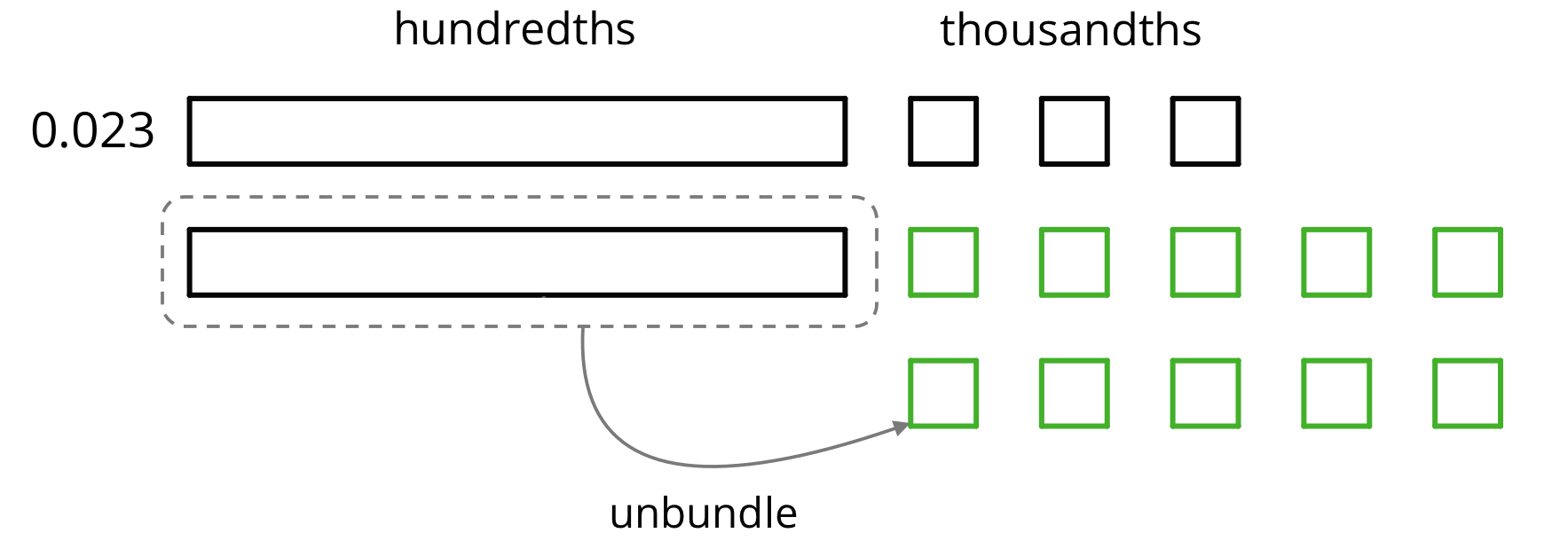
We now have 1 hundredth and 13 thousandths, from which we can remove 7 thousandths.
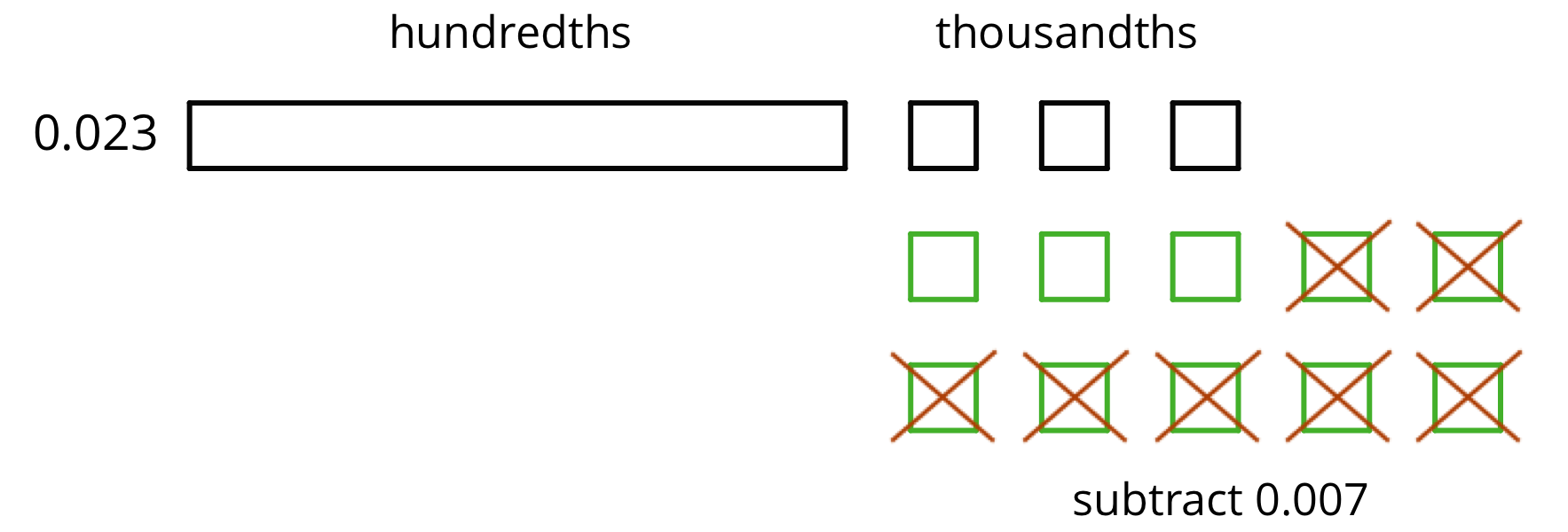
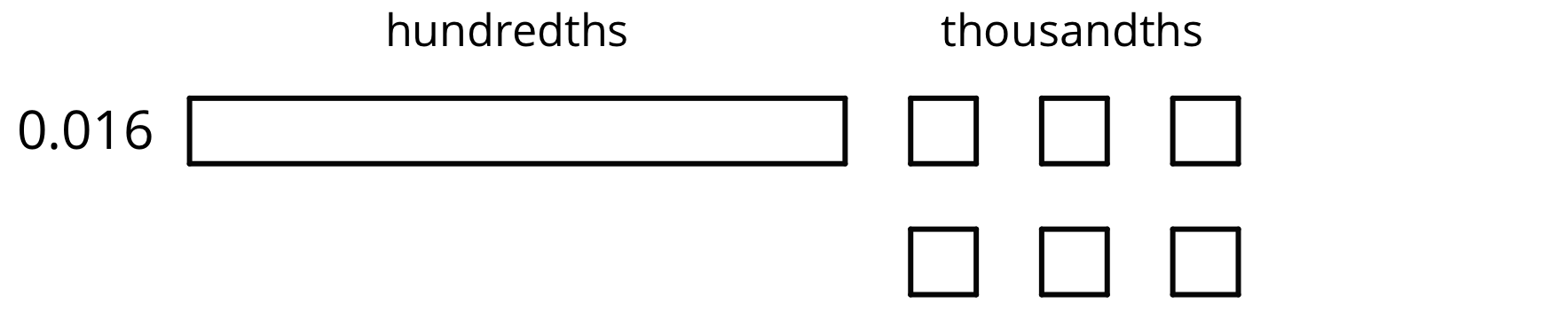
We have 1 hundredth and 6 thousandths remaining, so $0.023 - 0.007 = 0.016$.
Here is a vertical calculation of $0.023 - 0.007$.
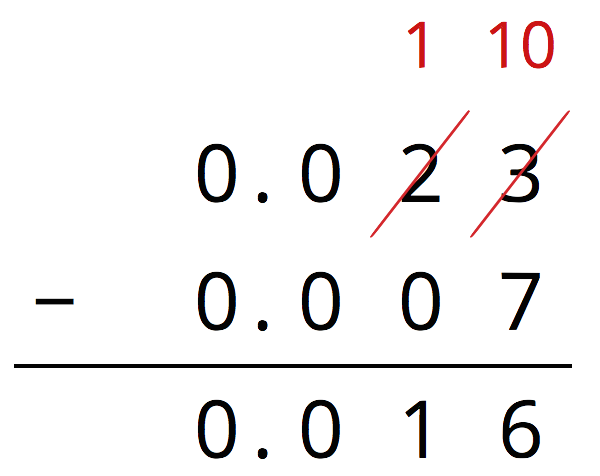
In both calculations, notice that a hundredth is unbundled (or decomposed) into 10 thousandths in order to subtract 7 thousandths.
