Let’s use ratios to compare situations.
Mai and Jada each ran on a treadmill. The treadmill display shows the distance, in miles, each person ran and the amount of time it took them, in minutes and seconds.
Here is Mai’s treadmill display:
Here is Jada’s treadmill display:
Diego paid \$47 for 3 tickets to a concert. Andre paid \$141 for 9 tickets to a concert. Did they pay at the same rate? Explain your reasoning.
Lin makes sparkling orange juice by mixing 3 liters of orange juice with 4 liters of soda water. Noah makes sparkling orange juice by mixing 4 liters of orange juice with 5 liters of soda water. How do the two mixtures compare in taste? Explain your reasoning. If you get stuck, you can draw double number line diagrams to represent each situation.
Sometimes we want to know whether two situations are described by the same rate. To do that, we can write an equivalent ratio for one or both situations so that one part of their ratios has the same value. Then we can compare the other part of the ratios.
For example, do these two paint mixtures make the same shade of orange?
Here is a double number line that represents Kiran's paint mixture. The ratio $9:15$ is equivalent to the ratios $3:5$ and $6:10$.
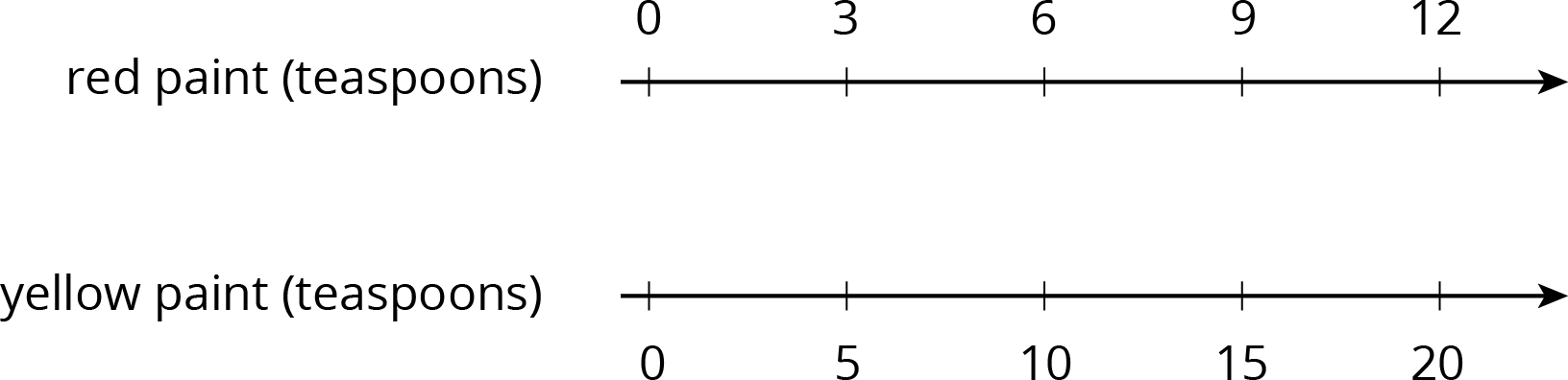
For 10 teaspoons of yellow paint, Kiran would mix in 6 teaspoons of red paint. This is less red paint than Tyler mixes with 10 teaspoons of yellow paint. The ratios $6:10$ and $7:10$ are not equivalent, so these two paint mixtures would not be the same shade of orange.
When we talk about two things happening at the same rate, we mean that the ratios of the quantities in the two situations are equivalent. There is also something specific about the situation that is the same.
In two situations involving ratios of the same two quantities, if the ratio of the quantities in one situation is equivalent to the ratio of the quantities in the other situation then we say the two situations involve the same rate.
