Lesson 14: Using Operations on Decimals to Solve Problems

Let’s solve some problems using decimals.

14.1: Close Estimates

For each expression, choose the best estimate of its value.

  1. $76.2\div15$
    • 0.5
    • 5
    • 50
  1. $56.34\div48$
    • 1
    • 10
    • 100
  1. $124.3\div 20$
    • 6
    • 60
    • 600

14.2: Applying Division with Decimals

Your teacher will assign to you either Problem A or Problem B. Work together as a group to answer the questions. Be prepared to create a visual display to show your reasoning with the class. 

Problem A: A piece of rope is 5.75 meters in length.

  1. If it is cut into 20 equal pieces, how long will each piece be?

  2. If it is cut into 0.05-meter pieces, how many pieces will there be?

Problem B: A tortoise travels 0.945 miles in 3.5 hours.

  1. If it moves at a constant speed, how many miles per hour is it traveling?

  2. At this rate, how long will it take the tortoise to travel 4.86 miles?

Tortoise Copyright Owner: skeeze License: Public Domain Via: Pixabay

14.3: Distance between Hurdles

There are 10 equally-spaced hurdles on a race track. The first hurdle is 13.72 meters from the start line. The final hurdle is 14.02 meters from the finish line. The race track is 110 meters long.

  1. Draw a diagram that shows the hurdles on the race track. Label all known measurements.
August 3rd London Olympics 2012 stadium hurdles Copyright Owner: Steve Flair License: CC BY 2.0 Via: Wikimedia Commons
  1. How far are the hurdles from one another? Explain or show your reasoning.

  2. A professional runner takes 3 strides between each pair of hurdles. The runner leaves the ground 2.2 meters before the hurdle and returns to the ground 1 meter after the hurdle.

    About how long are each of the runner’s strides between the hurdles? Show your reasoning.

14.4: Examining a Tennis Court

Here is a diagram of a tennis court.


 

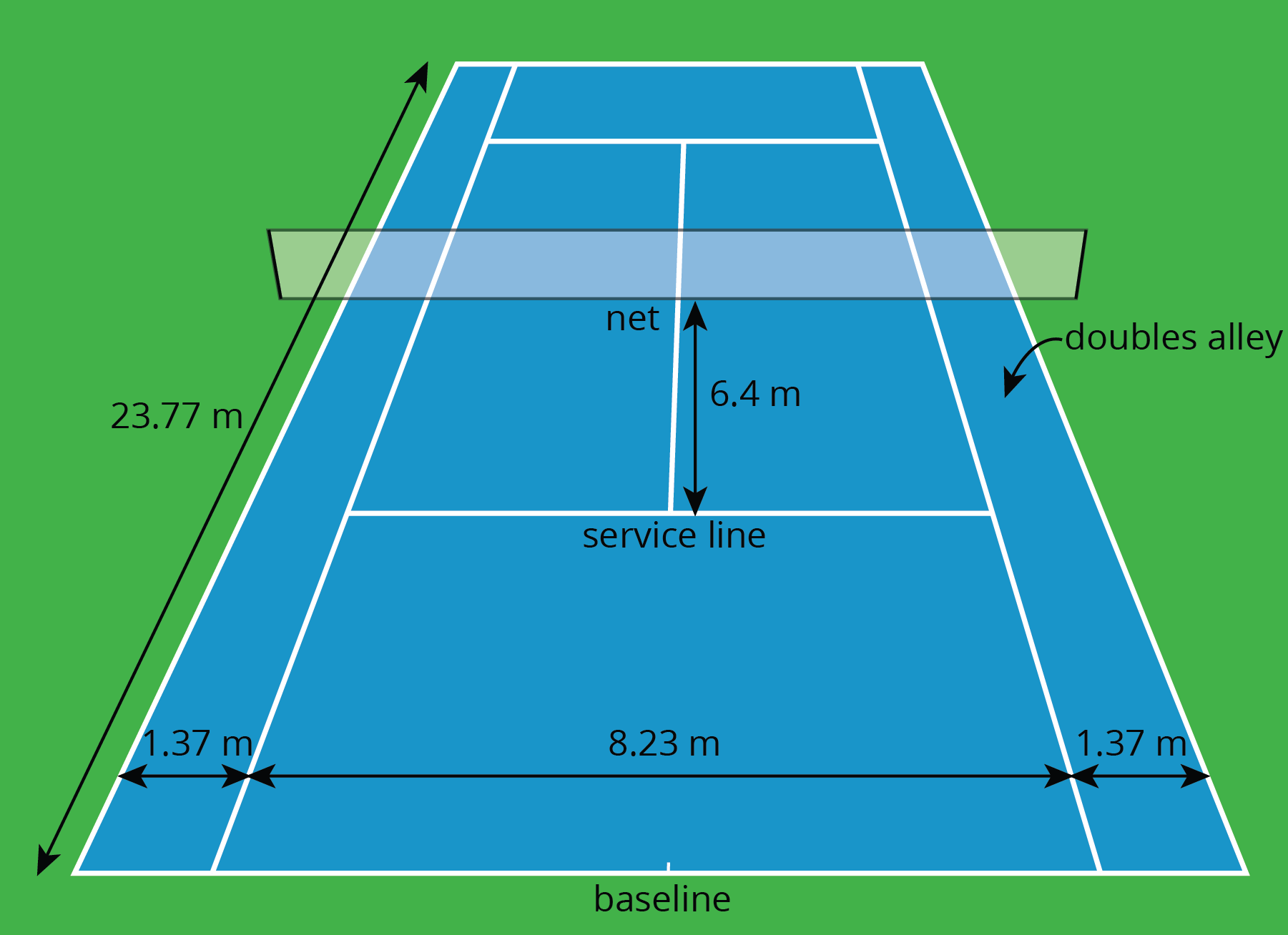
The full tennis court, used for doubles, is a rectangle. All of the angles made by the line segments in the diagram are right angles.

  1. The net partitions the tennis court into two halves. Is each half a square? Explain your reasoning.
  2. Is the service line halfway between the net and the baseline? Explain your reasoning.

  3. Lines painted on a tennis court are 5 cm wide. A painter made markings to show the length and width of the court, then painted the lines to the outside of the markings.

    1. Did the painter’s mistake increase or decrease the overall size of the tennis court? Explain how you know.
    2. By how many square meters did the court’s size change? Explain your reasoning.

Summary

Diagrams can help us communicate and model mathematics. A clearly-labeled diagram helps us visualize what is happening in a problem and accurately communicate the information we need.

Sports offer great examples of how diagrams can help us solve problems. For example, to show the placement of the running hurdles in a diagram, we needed to know what the distances 13.72 and 14.02 meters tell us and the number of hurdles to draw. An accurate diagram not only helped us set up and solve the problem correctly, but also helped us see that there are only nine spaces between ten hurdles. 

To communicate information clearly and solve problems correctly, it is also important to be precise in our measurements and calculations, especially when they involve decimals.

In tennis, for example, the length of the court is 23.77 meters. Because the boundary lines on a tennis court have a significant width, we would want to know whether this measurement is taken between the inside of the lines, the center of the lines, or the outside of the lines. Diagrams can help us attend to this detail, as shown here. 

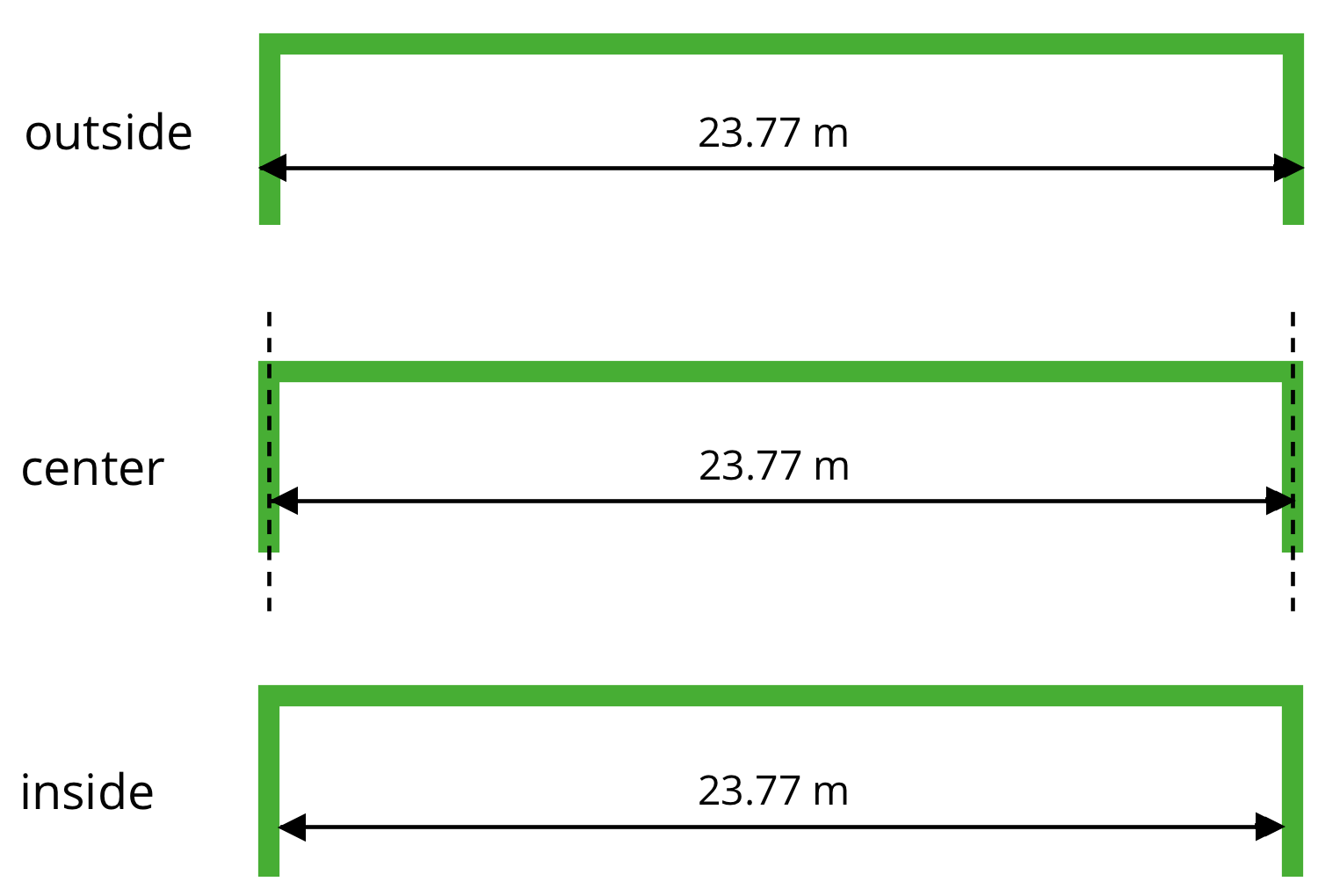
The accuracy of this measurement matters to the tennis players who use the court, so it matters to those who paint the boundaries as well. The tennis players practice their shots to be on or within certain lines. If the tennis court on which they play is not precisely measured, their shots may not land as intended in relation to the boundaries. Court painters usually need to be sure their measurements are accurate to within $\frac{1}{100}$ of a meter or one centimeter.

Practice Problems ▶