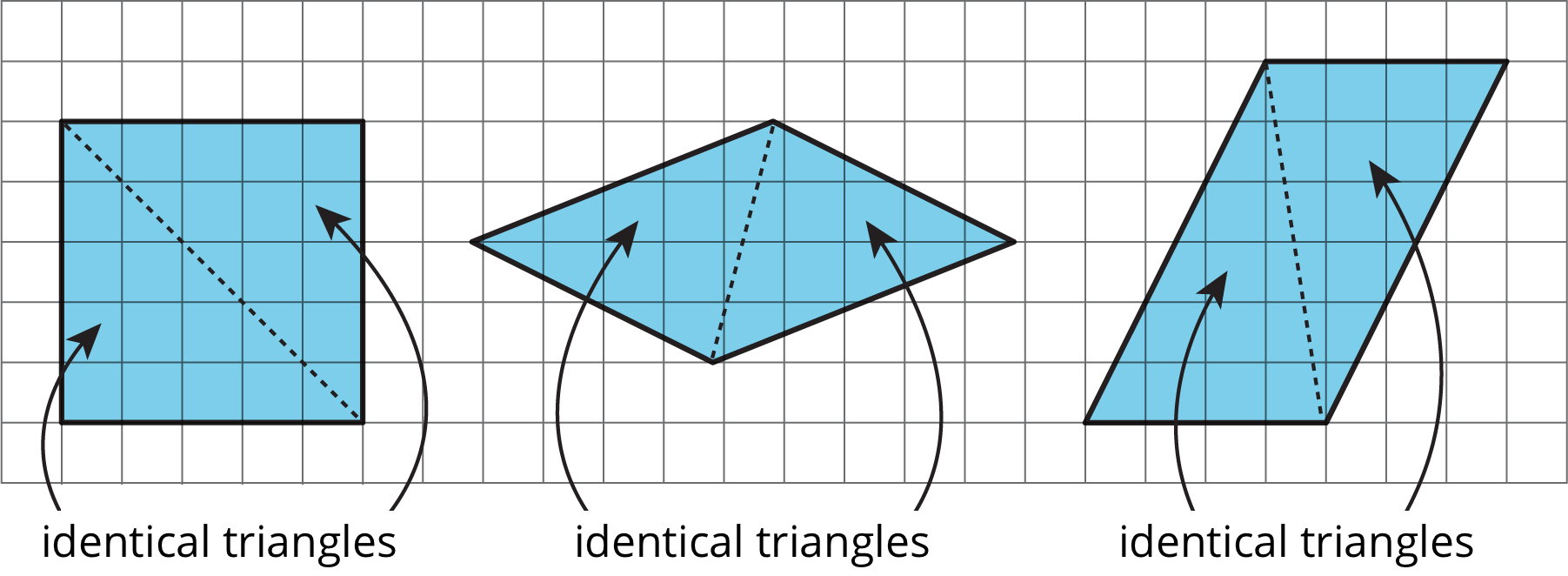
7.1: Same Parallelograms, Different Bases
Here are two copies of a parallelogram. Each copy has one side labeled as the base b and a segment drawn for its corresponding height and labeled h.

- The base of the parallelogram on the left is 2.4 centimeters; its corresponding height is 1 centimeter. Find its area in square centimeters.
- The height of the parallelogram on the right is 2 centimeters. How long is the base of that parallelogram? Explain your reasoning.