Lesson 8: Calculating Products of Decimals

Let’s multiply decimals.

8.1: Number Talk: Twenty Times a Number

Evaluate mentally.

$20 \boldcdot 5$

$20 \boldcdot (0.8)$

$20 \boldcdot (0.04)$

$20 \boldcdot (5.84)$

8.2: Calculating Products of Decimals

  1. A common way to find a product of decimals is to calculate a product of whole numbers, then place the decimal point in the product. 

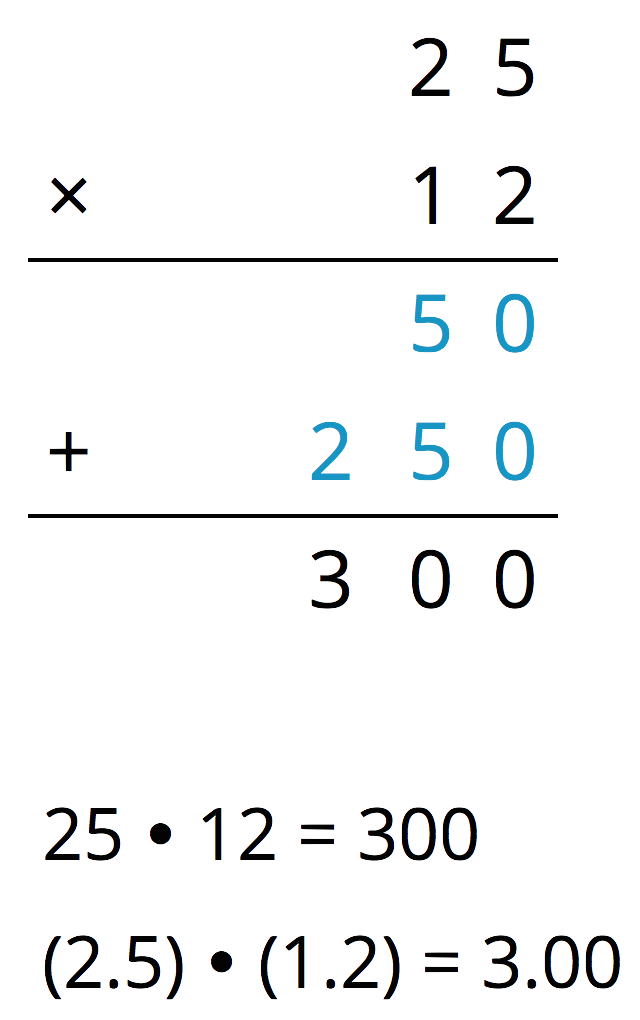
    Here is an example for $(2.5) \boldcdot (1.2)$.

    Use what you know about decimals and place value to explain why the decimal point of the product is placed where it is.

  2. Use the method shown in the first question to calculate each product.

    1. $(4.6) \boldcdot (0.9)$
    1. $(16.5) \boldcdot (0.7)$

  3. Use area diagrams to check your earlier calculations. For each problem:

    • Decompose each number into its base-ten units and write them in the boxes on each side of the rectangle.
    • Write the area of each lettered region in the diagram. Then find the area of the entire rectangle. Show your reasoning.

    1. $(4.6) \boldcdot (0.9)$

    2. $(16.5) \boldcdot (0.7)$

  4. About how many centimeters are in 6.25 inches if 1 inch is about 2.5 centimeters? Show your reasoning.

8.3: Practicing Multiplication of Decimals

  1. Calculate each product. Show your reasoning. If you get stuck, draw an area diagram to help.

    1. $(5.6) \boldcdot (1.8)$
    1. $(0.008) \boldcdot (7.2)$

  2. A rectangular playground is 18.2 meters by 12.75 meters.

    1. Find its area in square meters. Show your reasoning.
    1. If 1 meter is approximately 3.28 feet, what are the approximate side lengths of the playground in feet? Show your reasoning.

Summary

We can use $84\boldcdot 43$ and what we know about place value to find $(8.4) \boldcdot (4.3)$. 

Since 8.4 is 84 tenths and 4.3 is 43 tenths, then: $$(8.4) \boldcdot (4.3) =\frac{84}{10} \boldcdot \frac{43}{10}$$$$(8.4) \boldcdot (4.3) =\frac{84 \boldcdot 43}{100}$$

That means we can compute $84\boldcdot 43$ and then divide by 100 to find $(8.4) \boldcdot (4.3)$.

$$84\boldcdot 43= 3612$$ $$(8.4) \boldcdot (4.3) = 36.12$$

Using fractions such as $\frac{1}{10}$, $\frac{1}{100}$, and $\frac{1}{1,000}$ allows us to find the product of two decimals using the following steps:

  • Write each decimal factor as a product of a whole number and a fraction. 
  • Multiply the whole numbers.
  • Multiply the fractions.
  • Multiply the products of the whole numbers and fractions. 

We know multiplying by fractions such as $\frac{1}{10}$, $\frac{1}{100}$, and $\frac{1}{1,000}$ is the same as dividing by 10, 100, and 1,000, respectively. This means we can move the decimal point in the whole-number product to the left the appropriate number of spaces to correctly place the decimal point. 

Practice Problems ▶