Lesson 6: Using Diagrams to Find the Number of Groups

Let’s draw tape diagrams to think about division with fractions.

We can think of the division expression $10 \div 2\frac12$ as the answer to the question: “How many groups of $2\frac 12$ s are in 10?” Complete the tape diagram to represent the question. Then answer the question.
Complete the tape diagram to represent the question: “How many groups of 2 are in 7?” Then answer the question.

To make sense of the question “How many $\frac 23$ s are in 1?,” Andre wrote equations and drew a tape diagram.

${?} \boldcdot \frac 23 = 1$

$1 \div \frac 23 = {?}$

A tape diagram with three equal parts. The first two parts are shaded and are each labeled one third. Above the tape diagram is a bracket labeled 1, and contains all three parts. Below the diagram there is a bracket labeled "1 group of two thirds," and contains the first two parts.

In an earlier task, we used pattern blocks to help us solve the equation $1 \div \frac 23 = {?}$ . Explain how Andre’s tape diagram can also help us solve the equation.
Write a multiplication equation and a division equation for each of the following questions. Draw a tape diagram to find the solution. Use the grid to help you draw, if needed.
1. How many $\frac 34$ s are in 1?
2. How many $\frac23$ s are in 3?
3. How many $\frac32$ s are in 5?

For each question, draw a diagram to show the relationship of the quantities and to help you answer the question. Then, write a multiplication equation or a division equation for the situation described in the question. Be prepared to share your reasoning.
1. How many $\frac38$ -inch thick books make a stack that is 6 inches tall?
2. How many groups of $\frac12$ pound are in $2\frac 34$ pounds?
Write a question that can be represented by the division equation $5 \div 1\frac12 = {?}$ . Then answer the question. Show your reasoning.

Summary

A baker used 2 kilograms of flour to make several batches of a pastry recipe. The recipe called for $\frac25$ kilogram of flour per batch. How many batches did she make?

We can think of the question as: “How many groups of $\frac25$ kilogram make 2 kilograms?” and represent that question with the equations:

${?} \boldcdot \frac25=2$

$2 \div \frac25 = {?}$

To help us make sense of the question, we can draw a tape diagram. This diagram shows 2 whole kilograms, with each kilogram partitioned into fifths.

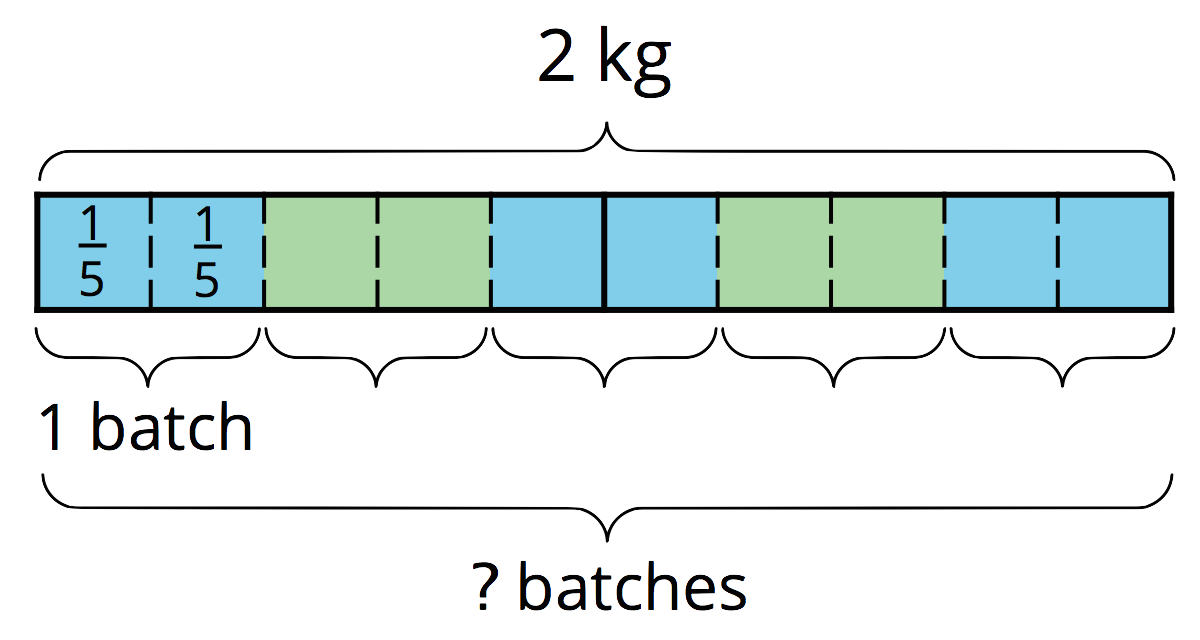
We can see there are 5 groups of $\frac 25$ in 2. Multiplying 5 and $\frac25$ allows us to check this answer: $5 \boldcdot \frac 25 = \frac{10}{5}$ and $\frac {10}{5} = 2$ , so the answer is correct.

Notice the number of groups that result from $2 \div \frac25$ is a whole number. Sometimes the number of groups we find from dividing may not be a whole number. Here is an example:

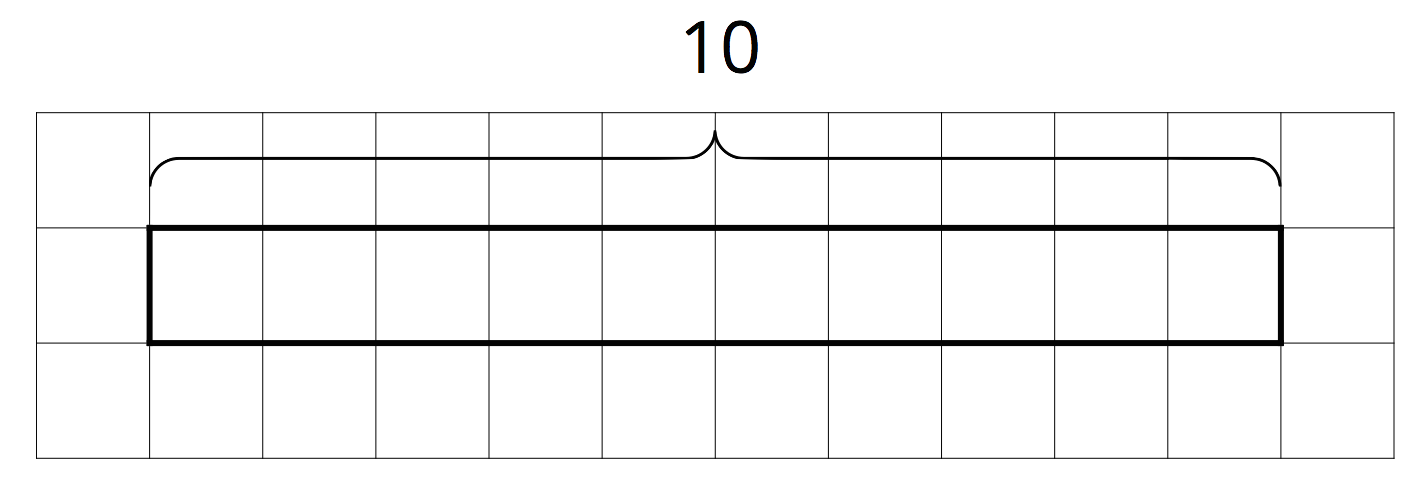
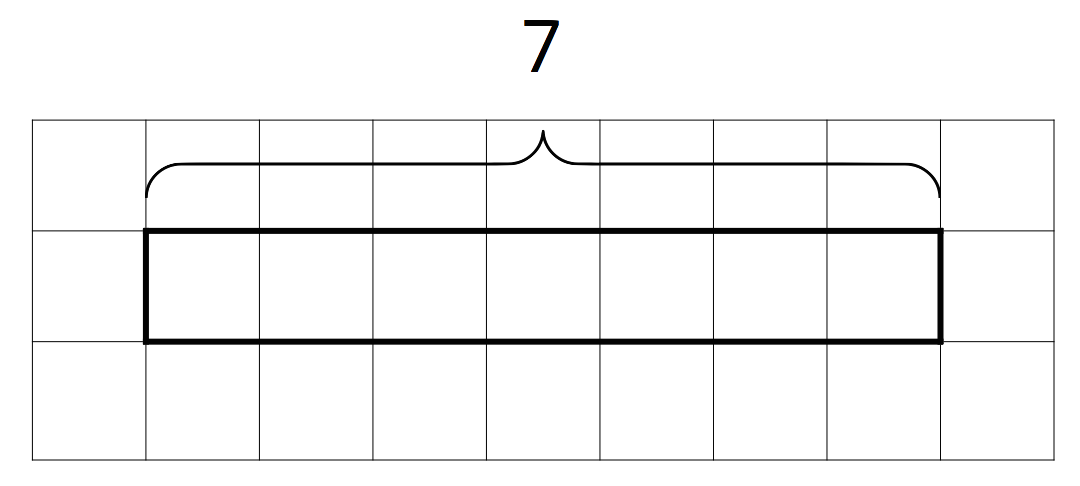
Suppose one serving of rice is $\frac34$ cup. How many servings are there in $3\frac12$ cups?

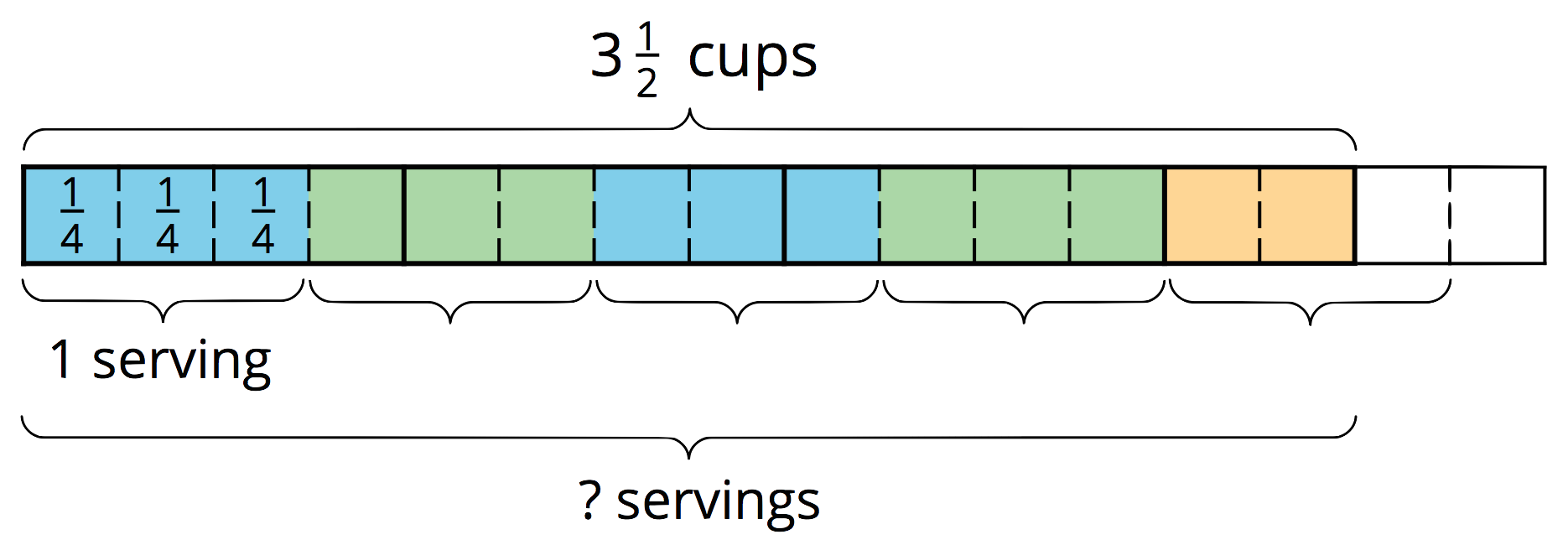
${?}\boldcdot \frac34 = 3\frac12$ $3\frac12 \div \frac34 = {?}$

Looking at the diagram, we can see there are 4 full groups of $\frac 34$ , plus 2 fourths. If 3 fourths make a whole group, then 2 fourths make $\frac 23$ of a group. So the number of servings (the “?” in each equation) is $4\frac23$ . We can check this by multiplying $4\frac23$ and $\frac34$ .

$4\frac23 \boldcdot \frac34 = \frac{14}{3} \boldcdot \frac34$ , and $\frac{14}{3} \boldcdot \frac34 = \frac{14}{4}$ , which is indeed equivalent to $3\frac12$ .

Lesson 6: Using Diagrams to Find the Number of Groups

6.1: How Many of These in That?

6.2: Representing Groups of Fractions with Tape Diagrams

6.3: Finding Number of Groups

Summary

Practice Problems ▶