Lesson 11: Using an Algorithm to Divide Fractions

Let’s divide fractions using the rule we learned.

11.1: Multiplying Fractions

Evaluate each expression. 

  1. $\frac 23 \boldcdot 27$
  2. $\frac 12 \boldcdot \frac 23$
  1. $\frac 29 \boldcdot \frac 35$
  2. $\frac {27}{100} \boldcdot \frac {200}{9}$
  1. $\left( 1\frac 34 \right) \boldcdot \frac 57$

11.2: Dividing a Fraction by a Fraction

Work with a partner. One person should work on the questions labeled “Partner A,” and the other should work on those labeled “Partner B.”

  1. Partner A.

    Find the value of each expression, and answer the question by completing the diagram that has been started for you. Show your reasoning.

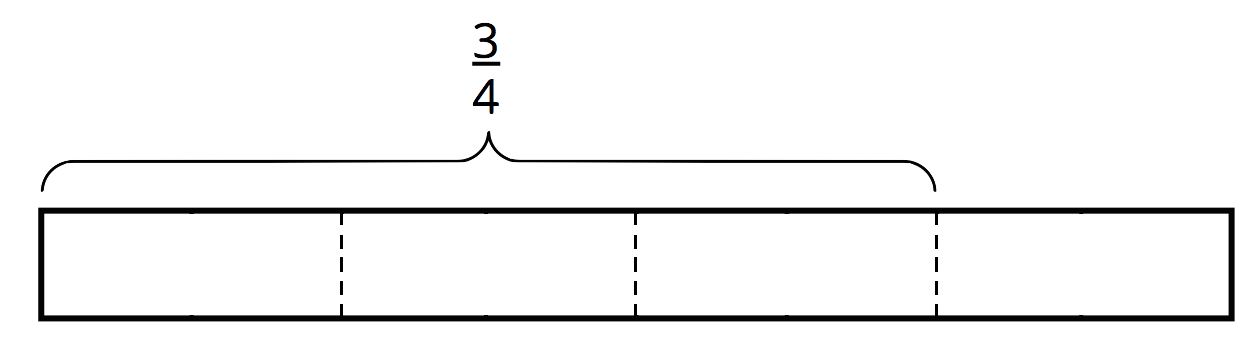
    1. $\frac 34 \div \frac 18$

      How many $\frac 18$s in $\frac 34$?

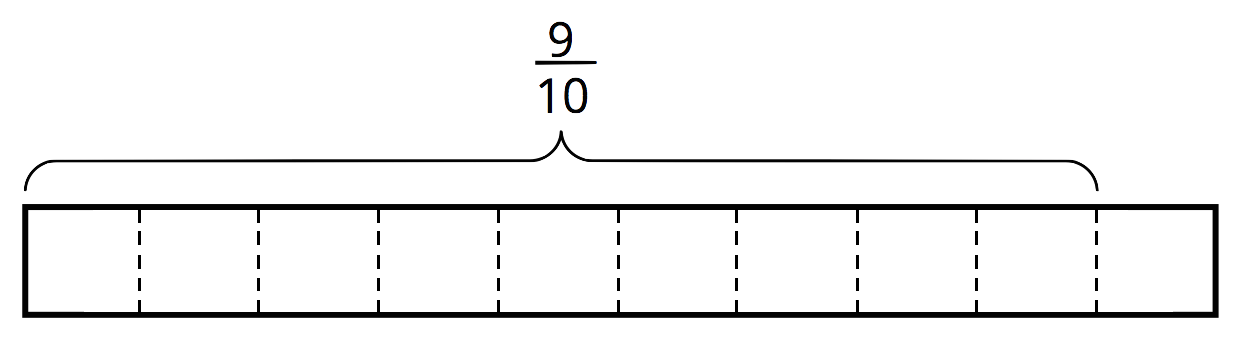
    1. $\frac {9}{10} \div \frac 35$

      How many $\frac 35$s in $\frac{9}{10}$?

    A tape diagram of 10 equal parts. From the beginning of the diagram to the end of the ninth part of the diagram a brace is drawn and labeled nine tenths.

    Use the applet to confirm your answers and explore your own examples.

    GeoGebra Applet wJWDB3pw

  2. Partner B.

    Elena said: “If you want to divide 4 by $\frac 25$, you can multiply 4 by 5, then divide it by 2 or multiply it by $\frac 12$.”

    Find the value of each expression using the strategy that Elena described.

    1. $\frac 34 \div \frac 18$

    1. $\frac{9}{10} \div \frac35$

    Pause here for a discussion with your partner.

  3. Complete this statement based on your observations:

    To divide a number $n$ by a fraction $\frac {a}{b}$, we can multiply $n$ by ________ and then divide the product by ________.

  4. Select all equations that represent the statement you completed.

    1. $n \div \frac {a}{b} = n \boldcdot b \div a$
    2. $n \div \frac {a}{b}= n \boldcdot a \div b$
    1. $n \div \frac {a}{b} = n \boldcdot \frac {a}{b}$
    2. $n \div \frac {a}{b} = n \boldcdot \frac {b}{a}$

11.3: Using an Algorithm to Divide Fractions

  1. Calculate each quotient using your preferred strategy. Show your work and be prepared to explain your strategy.

    1. $\frac 89 \div 4$

    2. $\frac 34 \div \frac 12$
    3. $3 \frac13 \div \frac29$

    1. $\frac92 \div \frac 38$

    2. $6 \frac 25 \div 3$
  2. After biking $5 \frac 12$ miles, Jada has traveled $\frac 23$ of the length of her trip. How long (in miles) is the entire length of her trip? Write an equation to represent the situation, and find the answer using your preferred strategy.

Summary

The division $a \div \frac34 = {?}$ is equivalent to $\frac 34 \boldcdot {?} = a$, so we can think of it as meaning “$\frac34$ of what number is $a$?” and represent it with a diagram as shown. The length of the entire diagram represents the unknown number.

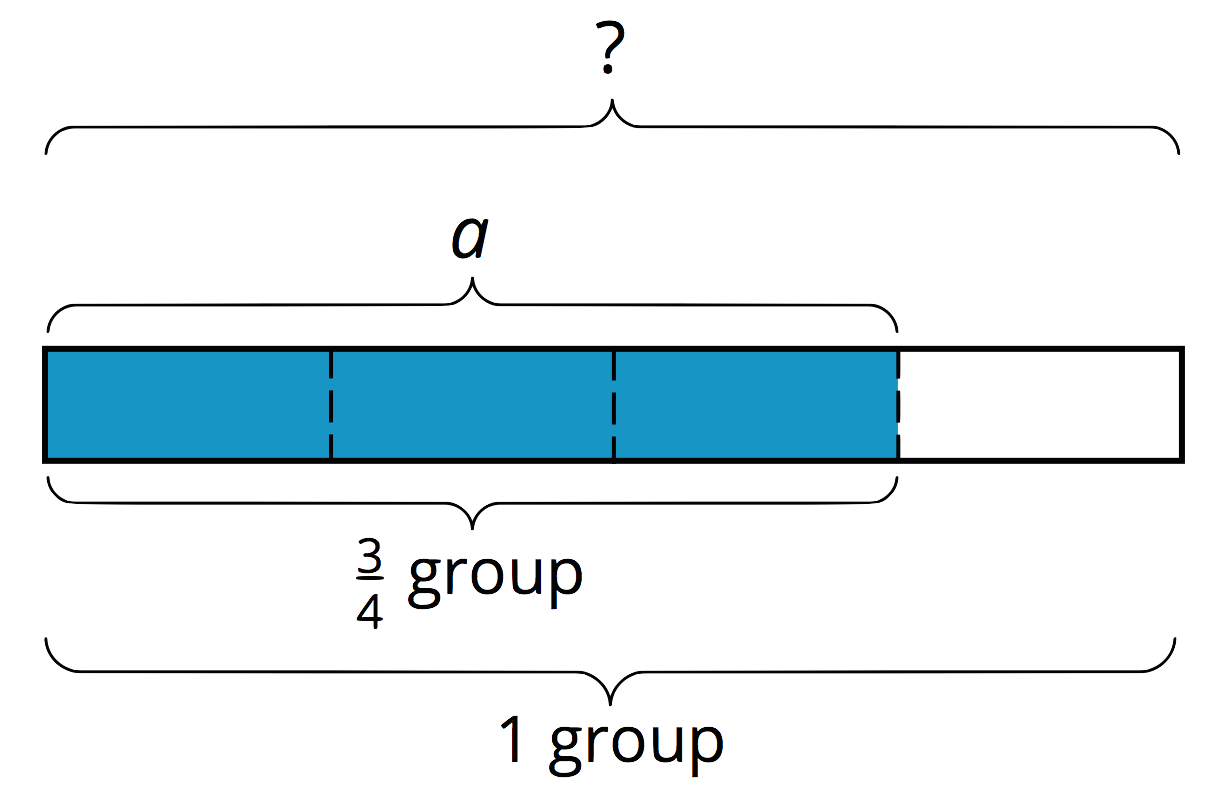
If $\frac34$ of a number is $a$, then to find the number, we can first divide $a$ by 3 to find $\frac14$ of the number. Then we multiply the result by 4 to find the number.

The steps above can be written as: $a \div 3 \boldcdot 4$. Dividing by 3 is the same as multiplying by $\frac13$, so we can also write the steps as: $a \boldcdot \frac13 \boldcdot 4$.

In other words: $a \div 3 \boldcdot 4= a \boldcdot \frac13 \boldcdot 4$. And $a \boldcdot \frac13 \boldcdot 4 = a \boldcdot \frac43$, so we can say that: $$a \div \frac34= a \boldcdot \frac43$$

In general, dividing a number by a fraction $\frac{c}{d}$ is the same as multiplying the number by $\frac{d}{c}$, which is the reciprocal of the fraction.

Practice Problems ▶