5.1: Dots and Half Dots
Dot Pattern 1:
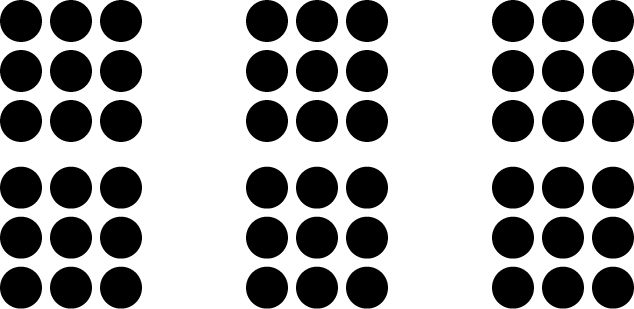
Dot Pattern 2:

Let’s investigate equivalent ratios some more.
Dot Pattern 1:

Dot Pattern 2:

Here is a recipe for tuna casserole.
Combine the pasta, tuna, soup, and half of the cheese. Transfer into a 9 inch by 18 inch baking dish. Put the remaining cheese on top. Bake 30 minutes at 350 degrees. During the last 5 minutes, add the French fried onions. Let sit for 10 minutes before serving.
How many batches of casserole would you make if you used the following amounts of ingredients?
The recipe says to use a 9 inch by 18 inch baking dish. Determine the length and width of a baking dish with the same height that could hold:
The ratios 5:3 and 10:6 are equivalent ratios.
Write a definition of equivalent ratios.
Pause here so your teacher can review your work and assign you a ratio to use for your visual display.
Create a visual display that includes:
All ratios that are equivalent to a:b can be made by multiplying both a and b by the same number.
For example, the ratio 18:12 is equivalent to 9:6 because both 9 and 6 are multiplied by the same number: 2.

3:2 is also equivalent to 9:6, because both 9 and 6 are multiplied by the same number: \frac13.

Is 18:15 equivalent to 9:6?
No, because 18 is 9 \boldcdot 2, but 15 is not 6 \boldcdot 2.

Two ratios a:b and c:d are equivalent ratios if there is a number s that you can multiply both a and b by to get c and d (respectively). In other words, a\boldcdot s = c and b \boldcdot s = d.