6.1: Missing Dots
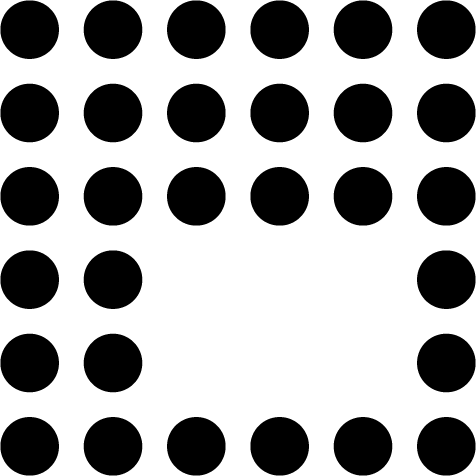
How many dots are in the image?
How do you see them?
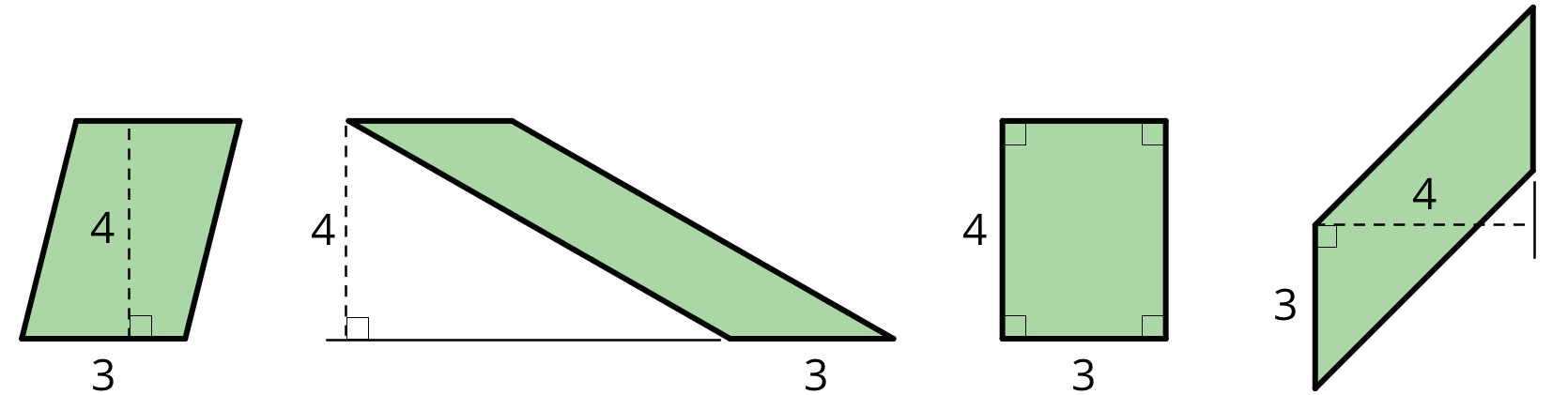
Let's practice finding the area of parallelograms.

How many dots are in the image?
How do you see them?
Here is a parallelogram composed of smaller parallelograms. The shaded region is composed of four identical parallelograms. All lengths are in inches.
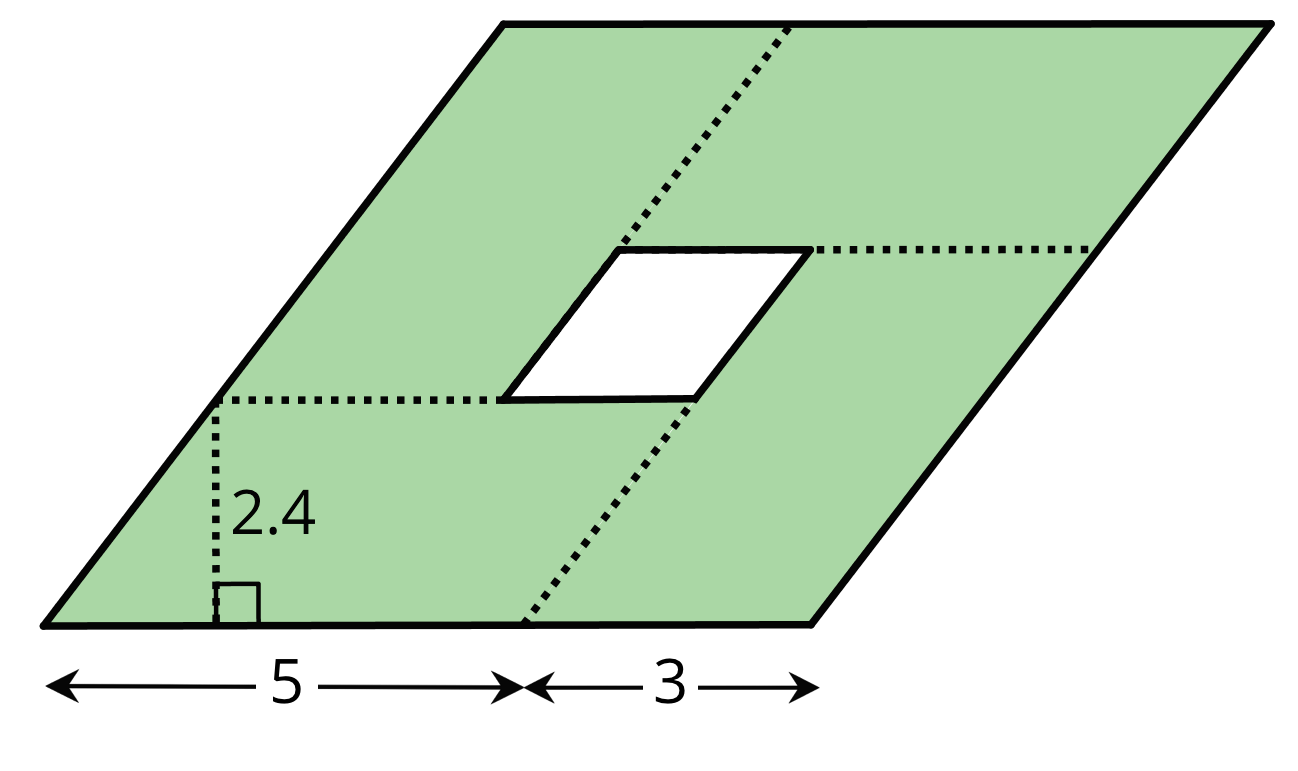
What is the area of the unshaded parallelogram in the middle? Explain or show your reasoning.
Any pair of base and corresponding height can help us find the area of a parallelogram, but some base-height pairs are more easily identified than others.
When a parallelogram is drawn on a grid and has horizontal sides, we can use a horizontal side as the base. When it has vertical sides, we can use a vertical side as the base. The grid can help us find (or estimate) the lengths of the base and of the corresponding height.
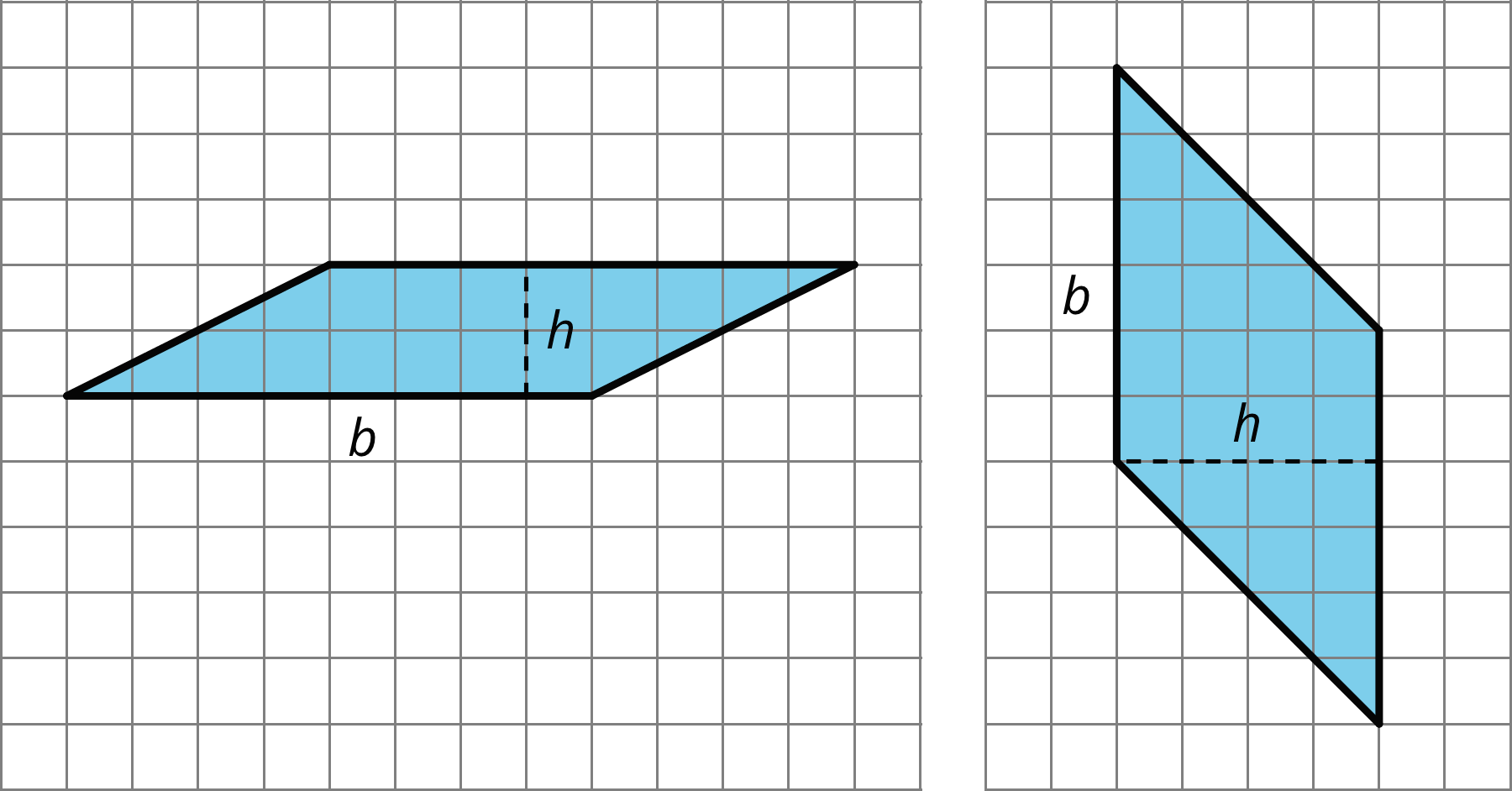
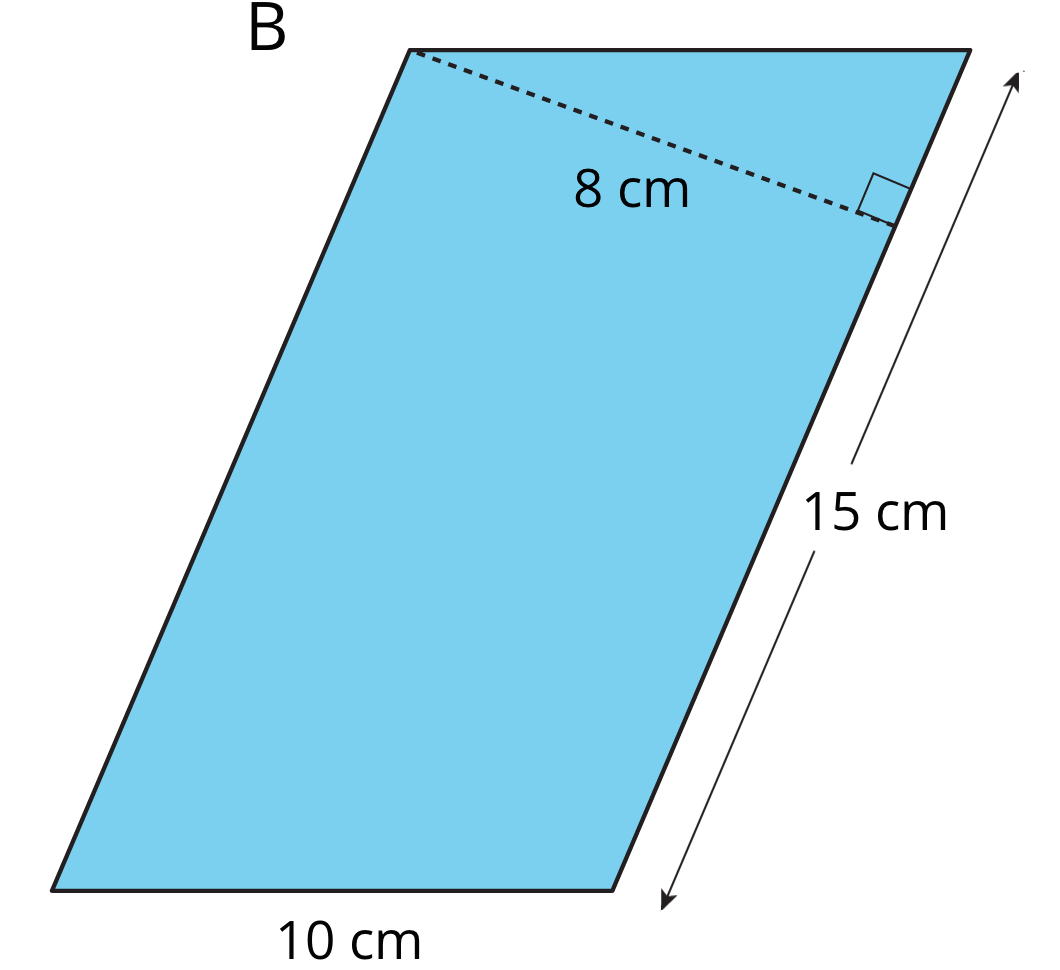
When a parallelogram is not drawn on a grid, we can still find its area if a base and a corresponding height are known.
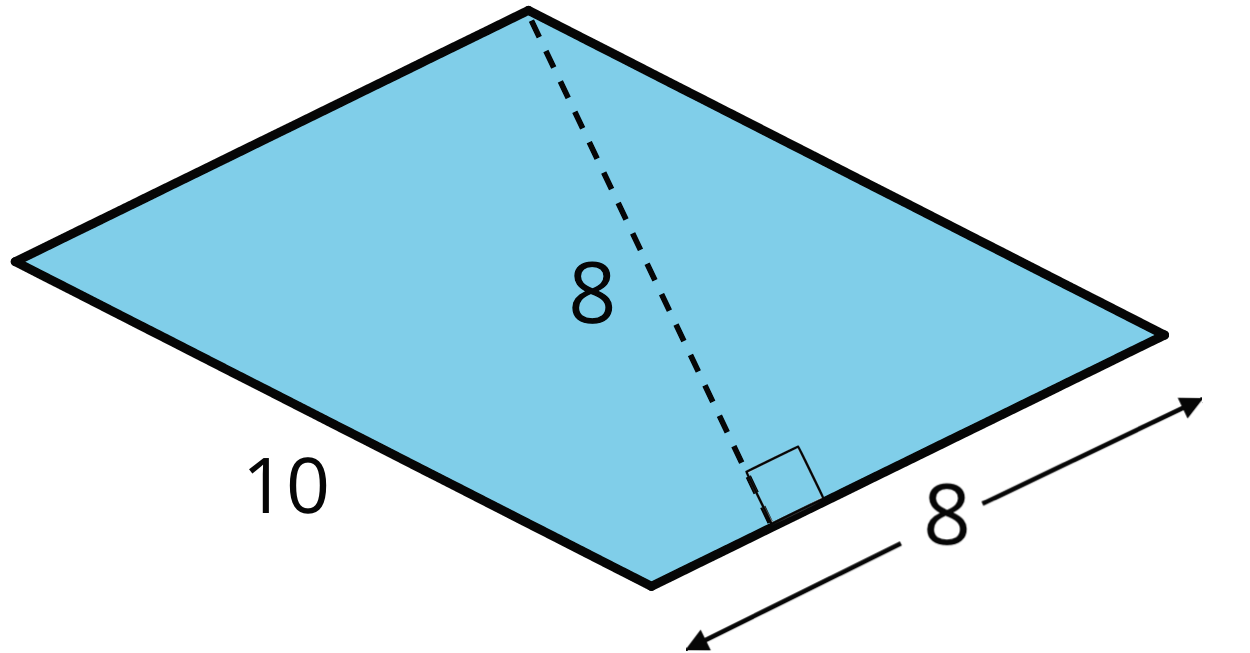
In this parallelogram, the corresponding height for the side that is 10 units long is not given, but the height for the side that is 8 units long is given. This base-height pair can help us find the area.
Regardless of their shape, parallelograms that have the same base and the same height will have the same area; the product of the base and height will be equal. Here are some parallelograms with the same pair of base-height measurements.