3.1: Flower Pattern
This flower is made up of yellow hexagons, red trapezoids, and green triangles.
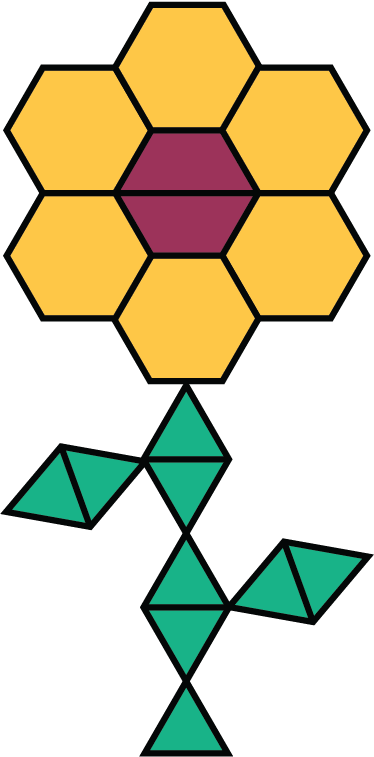
- Write sentences to describe the ratios of the shapes that make up this pattern.
- How many of each shape would be in two copies of this flower pattern?
Let’s explore how ratios affect the way a recipe tastes.
This flower is made up of yellow hexagons, red trapezoids, and green triangles.

Here are diagrams representing three mixtures of powdered drink mix and water:
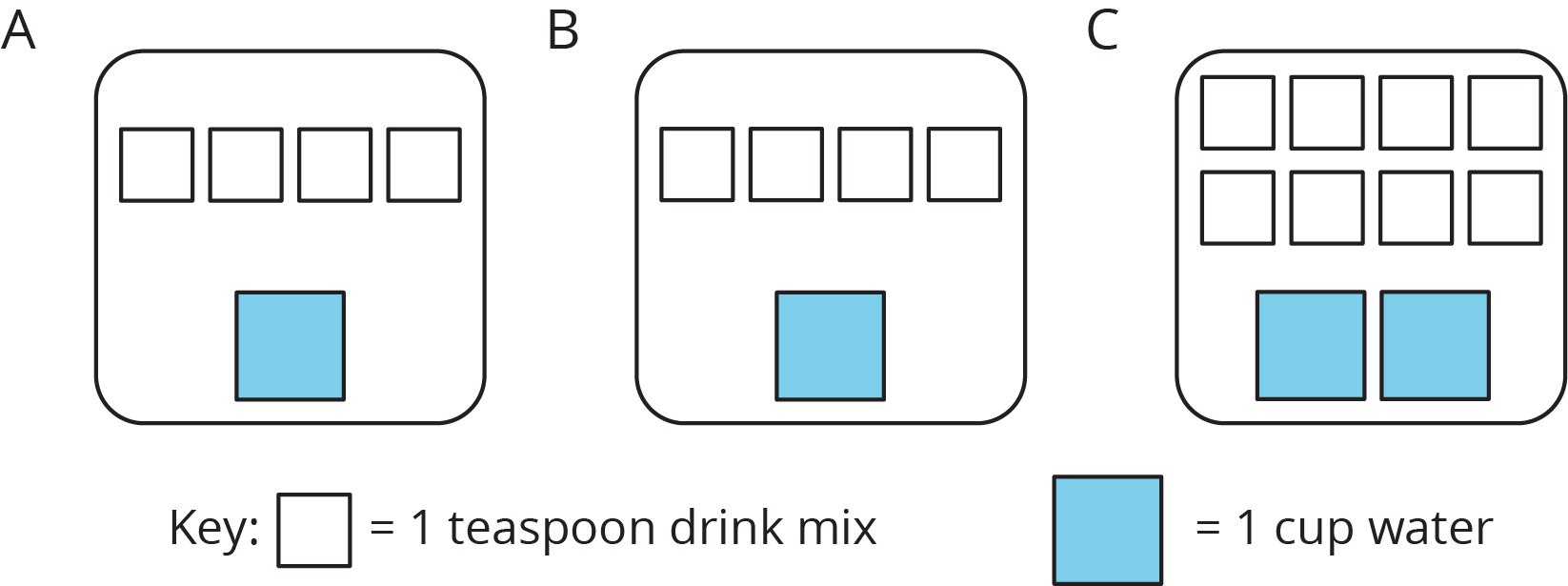
How would the taste of Mixture A compare to the taste of Mixture B?
Use the diagrams to complete each statement:
Mixture B uses ______ cups of water and ______ teaspoons of drink mix. The ratio of cups of water to teaspoons of drink mix in Mixture B is ________.
Mixture C uses ______ cups of water and ______ teaspoons of drink mix. The ratio of cups of water to teaspoons of drink mix in Mixture C is ________.
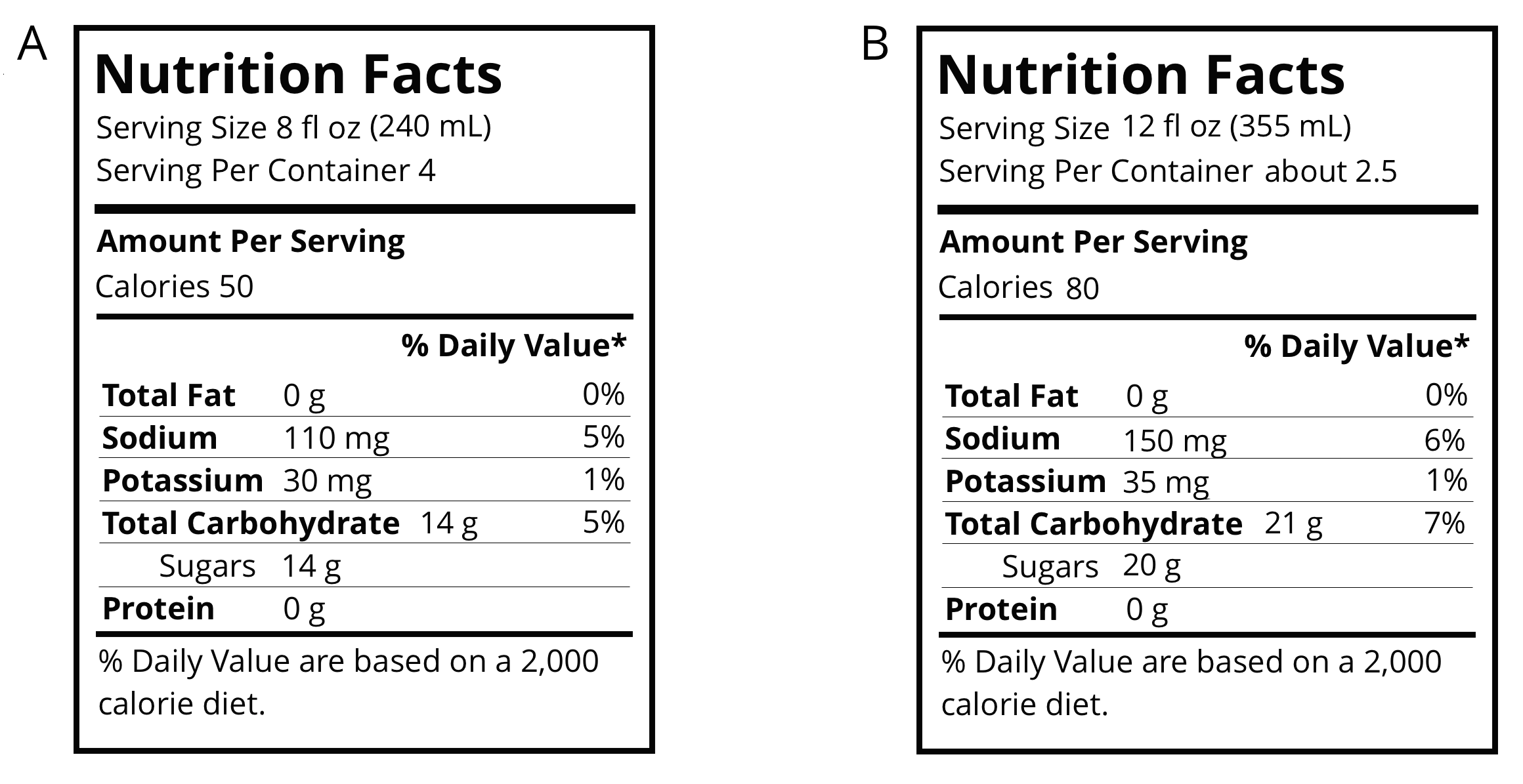
Sports drinks use sodium (better known as salt) to help people replenish electrolytes. Here are the nutrition labels of two sports drinks.
A recipe for one batch of cookies calls for 5 cups of flour and 2 teaspoons of vanilla.
Whether the ratio of cups of flour to teaspoons of vanilla is 5:2, 10:4, or 15:6, the recipes would make cookies that taste the same. We call these equivalent ratios.
A recipe for fizzy juice says, “Mix 5 cups of cranberry juice with 2 cups of soda water.”
To double this recipe, we would use 10 cups of cranberry juice with 4 cups of soda water. To triple this recipe, we would use 15 cups of cranberry juice with 6 cups of soda water.
This diagram shows a single batch of the recipe, a double batch, and a triple batch:
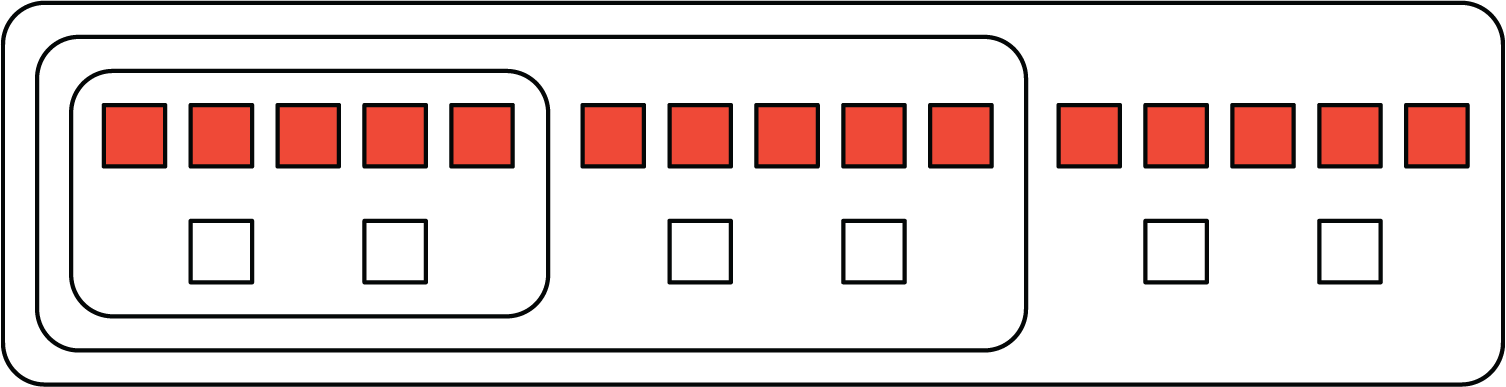
We say that the ratios 5 : 2, 10 : 4, and 15 : 6 are equivalent. Even though the amounts of each ingredient within a single, double, or triple batch are not the same, they would make fizzy juice that tastes the same.
Two ratios a:b and c:d are equivalent ratios if there is a number s that you can multiply both a and b by to get c and d (respectively). In other words, a\boldcdot s = c and b \boldcdot s = d.