Lesson 11: Percentages and Double Number Lines

Let’s use double number lines to represent percentages.

11.1: Fundraising Goal

Each of three friends—Lin, Jada, and Andre—had the goal of raising \$40. How much money did each person raise? Be prepared to explain your reasoning.

  1. Lin raised 100% of her goal.

  2. Jada raised 50% of her goal.

  3. Andre raised 150% of his goal.

11.2: Three-Day Biking Trip

Elena biked 8 miles on Saturday. Use the double number line to answer the questions. Be prepared to explain your reasoning.

A double number line for “distance in miles” with 7 evenly spaced tick marks. The top number line has the number 0 on the first tick mark and the remaining tick marks are blank. The bottom number line starting with the first tick mark, 0 percent, 25 percent, 50 percent, 75 percent, 100 percent, 125 percent and 150 percent are labeled

  1. What is 100% of her Saturday distance?

  2. On Sunday, she biked 75% of her Saturday distance. How far was that?

  3. On Monday, she biked 125% of her Saturday distance. How far was that?

 

11.3: Puppies Grow Up

  1. Jada has a new puppy that weighs 9 pounds. The vet says that the puppy is now at about 20% of its adult weight. What will be the adult weight of the puppy?

    A double number line for “weight in pounds” with 2 tick marks. The top number line has the number 0 on the first tick mark and 9 on the second. The bottom number line has 0 percent on the first tick mark and 20 percent on the second.

  2. Andre also has a puppy that weighs 9 pounds. The vet says that this puppy is now at about 30% of its adult weight. What will be the adult weight of Andre’s puppy?

    A double number line for “weight in pounds” with 2 tick marks. The top number line has the number 0 on the first tick mark and 9 on the second. The bottom number line has 0 percent on the first tick mark and 30 percent on the second.
  3. What is the same about Jada and Andre’s puppies? What is different?

Summary

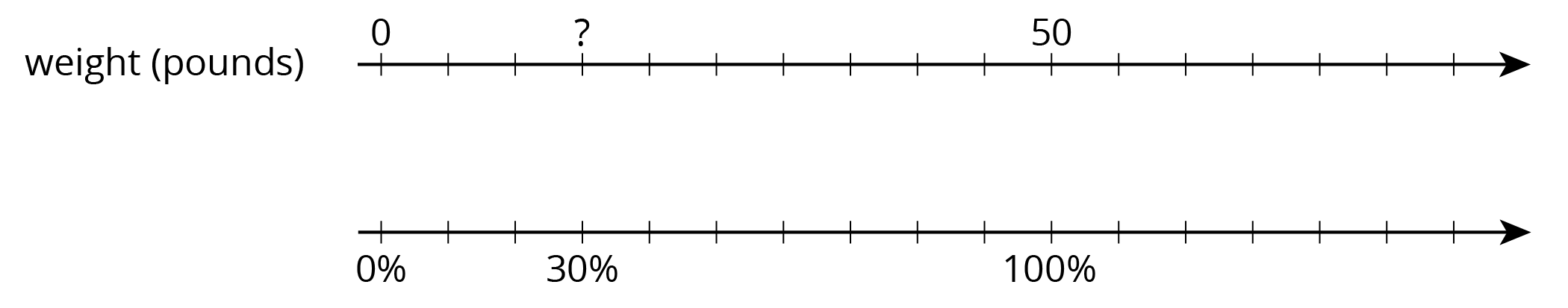
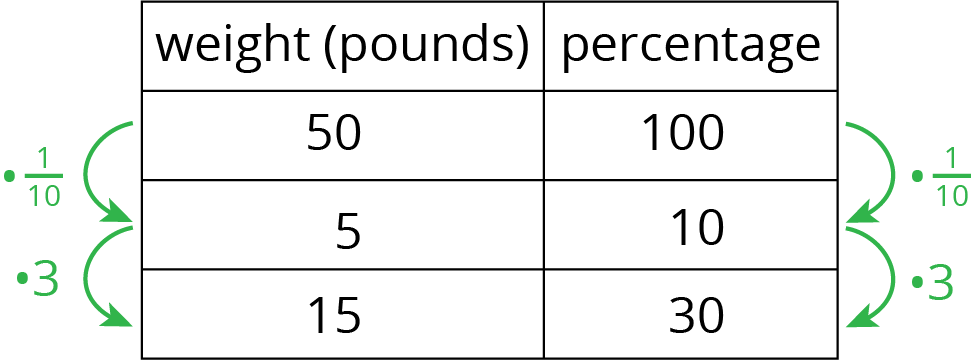
We can use a double number line to solve problems about percentages. For example, what is 30% of 50 pounds? We can draw a double number line like this:

“”

We divide the distance between 0% and 100% and that between 0 and 50 pounds into ten equal parts. We label the tick marks on the top line by counting by 5s ($50 \div 10 = 5$) and on the bottom line counting by 10% ($100 \div 10 =10$). We can then see that 30% of 50 pounds is 15 pounds.

We can also use a table to solve this problem.

A table for weight in pounds: 50, 5, 15, and percentage: 100, 10, 30. Multiply both 50 pounds and 100% by $\frac{1}{10}$ to find 5 pounds is 10%. Multiply 5 pounds and 10% by 3 to find 15 pounds is 30%.

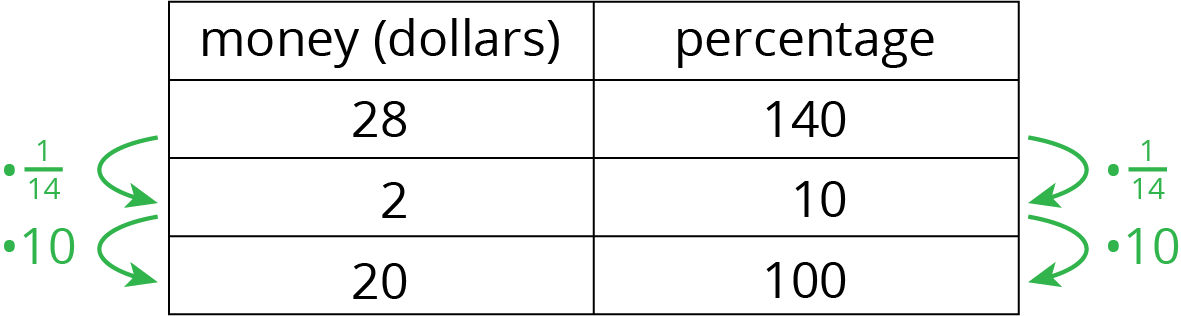
Suppose we know that 140% of an amount is \$28. What is 100% of that amount? Let’s use a double number line to find out.

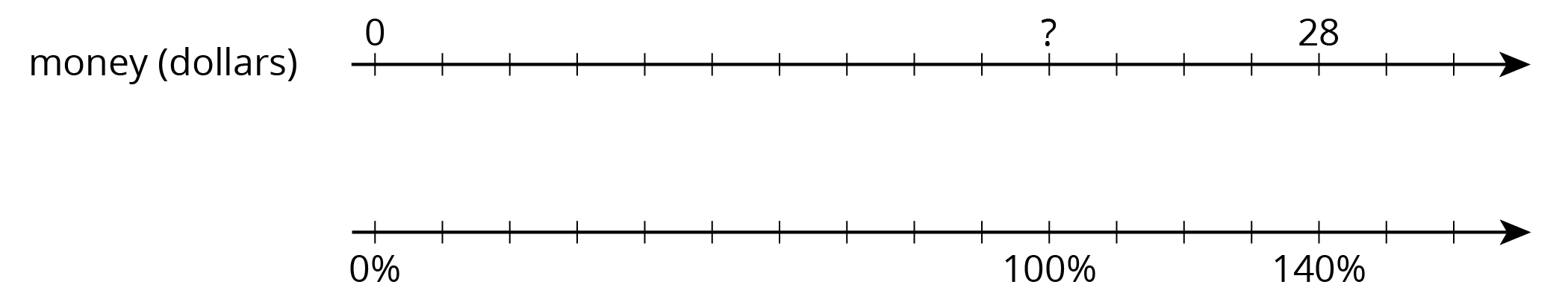
A double number line with 17 evenly spaced tick marks. The top number line is labeled “money, in dollars” and the first tick mark is labeled 0, the eleventh tick mark is labeled with a question mark, and the fifteenth tick mark is labeled 28. The bottom number line is unlabeled and the first tick mark is labeled 0 percent, the eleventh tick mark is labeled 100 percent, and the fifteenth tick mark is labeled 140 percent.

We divide the distance between 0% and 140% and that between \$0 and \$28 into fourteen equal intervals. We label the tick marks on the top line by counting by 2s and on the bottom line counting by 10%. We would then see that 100% is \$20.

Or we can use a table as shown.

A two column table with three rows of data. The first column has the heading “weight, in pounds”. The second column has the heading “percentage.” Row 1; 28, 140. Row 2; 2, 10. Row 3; 20, 100. Arrows on both sides of the table from row 1 to row 2 are labeled "multiply by one fourteenth." Arrows on both sides of the table from row 2 to row 3 are labeled "multiply by 10."

Practice Problems ▶